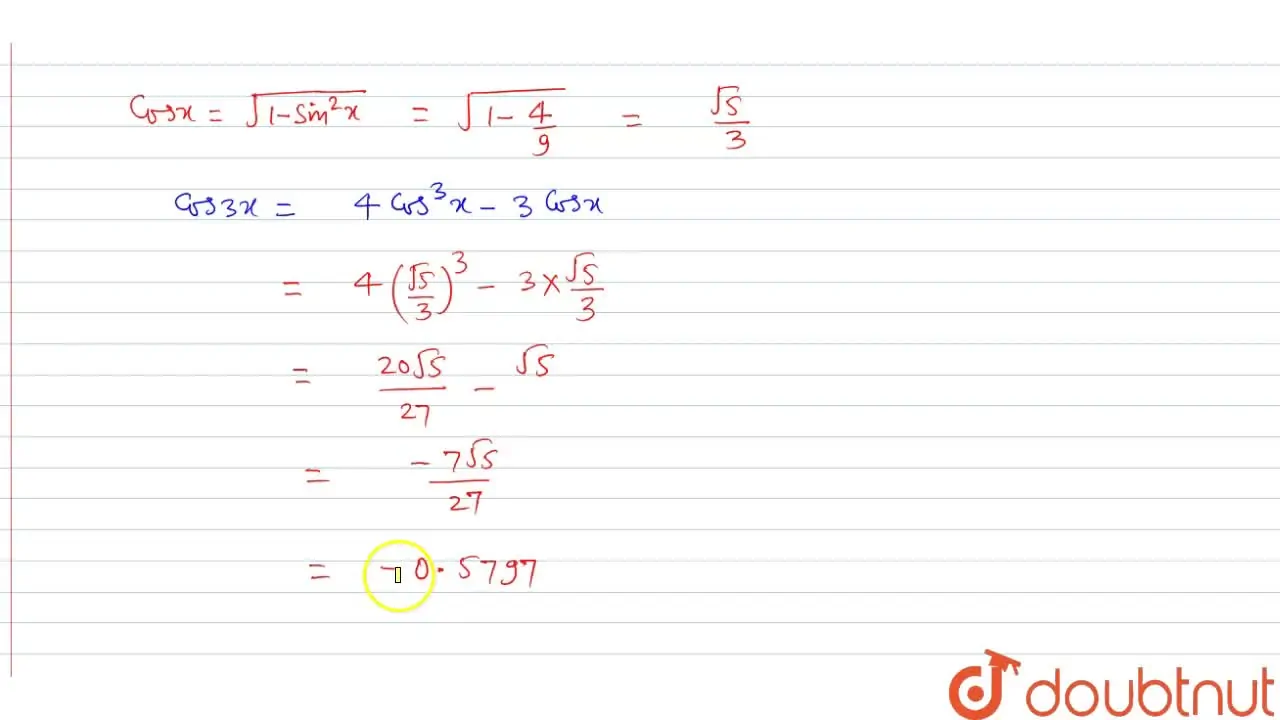
If sinx=23, then find the value of cos3x.
यदि sinx=23 ,तो cos3x का मान ज्ञात कीजिए।
0.6735
−0.8765
−0.5797
0.5678
Step by step video & image solution for If sinx =2/3, then find the value of cos3x. यदि sinx =2/3 ,तो cos3x का मान ज्ञात कीजिए। by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
If sin(x+y) = cos(x-y), then the value of cos2x is:
यदि sin(x+y) = cos(x-y), तो cos2x का मान ज्ञात करें :View SolutionIf D = 4, DoG = 26, then find the value of ANIMAL = ?
View SolutionIf x=2+√3 then the valule of x3−x−3 is:
यदि x=2+√3 है, तो x3−x−3 का मान ज्ञात करें |View SolutionIf x+x−1=2, then the value of x3+x−3 is:
यदि x+x−1=2 है, तो x3+x−3 का मान ज्ञात करें |View SolutionIf x=2+√3 then find the valule of x4−8x3+16x2 is:
यदि x=2+√3 है, तो x4−8x3+16x2 का मान ज्ञात कीजिए ।View SolutionIf sinx = 34 , then cos x = ?
View SolutionIf x=2+√5 then the valule of x3−x−3 is:
यदि x=2+√5 है, तो x3−x−3 का मान ज्ञात करें |View SolutionIf a - b = 4 and a3−b3=88, then find the value of a2−b2.
यदि a - b = 4 और a3−b3=88 है, तो a2−b2 का मान ज्ञात कीजिए।View SolutionIf 2x + y = 6 and xy = 4, then find the value of 8x3+y3 is:
यदि 2x + y = 6 तथा xy = 4 है, तो 8x3+y3 का मान ज्ञात कीजिए।View SolutionIf x=2−√3 then the value of x3−x−3 is:
यदि x=2−√3 है, तो x3−x−3 का मान क्या होगा ?View Solution