Is it possible to have a regular polygon with measure of each exterior angle as 22∘?Solution in Punjabi
Step by step video & image solution for Is it possible to have a regular polygon with measure of each exterior angle as 22^@? by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
What will be the unit digit of the squares of the following numbers:
49
View SolutionFor a given solid match,the views
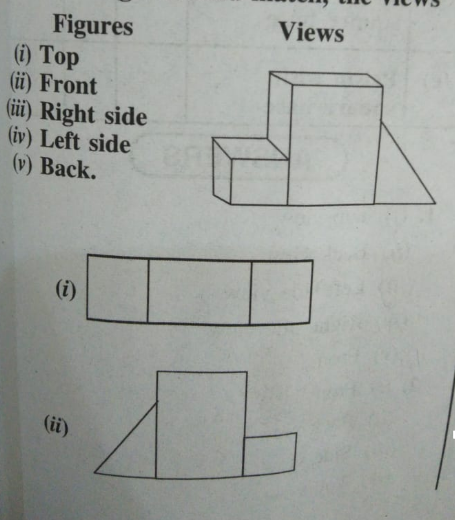 .
.
View Solution(a) Is it possible to have a regular polygon with measure of each exterior angle as 22∘ ?
b) Can it be an interior angle of a regular polygon? Why?
View SolutionIn a regular convex polygon, each interior angle in not more than each exterior angle is not more than each exterior angle. How many such polygons are possible ?
View SolutionIs it possible to have a regular polygon each of whose exterior angles is 25∘ ?
View SolutionIs it possible to have a regular polygon each of whose exterior angles is 50∘ ?
View SolutionFor which of the following measure of an exterior angle a regular polygon is possible?
View Solution(a) Is it possible to have a regular polygon with measure of each exterior angle as 22∘ ? b) Can it be an interior angle of a regular polygon? Why?
View SolutionIs it possible to have a regular polygon with measure of each exterior angle as 22∘?
View SolutionIs it possible to have a regular polygon with measure of each exterior angle as 22∘?
View Solutionएक सम बहुभुज के प्रत्येक बाह्य कोण की माप ज्ञात कीजिए जिसकी
10 भुजाएँ हो
View Solutionउस समबहुभुज की भुजाओं की संख्या, जिसका प्रत्येक बहिष्कोण 45∘ माप का है, होगी
View Solution18 भुजाओं वाले एक सम बहुभुज के प्रत्येक बहिष्कोण की माप ______ है |
View Solutionउस सम बहुभुज की भुजाओं की संख्या, जिसके प्रत्येक बहिष्कोण की माप 60∘ है, ________हैं|
View SolutionThe measure of each exterior angle of a regular polygon of 18 sides is __________.
View Solutiona. Is it possible to have a regular polygon with measure of each exterior angle as 22∘?
b. Can it be an interior angle of a regular polygon? Why?
View Solution
