A 100 mH inductor, a 25μF capacitor and 15Ω resistoer are connected in series to a 120 V, 50Hz a.c source. Calculate the resonant frequency .
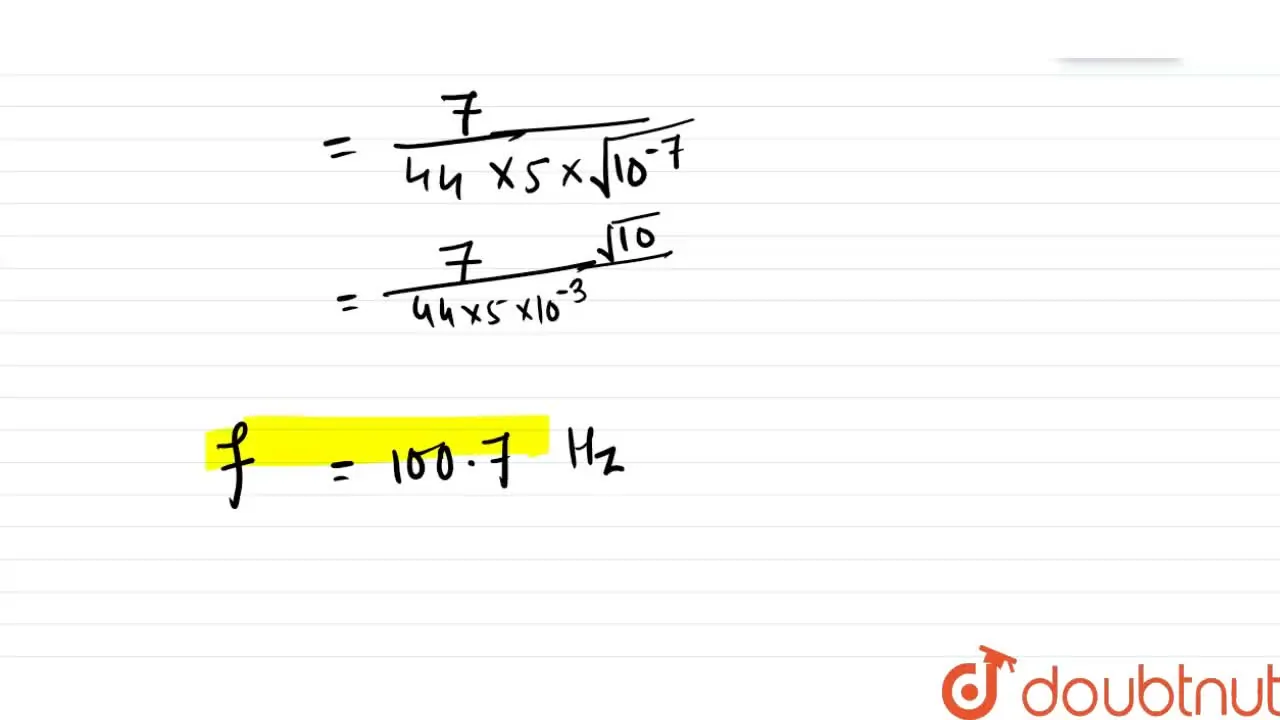
100.7Hz
100Hz
100.2Hz
1000Hz
Her, L=100mH=10−1H
C=25μF=25×10−6F
R=15ΩEV=120Vv=50Hz
At resonance , Z=R=150Ω
IV=EVR=12015=8A
v=12×π√LC=1×72×22×√10−1×25×10−4
7×10344×1.58=100.7Hz
Related Playlists
- BOOK - PHYSICS WALLAHCHAPTER - ALTERNATING CURRENT EXERCISE - LEVEL - 230videos
ALTERNATING CURRENT
- BOOK - PHYSICS WALLAHCHAPTER - ALTERNATING CURRENT EXERCISE - NEET PAST 5 YEARS QUESTIONS8videos
ALTERNATING CURRENT
- BOOK - PHYSICS WALLAHCHAPTER - CURRENT ELECTRICITYEXERCISE - NEET Past 5 Years Questions23videos
CURRENT ELECTRICITY
Similar Questions
A 100 mH inductor, a 20μF capacitor and a 10 ohm resistor are connected in series to a 100V,50Hz a.c. source. Calculate : (i) Inpendance of the circuit at resonance (ii) Current at resonance (iii) Resonant frequency.
View SolutionA 10μF capacitor and a 15Ω resistor are connected in series across a 220 V 50 Hz source . Calculate the value of rms current .
View SolutionA 9/100(π) inductor and a 12Ω resistanace are connected in series to a 225 V, 50 Hz ac source. Calculate the current in the circuit and the phase angle between the current and the source voltage.
View SolutionA 0.21 H inductor and a 12Ω resistance are connected in series to a 220 V, 50 Hz ac source.
The current in the circuit isView SolutionA 31.4Ω resistor and 0.1H inductor are connected in series to a 200V, 50Hz ac source. Calculate
(i) the current in the circuitView SolutionA 40 ohm resistor, 3 mH inductor and 2μF capacitor are connected in series to a 110 V, 5000 Hz a.c. source. Calculate the value of current in the circuit.
View SolutionAn inductor 200 mH, a capacitor C and a resistor 10 ohm are connected in series with a 100 V, 50 s−1 a.c. source. If the current and voltage are in phase with each other, calculate the capacitance of the capacitor.
View SolutionAn inductor 200 mH, capacitor 500 uF, resistor 10 Ω are connected in series with a 100 V variable frequency a.c. source. Calculate :
(i) frequency at which power factor in the circuit is unity,
(ii) current amplitude at this frequency,
(iii) Q-factor.View SolutionAn inductor 200 mH, capactior 500μF, resistor 10 ohm are connected in series with a 100 V, varialbe frequency a.c. source. Calculate (i) frequency at which power factor of the circuit is unity (ii) current amplitude at this frequency (iii) Q factor.
View SolutionA 9100π H inductor and a 12ohm resitance are connected in series to a 225V, 50Hz ac source. Calculate the current in the circuit and the phase angle betweent the current and the source voltage.
View Solution