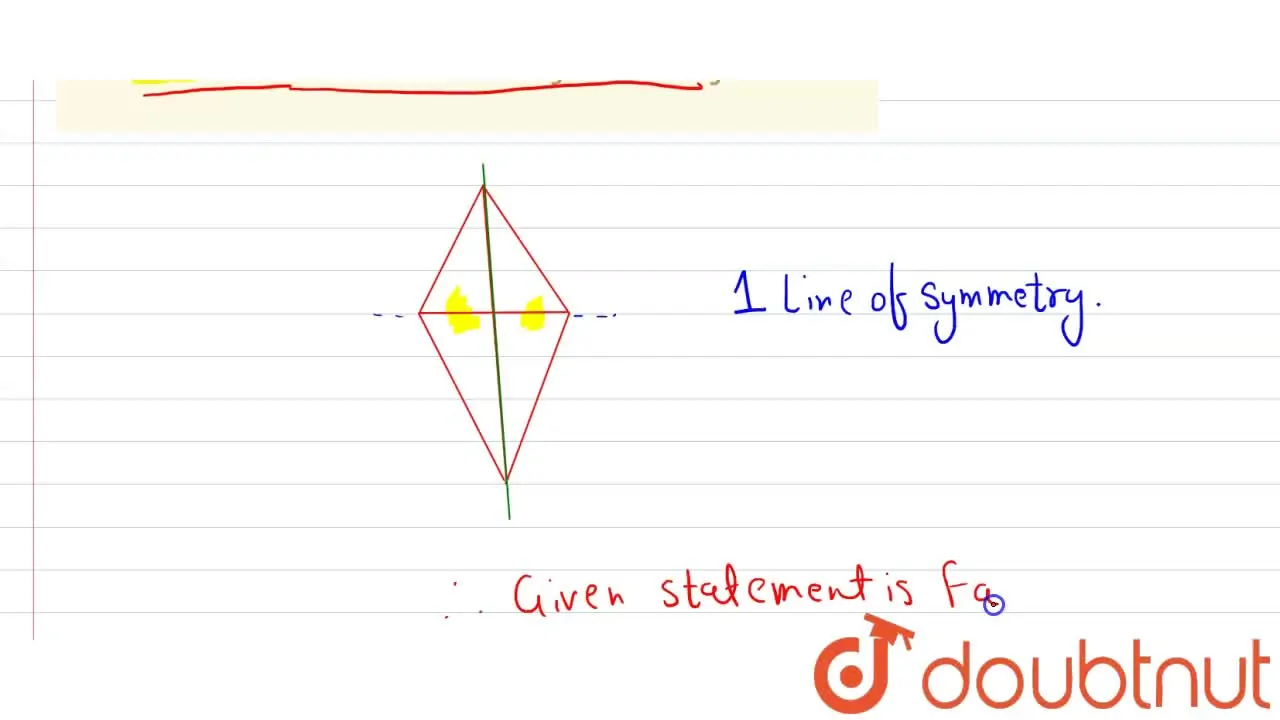
A kite has two lines of symmetry.
Step by step video & image solution for A kite has two lines of symmetry. by Maths experts to help you in doubts & scoring excellent marks in Class 6 exams.
Related Playlists
- BOOK - NCERT EXEMPLARCHAPTER - SYMMETRY AND PRACTICAL GEOMETRY EXERCISE - Exercise29videos
SYMMETRY AND PRACTICAL GEOMETRY
- BOOK - NCERT EXEMPLARCHAPTER - SYMMETRY AND PRACTICAL GEOMETRY EXERCISE - Exercise (In questions 18 to 42, fill in the blanks to make the statements true.)25videos
SYMMETRY AND PRACTICAL GEOMETRY
- BOOK - NCERT EXEMPLARCHAPTER - RATIO AND PROPORTIONEXERCISE - Exercise (In questions 35 to 46, fill in the blanks to make the statements true.)11videos
RATIO AND PROPORTION
Similar Questions
Line of symmetry
View SolutionA kite has one line of symmetry. true or false?
View SolutionA rectangle has two lines of symmetry. Each one of which being the line joining the midpoints of opposite sides. true or false?
View SolutionWhich of the following staements are true and which of them are false?
(vi)A rectangle has two lines of symmetry.View SolutionThe figure which has two lines of symmetry and rotational symmetry of order 2 is
View SolutionWhich of the following statements are true and which are false ?
(i) A parallelogram has no line of symmetry.
(ii) An angle with equal arms has its bisector as the line of symmetry
(iii) An equilaterla triangle has three lines of symmetry.
(iv) A rhombus has four lines of symmetry.
(v) a square has for lines of symmetry.
(vi) A rectangle has two lines of symmetry.
(vii) Each one of the letters H,I,O,X of the English alpabet has two lines of symmetry.View SolutionA rectangle has two lines of symmetry, each one of which is the line joining the midpoints of opposite sides.true or false?
View SolutionEach of the following letters from English alphabet has two lines of symmetry. Identify lines of symmetry in each case: (i) H (ii) I (iii) O
View SolutionWhich of the following has more than two lines of symmetry
View SolutionFigures with more than two lines of symmetry
View Solution