The equation of the tangents drawn from the origin to the circle x2+y2−2rx−2hy+h2=0 are Solution in Tamil
A
x = 0
B
y = 0
C
(h2−r2)x−2rhy=0
D
(h2−r2)x+2rhy=0
Video Solution
Text Solution
Since, tangents are drawn from origin. So, the equation of tangent be y = mx
⇒ Length of perpendicular from origin = radius
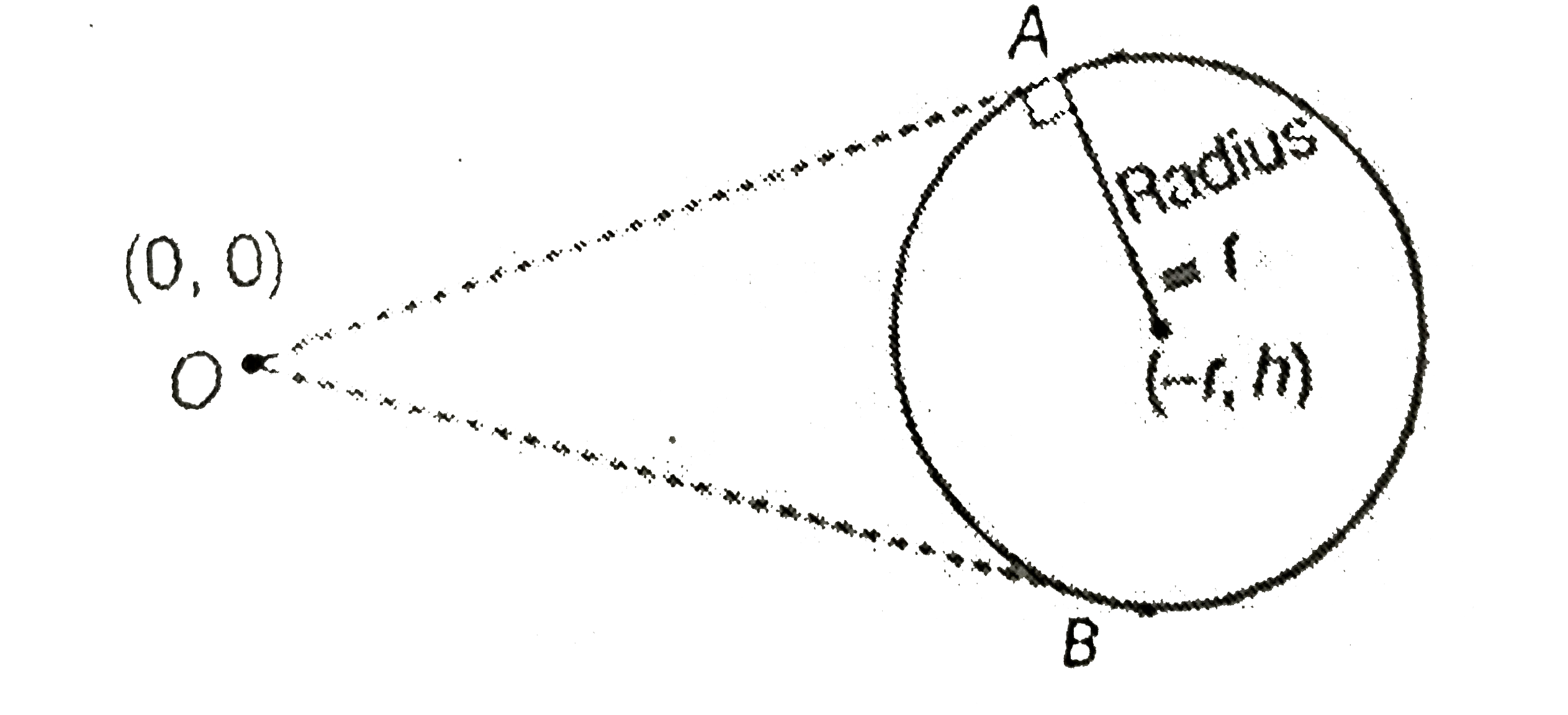
⇒|mr+h|√m2+1=r
⇒m2r2+h2+2mrh=r2(m2+1)
⇒m=∣∣∣r2−h22rh∣∣∣,m=∞
∴ Equation oftangents are y=∣∣∣r2−h22rh∣∣∣x,x=0 Therefore (a) and (c) are the correct answers.
|
Similar Questions
मूल बिन्दु से वृत्त x2+y2−2rx−2hy+h2=0 पर खींची गयी स्पर्श रेखाओं के समीकरण है
View Solutionमूल बिन्दु से वृत्त x2+y2−2rx−2hy+h2=0 पर खींची गई स्पर्श रेखाओं के समीकरण हैं।
View Solutionमूल बिन्दु से वृत्त x2+y2−2rx−2hy+h2=0 पर खींची गयी स्पर्श रेखाओं के समीकरण है
View Solutionमूल बिन्दु से वृत्त x2+y2−2rx−2hy+h2=0 पर खींची गई स्पर्श रेखाओं के समीकरण हैं।
View Solutionवृत्त x2+y2−2rx−2hy+h2=0 पर मूलबिन्दु से खींची गई स्पर्श रेखाओं की समीकरण है

View SolutionThe equations of the tangents drawn from the origin to the circle x2+y2−2rx−2hy+h2=0 are
View SolutionThe tangents drawn from the origin to the circle x^2+y^2-2rx-2hy+h^2=0 are perpendicular if
View SolutionThe equation of the tangents drawn from the origin to the circle x2+y2−2rx+2hy+h2=0 are
View Solution