The locus of the midpoint of a chord of the circle x2+y2=4 which subtends a right angle at the origins is (a)x+y=2 (b) x2+y2=1 (c)x2+y2=2 (d) x+y=1Solution in Tamil
x+y=2
x2+y2=1
x2+y2=2
x+y=1
We have to find loucs of mid-point of chord and we know perpendicular from centre bisects the chord.
∴∠OAC=45∘
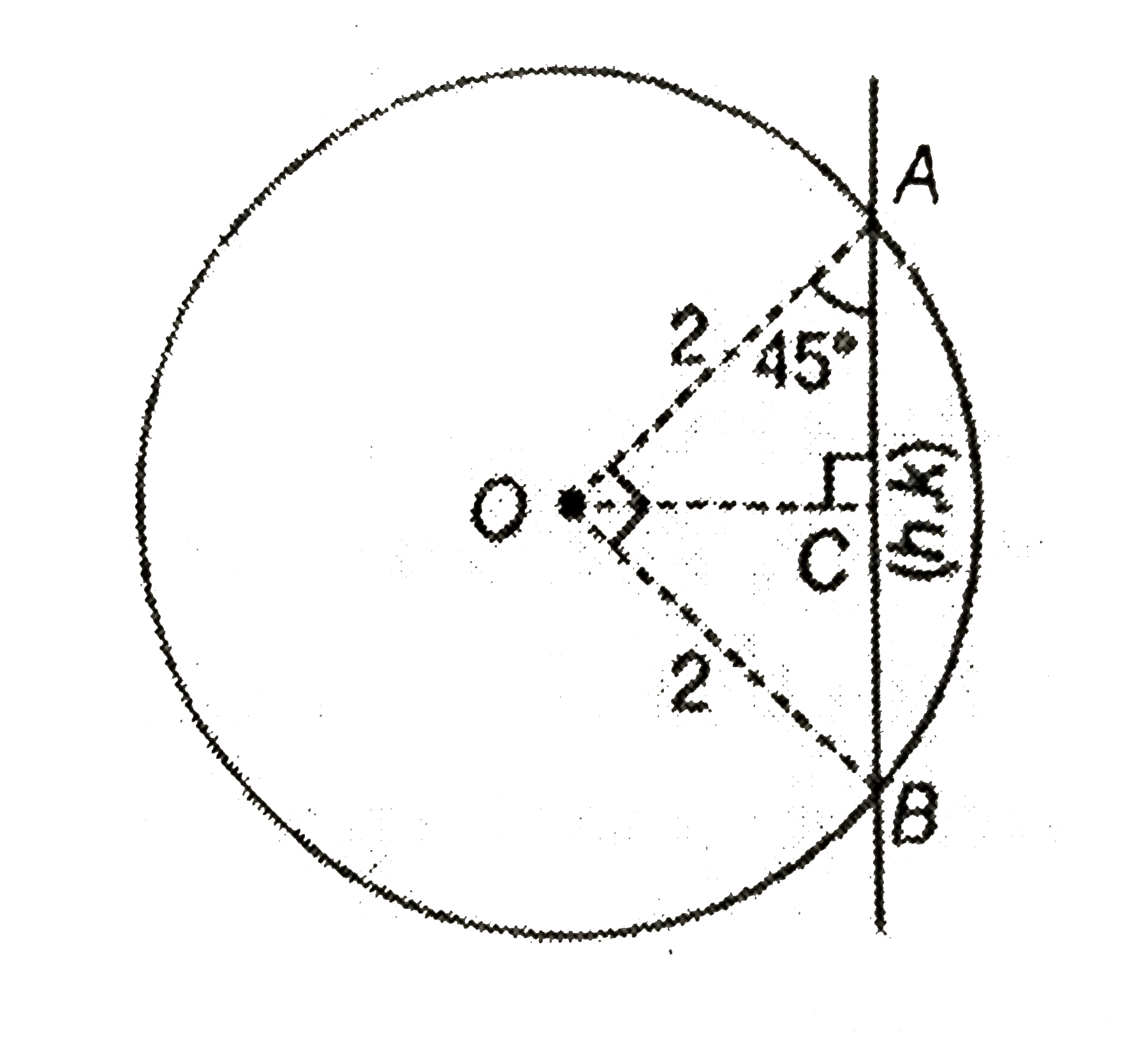
InΔOAC,OCOA=sin45∘
⇒OC=2√2=√2
Also, √h2+k2=OC2
Hence, x2+y2=2 is required equation of locus of mid-point of chord substending right angle at the centre.
Similar Questions
The locus of the midpoints of the chords of the circle x2+y2−ax−by=0 which subtend a right angle at (a2,b2) is (a)ax+by=0 (b) ax+by=a2+b2 (c) x2+y2−ax−by+a2+b28=0 (d) x2+y2−ax−by−a2+b28=0
View SolutionThe locus of the midpoint of a chord of the circle x2+y2=4 which subtends a right angle at the origins is (a) x+y=2 (b) x2+y2=1 (c)x2+y2=2 (d) x+y=1
View SolutionThe locus of the midpoint of the chord of the circle x2+y2=4 which subtends a right angle at the origin is?
View Solutionवृत x2+y2=1 की उस जीवा के मध्य बिंदु का बिन्दुपथ, जो इस प्रकार है की परवलय y=x2−x पर जीवा का खण्ड मूल बिंदु पर समकोण अंतरित करें एक वृत है जिसका केंद्र तथा त्रिज्या क्रमशः है
View SolutionLocus of midpoint of chord of circle x2+y2=1 which subtend right angle at (2,−1) is
View SolutionThe locus of the midpoints of the chords of the circle x2+y2−ax−by=0 which subtend a right angle at (a2,b2) is (a) ax+by=0 (b) ax+by=a2=b2 (c) x2+y2−ax−by+a2+b28=0 (d) x2+y2−ax−by−a2+b28=0
View SolutionThe locus of the midpoint of a chord of the circle x2+y2=4 which subtends a right angle at the origins is (a)x+y=2 (b) x2+y2=1 (c)x2+y2=2 (d) x+y=1
View SolutionThe locus of the midpoint of a chord of the circle x2+y2=4 which subtends a right angle at the origins is (a) x+y=2 (b) x2+y2=1 x2+y2=2 (d) x+y=1
View Solution
