Let C be the circle with centre at (1,1) and radius =1 . If T is the circle centered at (0,y) passing through the origin and touching the circle C externally. Then the radius of T is equal to Solution in Tamil
√3√2
√32
12
14
The correct Answer is:D
Plan Use the property, when two circles touch each other externally, then distance between the centre I s equal to sum of their radii, to get required radius.
Let the coordinate of the centre of T be (0, k).
Distance between their centre
k+1=√1+(k−1)2 [∴C1C2=k+1]
⇒K+1=√1+k2+1−2k
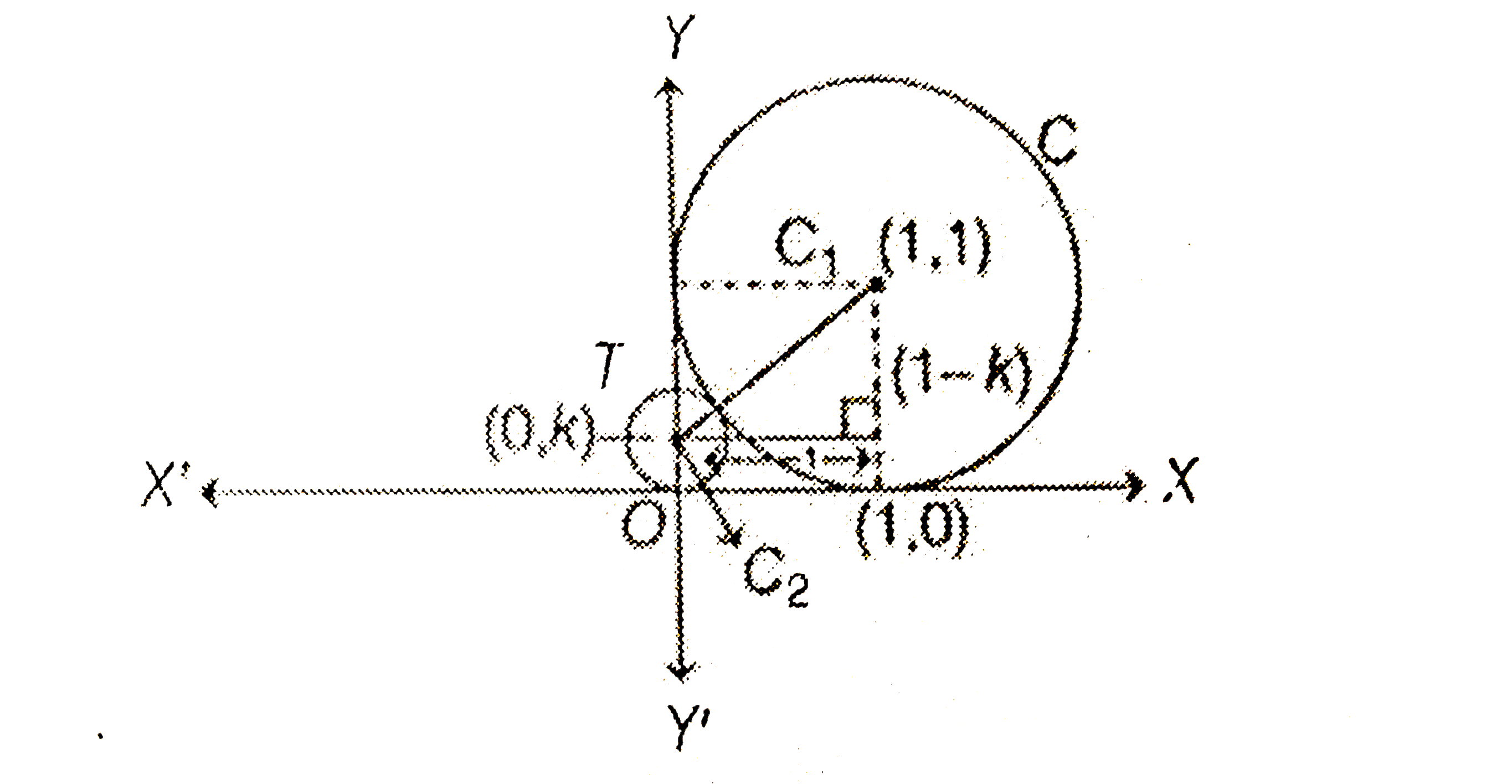
⇒k+1=√k2+2−2k
⇒k2+1+2k=k2+2−2k
⇒k=14
So, the radius of circle T is K, i. e.14.
Similar Questions
माना C एक वृत्त है जिसका केन्द्र (1,1) पर है तथा त्रिज्या 1 है। यदि T केन्द्र (0,y) वाला वृत्त है जी मूलबिन्दु से होकर जाता है तथा वृत्त C को बाह्म रूप से स्पर्श करता है, तो T की त्रिज्या बराबर है
View SolutionLet C be the circle with centre at (1, 1) and radius = 1. If T is the circle centred at (0, y), passing through origin and touching the circle C externally, then the radius of T is equal to :
View SolutionC என்ற வட்டத்தின் மையம் (1, 1) மற்றும் ஆரம் 1 அலகு என்க. T என்ற வட்டத்தின் மையம் (0,y) ஆகவும் ஆதிப்புள்ளி வழியாகவும் உள்ளது. மேலும் C என்ற வட்டத்தை வெளிப்புறமாகத் தொட்டுச் செல்கிறது எனில் வட்டம் -ன் ஆரம்
View SolutionLet "c" be the circle with centre at (1,1) and radius =1. If 'T' is the circle centred at (0,y) passing thorugh origin and touching the circle 'C' externally , then the radius of 'T' is equal to:
View SolutionLet C be the circle with centre at (1, 1) and radius = 1. If T is the circle centred at (0, y), passing through origin and touching the circle C externally, then the radius of T is equal to (1) √3√2 (2) √32 (3) 12 (3) 14
View SolutionLet C be the circle with centre at (1, 1) and radius = 1. If T is the circle centred at (0, y), passing through origin and touching the circle C externally, then the radius of T is equal to (1) √3√2 (2) √32 (3) 12 (3) 14
View SolutionLet C be the circle with centre at (1, 1) and radius = 1. If T is the circle centered at (0, Y) passing through origin and touching the circle C externally, then the radius of T is equal to :
View SolutionLet C be the circle with centre at (1, 1) and radius = 1. If T is the circle centred at (0, y), passing through origin and touching the circle C externally, then the radius of T is equal to
View Solution
