A hemi-spherical tank of radius 2 m is initially full of water and has an outlet of $12 c m^{2}$ cross-sectional area at the bottom. The outlet is opened at some instant. The flow through the outlet is according to the law $v (t) = \sqrt{0.62 g h (t)},$ where $v (t)$ and $h (t)$ are, respectively, the velocity of the flow through the outlet and the height of water level above the outlet and the height of water level above the outlet at time $t,$ and $g$ is the acceleration due to gravity. Find the time it takes to empty the tank.Solution in Tamil

Video Solution

Text Solution

Verified by Experts

The correct Answer is:((14pixx10^(5))/(27sqrt(g))unit)

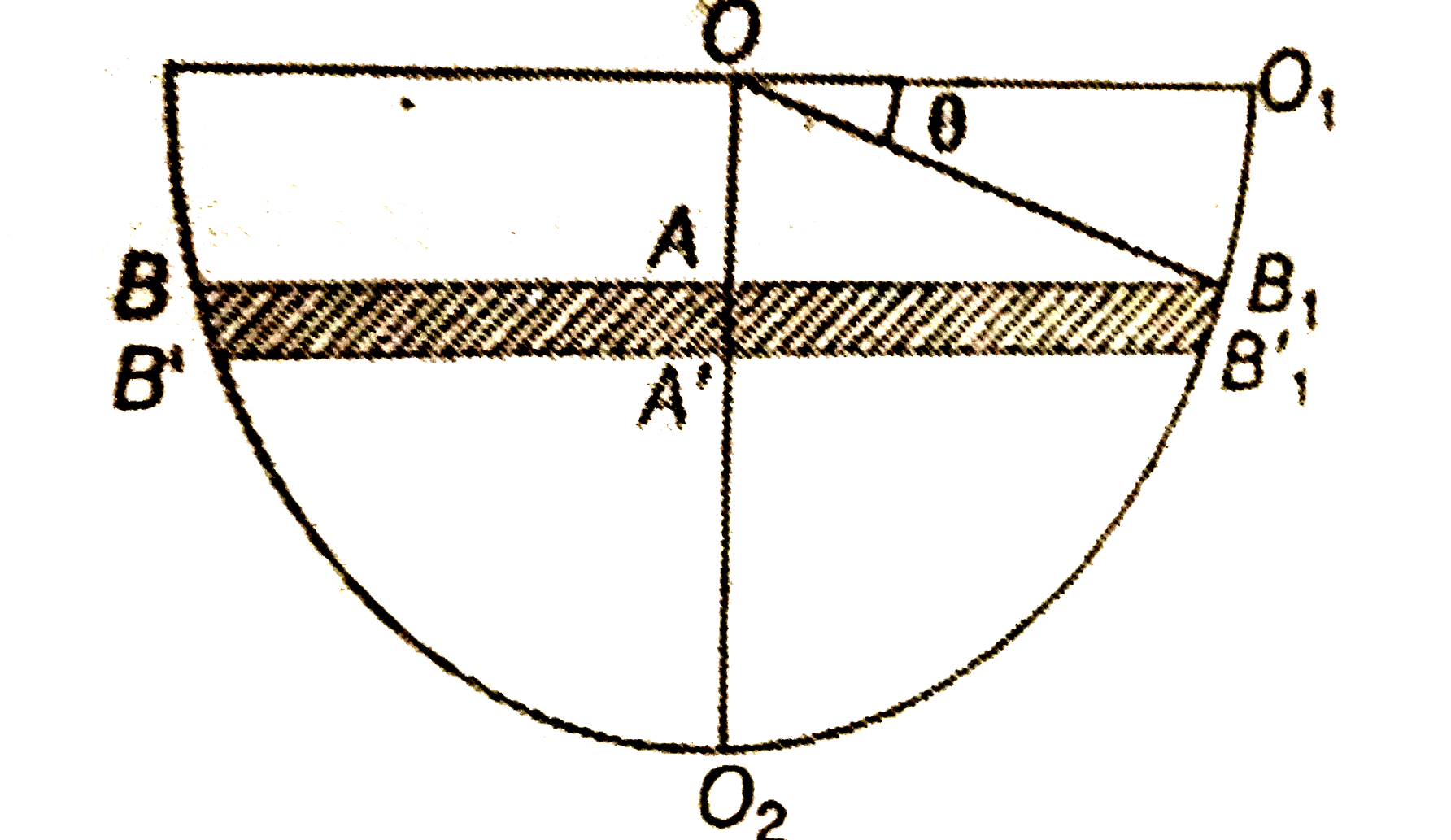
Let O be the centre of hemispherical tank. Let at any
instant t, water level be $B A B_{1}$ and t+dt, water level
is $B' A' B_{1} .$ Let $< O_{1} O B_{1} = θ .$
$\Rightarrow A B_{1} = r cos θ and O A = r sin θ$ decrease in the water
volume in time $d i = π A B_{1}^{2} . d (O A)$
[ $π r^{2}$ is surface area of water level and d (OA) is depth
of weter level]
$= π r^{2} \cdot {cos}^{2} θ \cdot r cos θ d θ$
$= π r^{3} \cdot {cos}^{3} θ d θ$
Also, $h (t) = O_{2} A = r - r sin θ = r (1 - sin θ)$
Now, outflow rate $Q = A \cdot v (t) = A \cdot 0.6 \sqrt{2 g r (- sin θ)}$ ltbr> Where, A is the area of the outlet.
Thus, volume fliwing out in time dt.
$\Rightarrow Q_{d t} = A \cdot (0.6) \cdot \sqrt{2 g r} \cdot \sqrt{1 - sin θ} d t$
We have, $π r^{3} {cos}^{3} θ d θ = A (0.6) \cdot \sqrt{2 g r} \cdot \sqrt{1 - sin θ} d t$
$\Rightarrow \frac{π r^{3}}{A (0.6) \sqrt{2 g r}} \cdot \frac{{cos}^{3} θ}{\sqrt{1 - sin θ}} d θ = d t$
Let the time taken to empty the tank be T.
Then, $T = \int_{0}^{π / 2} \frac{π r^{3}}{A (0.6) \sqrt{2 g r}} \cdot \frac{{cos}^{3} θ}{\sqrt{1 - sin θ}} d θ$
$= \frac{π r^{3}}{A (0.6) \sqrt{2 g r}} \int_{0}^{π / 2} \frac{1 - {sin}^{2} θ (- cos θ)}{\sqrt{1 - sin θ}} d θ$
Let $t_{1} = \sqrt{1 - sin θ}$
$\Rightarrow {d t}_{1} = \frac{- cos θ}{\sqrt{1 - sin θ}} d θ$
$∴ T = \frac{- 2 π r^{3}}{A (0.6) \sqrt{2 g r}} \int_{1}^{0} [1 - {(1 - t_{1}^{2})}^{2}] {d t}_{1}$
$\Rightarrow T = \frac{- 2 π r^{3}}{A (0.6) \sqrt{2 g r}} \int_{1}^{0} [1 - (1 + t_{1}^{4} - 2 t_{1}^{2})] {d t}_{1}$
$\Rightarrow T = \frac{- 2 π r^{3}}{A (0.6) \sqrt{2 g r}} \int_{1}^{0} [1 - (1 - t_{1}^{4} + 2 t_{1}^{2}] {d t}_{1}$
$\Rightarrow T = \frac{2 π r^{3}}{A (0.6) \sqrt{2 g r}} \int_{1}^{0} (t_{1}^{4} + 2 t_{1}^{2}) {d t}_{1}$
$\Rightarrow T = \frac{2 π r^{3}}{A (0.6) \sqrt{2 g r}} \int_{1}^{0} {[\frac{t_{1}^{5}}{5} - \frac{2 t_{1}^{3}}{3}]}_{1}^{0}$
$\Rightarrow T = \frac{2 π r \cdot r^{5 / 2}}{A (\frac{6}{10}) \sqrt{2 g r}} \cdot [0 - \frac{1}{5} - 0 + \frac{2}{3}]$
$\Rightarrow T = \frac{2 π \cdot 2^{5 / 2} {(10^{2})}^{5 / 2}}{12 \cdot \frac{3}{5} \cdot \sqrt{2} \cdot \sqrt{g}} [\frac{2}{3} - \frac{1}{5}]$
$= \frac{2 π \times 10^{5} \cdot 4 \cdot 5}{(12 \times 3) \sqrt{g}} [\frac{10 - 3}{15}]$
$= \frac{2 π \times 10^{5} \times 7}{3 \cdot 3 \cdot \sqrt{g} \cdot 3} = \frac{14 π \times 10^{5}}{27 \sqrt{g}} u n i t$

Updated on:21/07/2023

|

Class 12 MATHS DIFFERENTIAL EQUATIONS

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile