Let √3ˆi+ˆj,ˆi+√3ˆj and βˆi+(1−β)ˆj respectively be the position vectors of the points A, B and C with respect to the origin O. If distance of C from the bisector of the acute angle between OA and OB is 3√2, then the sum of all possible values of β is (a) 1 (b) 3 (c) 4 (c) 2 Solution in Tamil
1
3
4
2
The correct Answer is:A
According to given information, we have the following figure.
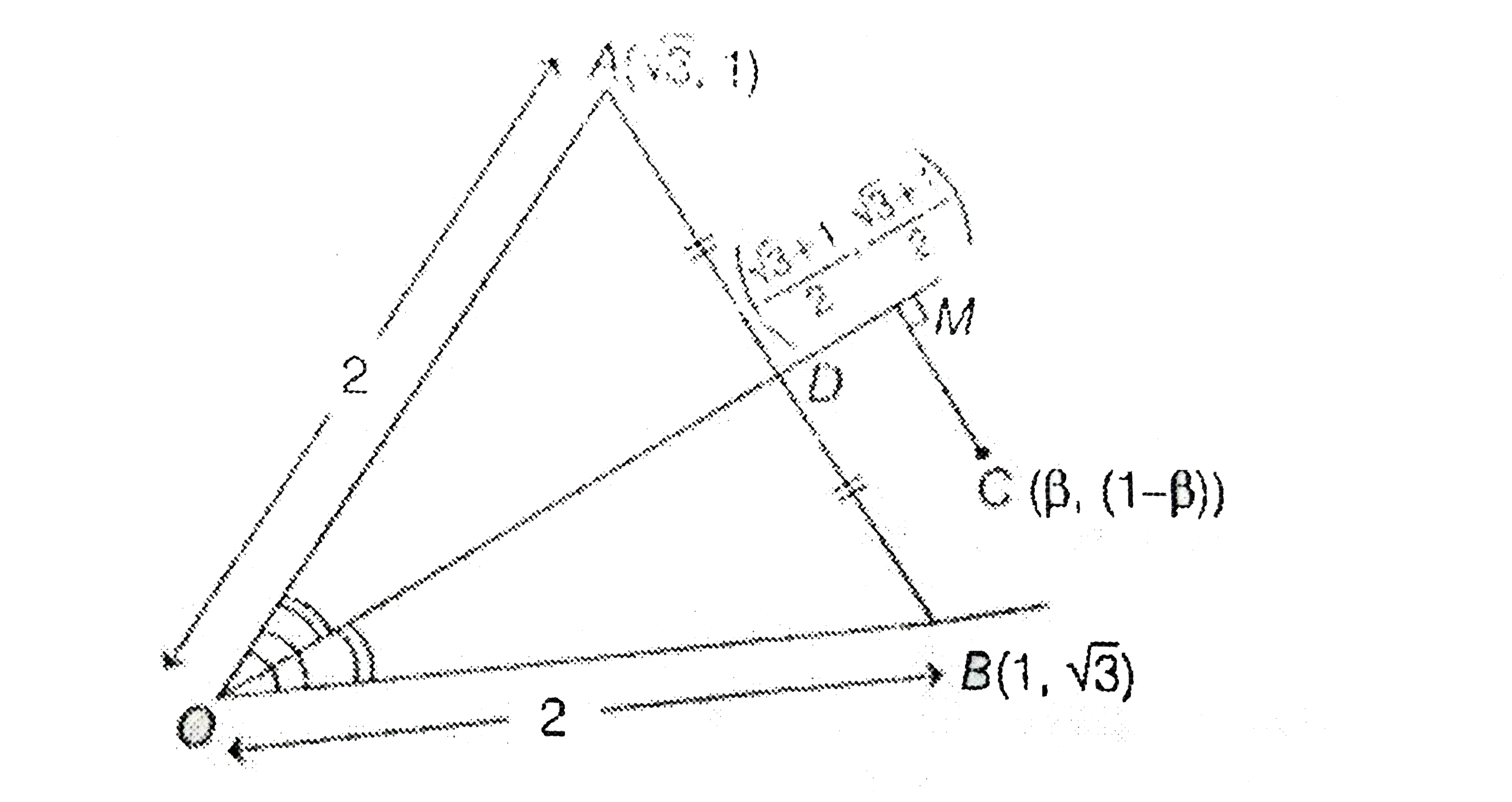
Clearly, angle bisector divides the sides AB in OA:OB,
i.e.,2:2=1:1 [using∠bisec→rtheorem]
So, D is the mid-point of AB and hence coordinates of D are (√3+12,√3+12)
Now, eauation of bisector OD is
(y−0)=⎛⎜⎝√3+12−0√3+12−0⎞⎟⎠(x−0)⇒y=x
⇒x−y=0
According to the problem,
3√2=CM=∣∣∣β−(1−β)√2∣∣∣
[Distance of a point P(x1,y1) from the line ax+by+c=0 is ∣∣∣ax1+by1+c√a2+b2∣∣∣]
⇒ |2β−1|=3⇒2β=±3+1
⇒ 2β=4,−2⇒β=2,−1
Sum of 2 and -1 is 1.
Similar Questions
Position vector of ponts A,B,C are →a,→b,→c respectively which are by →a=√3ˆi+ˆj,→b=ˆi+√3ˆj,→c=βˆi+(1−β)ˆj. If distance of point C from angle bisector of OA and OB is 3√2 then value of β is (A) 1 (B) 2 (C) 4 (D) 3
View SolutionThe position vectors of the points A, B, C are 2ˆi+ˆj−ˆk,3ˆi−2ˆj+ˆkandˆi+4ˆj−3ˆk respectively . These points
View SolutionLet √3ˆi+ˆj,ˆi+√3ˆj and βˆi+(1−β)ˆj respectively be the position vedors of the points A, B and C with respect the origin O. If the distance of C from the bisector of the acute angle between OA and OB is 3√2, then the sum all possible values of β is _______.
View SolutionIf vector →a=ˆi+ˆj+ˆk,→b=4ˆi+3ˆj+4ˆk and →c=ˆi+αˆj+βˆk are linearly dependent and |→c|=√3 , then value of |α|+|β| is
View SolutionThe position vectors of the points A, B, C are 2ˆi+ˆj−ˆk,3ˆi−2ˆj+ˆkandˆi+4ˆj−3ˆk respectively . These points
View SolutionThe position vectors of the points A, B, C are 2ˆi+ˆj−ˆk,3ˆi−2ˆj+ˆkandˆi+4ˆj−3ˆk respectively . These points
View SolutionA unit vector in xy plane makes an angle of 45∘ with the vector ˆi+ˆj and an angle of 60∘ with the vector 3ˆi−4ˆj is a)ˆi b)ˆi+ˆj√2 c)2ˆi+ˆj√2 d)None of these
View SolutionLet →a=ˆi−ˆj,→b=ˆj−ˆk,→c=ˆk−ˆi.Ifˆd is a unit vector such that →a.ˆd=0=[→b,→c,→d] then hatdequals(A)+-(hati+hatj-2hatk)/sqrt(6)(B)+-(hati+hatj-hatk)/sqrt(3)(C)+-(hati+hatj+hatk)/sqrt(3)(D)+-hatk
View Solution