Prove, by vector method or otherwise, that the point of intersection of the diagonals of a trapezium lies on the line passing through the midpoint of the parallel sides (you may assume that the trapezium is not a parallelogram.) Solution in Tamil
Let O be the origin of reference. Let the position vectors of A and B be →a and →b respectively.
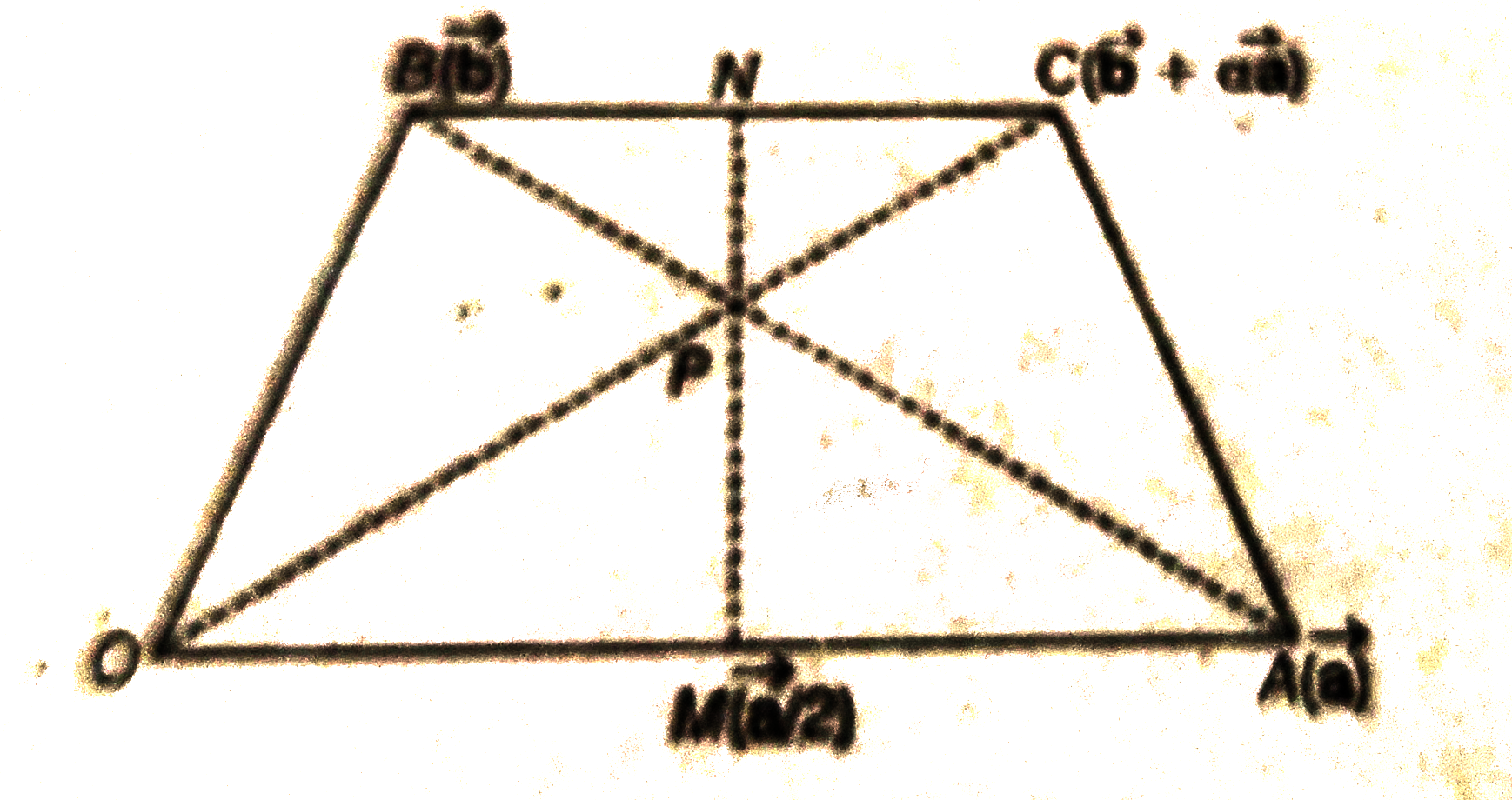
Since BC∣∣OA,−−→BA=α−−→OA=a→a for some constant α.
Equation of OC is →r=t(→b+α→a) and
equation of AB is →r=→a+λ(→b−→a)
Let P be the point of intersection of OCandAB. Then at point P,t(→b+α→a)=→a+λ(→b−→a) for some values of t and λ
⇒(tα−1+λ)→a=(λ−t)→b
Since →a and →b are non-parallel vectors we must have
tα=1+λ=0 and λ=t⇒t=1/(α+1)
Thus position vectors of is →r1=1,α+1(→b+α→a)
Equation of MN is →r=12→a+k[→b+12(a−1)→a]
For k=1/(α+1){which is the coefficient of →b in →r1} we get
→r=12→a+1α+1[→b+12(α−1)→a]
=1α+1→b+1α+1(α−1+α+1)→a
=1α+1(→b+α→a)=→r1⇒P lies on MN
Similar Questions
In a quadrilateral ABCD , if the midpoints of one pair of opposite sides and the point of intersection of the diagonals are collinear , using vector methods , prove that the quadrilateral ABCD is a trapezium .
View SolutionProve that the line segment joining the midpoints of the diagonals of a trapezium is parallel to the parallelogram of the trapezium and half of their difference.
View SolutionIn a trapezium, prove that the line joining the midpoints of non - parallel sides are parallel to the parallel sides of the trapezium.
View SolutionProve that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of trapezium and is half of their difference.
View SolutionProve that the line segment obtained by joining the midpoints of the diagonals of a trapezium is parallel to the parallel sides of the trapezium.
View SolutionABCD is trapezium in which AB and CD are parallel. Prove by vector methods that the mind points of the sides AB, CD and the intersection of the diagonals are collinear.
View SolutionProve that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of that trapezium.
View SolutionABCD is trapezium in which AB and CD are parallel. Prove by vector methods that the mind points of the sides AB, CD and the intersection of the diagonals are collinear.
View Solution
