In a high school, a committee has to be formed from a group of 6 boys M1,M2,M3,M4,M5,M6 and 5 girls G1,G2,G3,G4,G5.
(i) Let a1 be the total number of ways in which the committee can be formed such that the committee has 5 members, having exactly 3 boys and 2 girls
(ii) Let a2 be the total number of ways in which the committee can be formed such that the committee has at least 2 members, and having an equal number of boys and girls.
(iii) Let a3 be the total number of ways in which the committee can be formed such that the committee has 5 members, at least 2 of them being girls.
(iv) Let a4 be the total number of ways in which the committee can be formed such that the committee has 4 members, having at least 2 girls such that both M1 and G1 are NOT in the committee together.
The correct option isSolution in Tamil
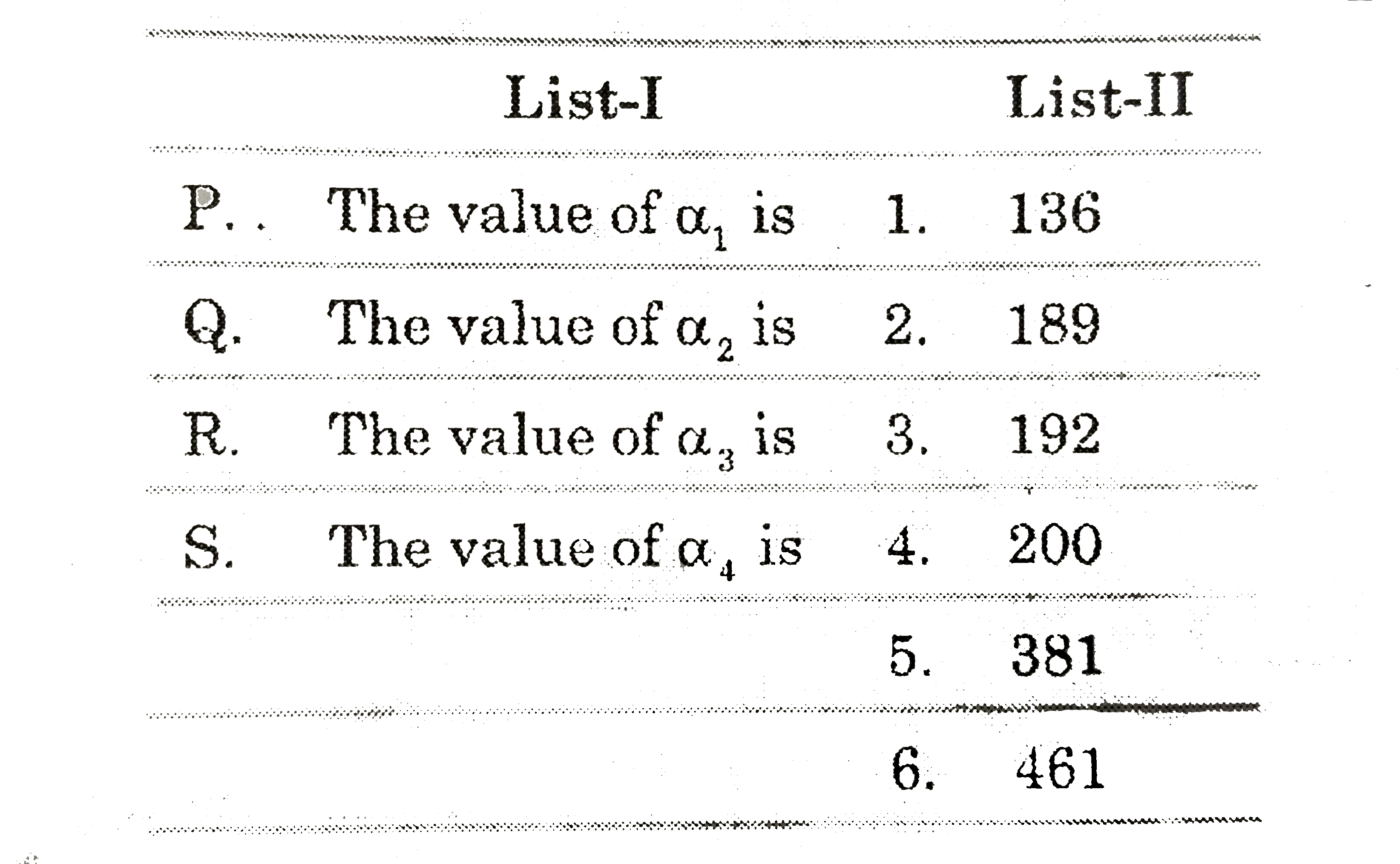
P→4,Q→6,R→2,S→1
P→1,Q→4,R→2,S→3
P→4,Q→6,R→5,S→2
P→4,Q→2,R→3,S→1
The correct Answer is:C
Given 6 boys M1,M2,M3,M4,M5,M6 and 5 girls G1,G2,G3,G4,G5
(i) α1→ Total number of ways of selecting 3 boys and 2 girls from 6 boys and 5 girls.
i.e., 6C3×5C2=20×10=200
∴ α1=200
(ii) α2→ Total number of ways selecting at least 2 member and having equal number of boys and girls
i.e., 6C15C1+6C25C2+6C35C3+6C45C4+6C55C5
= 30 + 150 + 200 + 75 + 6 = 461
⇒ α2=461
(iii) α3→ Total number of ways of selecting 5 members in which at least 2 of them girls
i.e., 5C26C3+5C36C2+5C46C1+5C56C0
= 200 + 150 + 30 + 1 = 381
α3=381
(iv) α4→ Total number of ways of selecting 4 members in which at least two girls such that M1 and G1 are not included together.
G1 is included →4C1⋅5C2+4C2⋅5C1+4C3
= 40 + 30 + 4 = 74
M1 is included →4C2⋅5⋅C1+4C3=30+4=34
G1 and M1 both are not included
4C4+4C3⋅5C1+4C2⋅5C2
1 + 20 + 60 = 81
∴ Total number = 74 + 34 + 81 = 189
α4=189
Now P→4,Q→6,R→5,S→2
Hence, option (c) is correct.
Similar Questions
In a high school, a commintee has to be formed from a group of 6 boys M1,M2,M3,M4,M5,M6 and 5 girls G1,G2,G3,G4,G5.
(i) Let α1 be the total number of ways in which of the commitee can be formed such that the commitee has 5 members, having exactly 3 boys and 2 girls.
(ii) Let α2 be the total number of ways in which the commitee can be formed such that the committee has at least 2 members, and having an equal number of bosy and girls.
(iii) Let α3 be the total number of ways in wihc the committee can be formed such that the commitee has 5 members, at least 2 of them being girls.
(iv) Let α4 be the total number of ways in which the committee can be formed such that the committee has 4 members, having at least 2 girls and such that both M1 and G1 are NOT in the committee together.
List - I List - II
P. The value of α1 is 1. 136
Q. The value of α2 is 2. 189
R. The value ofα3 is 3. 192
S. The value of α4 is 4. 200
5. 381
6. 461
View SolutionIf A1 ,be the A.M.and G1,G2 be two G.M.'s between two positive numbers then G31+G32G1G2A1 is equal to:
View Solutionएक हाई स्कूल (high school) में, 6 बालकों M1,M2,M3,M4,M5,M6 और 5 बालिकाओं G1,G2,G3,G4,G5, के समूह (group) में से एक समिति (committee) बनाई जानी है। (i) माना कि α1 समिति को इस प्रकार से बनाने के तरीकों (ways) की कुल संख्या है कि समिति में 5 सदस्य हैं, जिनमें से ठीक (exactly) 3 बालक और 2 बालिकाएँ हैं। (ii) माना कि α2 समिति को इस प्रकार से बनाने के तरीकों की कुल संख्या है कि समिति में कम से कम (atleast) 2 सदस्य हैं, और बालकों और बालिकाओं की संख्या बराबर (equal) है। (iii) माना कि α3 समिति को इस प्रकार से बनाने के तरीकों की कुल संख्या है कि समिति में 5 सदस्य हैं, जिनमें से कम से कम 2 बालिकाएँ हैं। (iv) माना कि α4 समिति को इस प्रकार से बनाने के तरीकों की कुल संख्या है कि समिति में 4 सदस्य हैं, जिनमें से कम से कम 2 बालिकाएँ हैं और M1 व G1 समिति में एक साथ नहीं हैं।

दिए हुए विकल्पों में से सही विकल्प है:
View Solutionएक हाई स्कूल में 6 बालकों M1,M2,M3,M4,M5,M6 और 5 बालिकाओं G1,G2,G3,G4,G5 के समूह में से एक समिति बनाई जाती है | इस प्रकार बनाने के तरीकों की कुल संख्या है कितनी है कि समिति में 5 सदस्य है जिनमें से ठीक 3 बालक और 2 बालिकाएं हैं
View SolutionSeven girls G1,G2,G3, …, G7 are such that their ages are in order G1<G2<G3<…<G7. Five girls are selected at random and arranged in increasing order of their ages. The probability that G5 and G7 are not consecutive is
View SolutionIn a high school, 6 boys M_1 ,M_2 ,M_3,M_4,M_5, M_6 And 5 girls G_1 ,G_2, G_3 ,G_4 ,G_5, A committee is to be formed from the group of (i) Admittedly alpha_1 The total number of ways to constitute the committee in such a way is that the committee has 5 members, out of which exactly 3 are boys and 2 are girls. (ii) Admittedly alpha_2 The total number of ways to form a committee is such that the committee has atleast 2 members, and the number of boys and girls is equal. (iii) Admittedly alpha_3 The total number of ways to form the committee is such that the committee has 5 members, out of which at least 2 are girls. (iv) Admittedly alpha_4 The total number of ways to constitute the committee in such a way is that the committee has 4 members, out of which at least 2 are girls and M_1 and G_1 The committee is not together. The correct option is from the given options:
View Solutionइकाई त्रिज्या के वृत्त के अन्तर्गत एक समसप्तभुज बनाया गया है जिसके शीर्ष A1A2,.....A7 है | ΔA1,A2,A5 का केन्द्रक G1 है। और ΔA2A5A7 का केन्द्रक G2 है तथा ΔOG1,G2 का केन्द्रक P है | जहाँ O परिवृत्त का केन्द्र है।
G3 इस प्रकार है कि त्रिभुज G1G2G3 का केन्द्रक O है, तो
View SolutionSeven girls G1,G2,G3, …, G7 are such that their ages are in order G1<G2<G3<…<G7. Five girls are selected at random and arranged in increasing order of their ages. The probability that G5 and G7 are not consecutive is
View Solution