If y=sec(tan−1x), then dydx at x=1 is equal toSolution in Tamil
A
1√2
B
12
C
1
D
√2
Video Solution
Text Solution
The correct Answer is:A
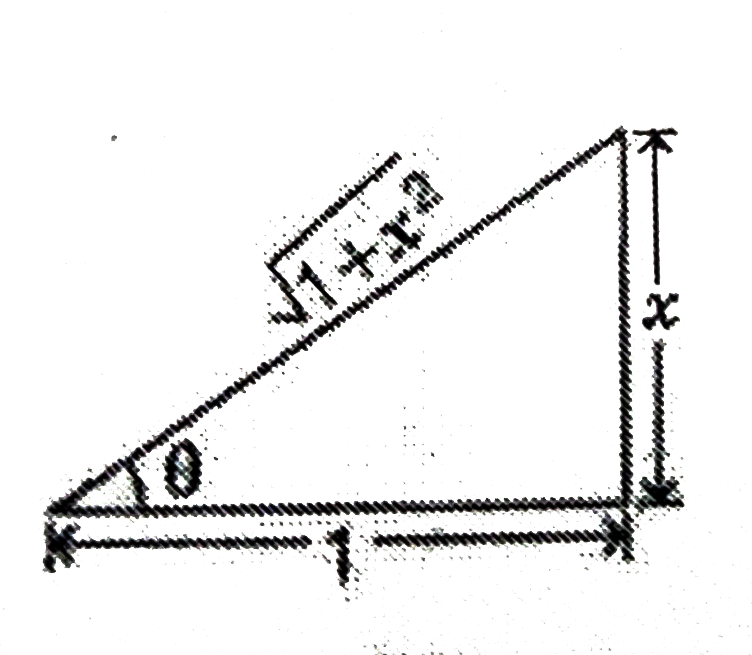
Given,y=sec(tan1x)
Let tan−1x=θ
⇒ x=tanθ
∴ y=tanθ=√1+x2
On differentiating w.r.t. X, we get
dydx=12√1+x2⋅2x
At x=1, dydx=1√2
|
Similar Questions
If y=tan−1x+sec−1x+cot−1x+cosec−1x,
then dydx is equal to
View SolutionIf y=sec(tan−1x), then dydx is equal to
View SolutionIf y=sec(tan−1x), then dydx∣∣∣x=1 is equal to
View SolutionIf y=tan−1(secx−tanx),thendydx is equal to
View SolutionIf y=sec(tan−1x) then dydx at x=1 is equal to
View SolutionIf y=sec(tan−1x), then dydx at x =1 is equal to
View SolutionIf y=sec(tan−1x) , then dydx at x = 1 is equal to a)1/2 b)1 c)√2 d)1/√2
View SolutionIf y=sec(tan−1x) , then dydx at x = 1 is equal to a)1/2 b)1 c)√2 d)1/√2
View Solution
