Draw a graph of the function y=[x]+|1−x|,−1≤x≤3. Determine the points if any, where this function is not differentiable. Solution in Tamil
The correct Answer is:{0,1,2)
y=[x]+|1−x|,−1≤x≤3
⇒ y=⎧⎪
⎪
⎪
⎪⎨⎪
⎪
⎪
⎪⎩−1+1−x, −1≤x<00+1−x, 0≤x<11+x−1, 1≤x<22+x−1, 2≤x≤3
⇒ y=⎧⎪
⎪
⎪
⎪⎨⎪
⎪
⎪
⎪⎩−x, −1≤x<01−x, 0≤x<1+x, 1≤x<2x+1, 2≤x<3
which could be shown as,
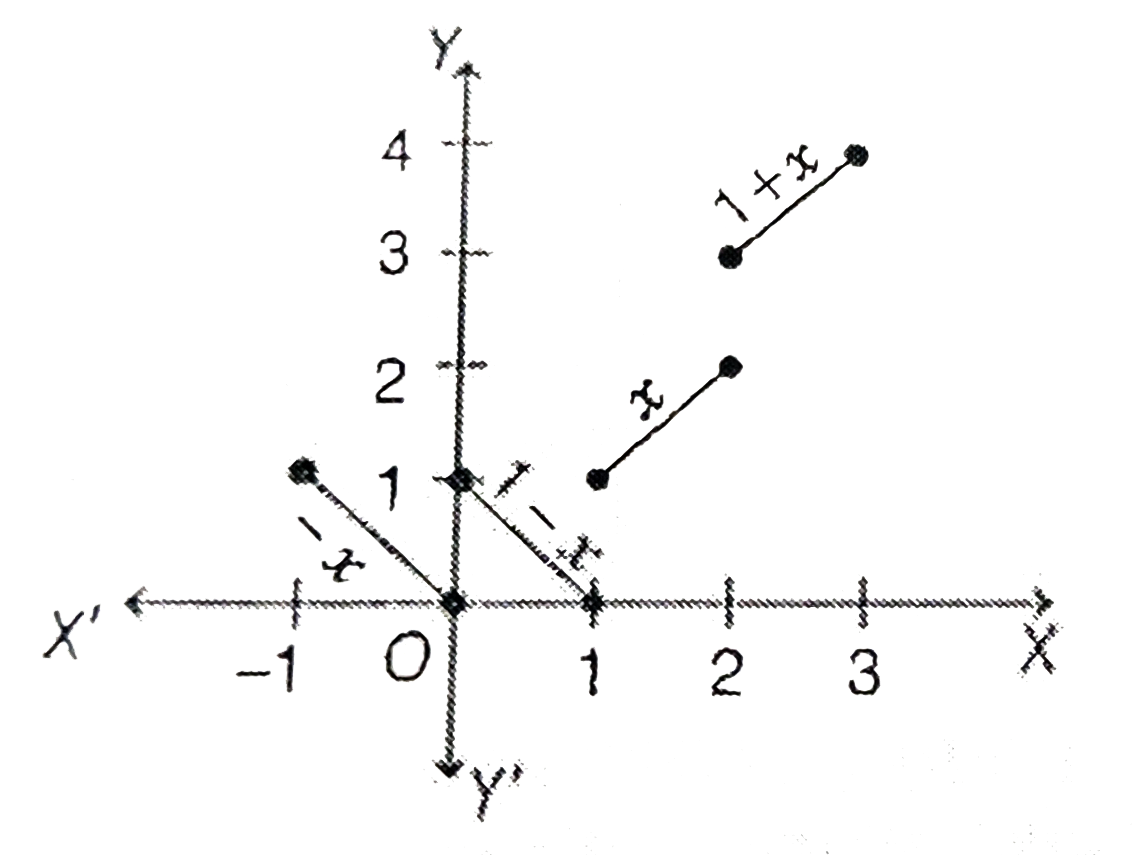
Clearly, from above figure, y is not continuous and not differentiable at x={0,1,2}.
Similar Questions
If Ri={x∈R:1≤x≤1+1i} then ∩5i=1Ri=
View Solutiony=[x]+|1−x|,−1≤x≤3 का लेखाचित्र बनाइए | वह बिंदु, जहाँ यह फलन अवकलनीय नहीं है, यदि है, तो ज्ञात कीजिए |
View SolutionDetermine the set of all integers x such that x=3(mod7), defined as x−3=7k (k is any integer) and −21≤x≤21
View SolutionDraw the graph of y=1{x}, where {⋅} denotes the fractional part function.
View SolutionLet f(x)=1+x, 0≤x≤2 and f(x)=3−x,2<x≤3. Find f(f(x)).
View SolutionMake a rough sketch of the region given below and find its area using integration {(x,y):0≤y≤x2+3,0≤y≤2x+3,0≤x≤3}.
View SolutionThe area between the curve 2{y}=[x]+1,0≤y<1, where {.}and[.] are the fractional part and greatest integer functions, respectively and the X-axis is
View SolutionLet 'f is a function, continuous on [0,1] such that f(x)≤√5∀x∈[0,12]andf(x)≤2x∀x∈[12,1] then smallest 'a' for which ∫10f(x)dx≤a holds for all 'f' is
View Solution