If the system of linear equations
x - 4y + 7z = g
3y - 5z = h
-2x + 5y - 9z = k
is consistent, then Solution in Tamil
2g + h +k = 0
g + 2h + k =0
g + h + k =0
g + h + 2k =0
(a) Here, D=∣∣
∣∣1−4703−5−25−9∣∣
∣∣
=1(−27+25)+4(0−10)+7(0+6) [expanding along R1]
=−2−40+42=0
∴ The system of linear equations have infinite many solutions.
[∵system of consistent and does not have unique solution as D = 0]
⇒D1=D2=D3=0
Now, D1=0⇒∣∣
∣
∣∣g−47h3−5k5−9∣∣
∣
∣∣=0
⇒g(−27+25)+4(−9h+5k)+7(5h−3k)=0
⇒−2g−36h+20k+35h−21k=0
⇒−2g−h−k=0⇒2g+h+k=0
Similar Questions
If the system of linear equations x−4y+7z=g, 3y−5z=h, −2x+5y−9z=k is consistent, then
View SolutionIf the bond energies of H-H, Br-Br and HBr are 433,192and364kJmol−1 respectively, then ΔH∘ for the reaction :
H2(g)+Br2(g)→2HBr(g) is
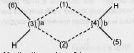
View SolutionWite down the corresponding elements in the figure : 1)……………… br 2)…………… br 3).……………. Br 4)………….. Br 5)…………. Br 6)…………..
View SolutionThe bond energies of H−−H , Br−−Br and H−−Br are 433,,192 and 364KJmol−1 respectively. The ΔH∘ for the reaction
H2(g)+Br2(g)→2HBr(g) is
View SolutionIf the end energies of H-H, Br-Br and H-Br are 433, 192 and 364 kJ mol−1 respectively, then ΔH∘ for the reaction, H2(g)+Br2(g)→2HBr(g) is
View SolutionIf the system of linear equations x−4y+7z=g, 3y−5z=h, −2x+5y−9z=k is consistent, then (a) g+2h+k=0 (b) g+h+2k=0 (c) 2g+h+k=0 (d) g+h+k=0
View SolutionIf the system of linear equations x-4y+7z=g , 3y-5z=h , -2x+5y-9z=k is consistent, then (a) g+2h+k=0 (b) g+h+2k=0 (c) 2g+h+k=0 (d) g+h+k=0
View SolutionBr(g)+e−→Br− के लिए ΔH का मान होगा
View Solution
