Find the area bounded by the curves x2+y2=25,4y=∣∣4−x2∣∣, and x=0 above the x-axis.Solution in Tamil
Video Solution
Text Solution
The correct Answer is:[4+25sin−1(45)] sq units
Given curves , x2+y2=25,4y=∣∣4−x2∣∣ could be sketched as below, whose points of intersection are
x2+(4−x2)216=25
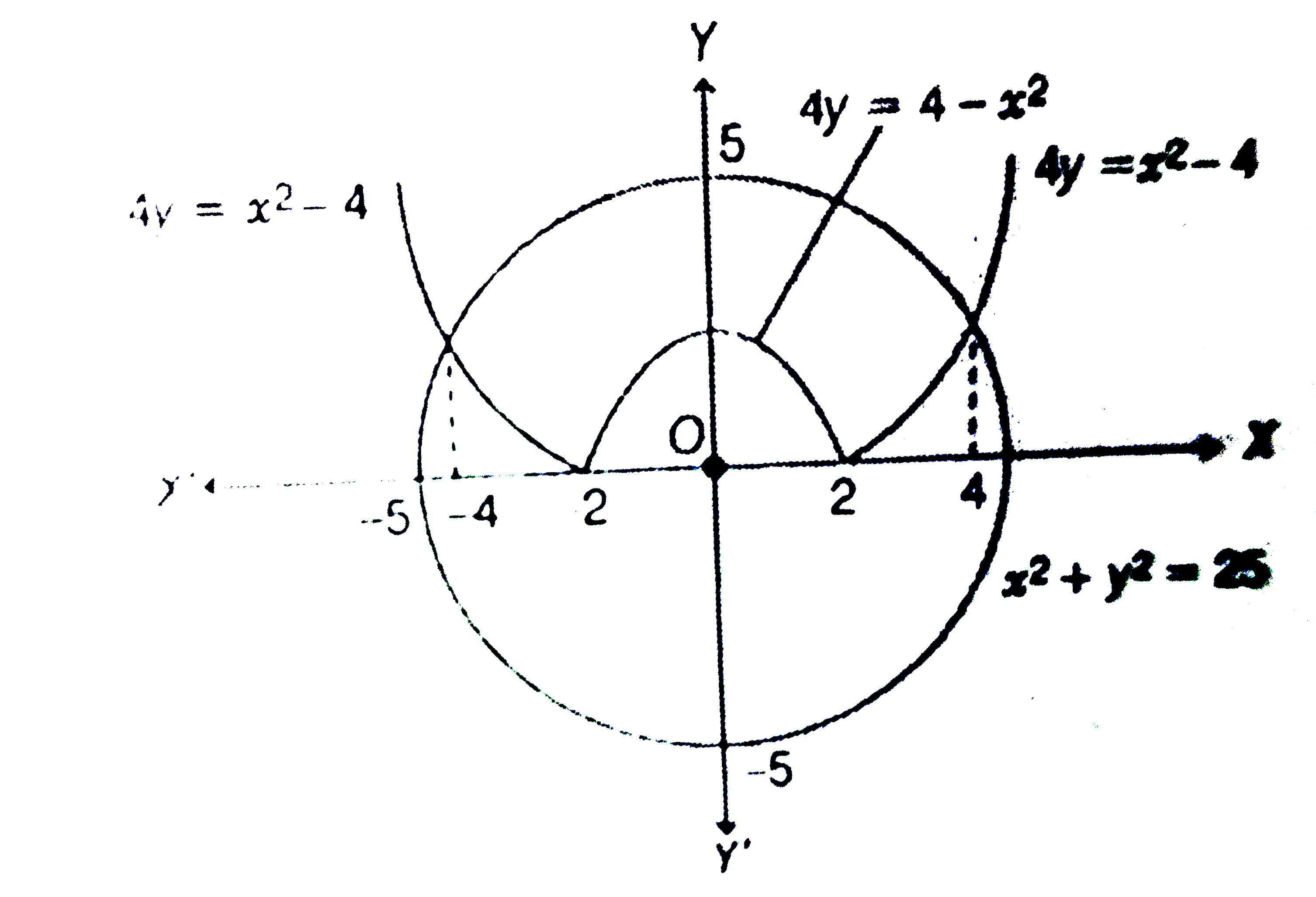
⇒(x2+24)(x2−16)=0
⇒x=±4
∴ Required area =2[∫40√25−x2dx−∫20(4−x24)dx−∫42(x2−44)dx]
=2[[x2√25−x2+252sin−1(x5)]40−14[4x−x33]20−14[x33−4x]42]
=2[[6+252sin−1(45)]14[8−83]−14[(643−16)−(83−8)]]
=2[6+252sin−1(45)−43−43−43]
=[4+25sin−1(45)] sq units
|
Similar Questions
वक्र x2+y2=25,4y=∣∣4−x2∣∣ और x = 0 द्वारा x-अक्ष के ऊपर घिरा क्षेत्रफल होगा
View SolutionThe area bounded by the curve x=y2+4y with y-axis is
View SolutionThe area bounded by the curve y=x24+x4−12 with X axis in [0, 2] is
View SolutionThe area bounded by the curve x=y2+4y with y-axis is
View SolutionFind the area bounded by the curves x2+y2=25,4y=∣∣4−x2∣∣, and x=0 above the x-axis.
View Solutionfind the area bounded by the curve y=2cosxand the X - axis from x=0 to x=2π
View Solutionवक्र x2+y2=25,4y=∣∣4−x2∣∣ और x = 0 द्वारा x-अक्ष के ऊपर घिरा क्षेत्रफल होगा
View SolutionFind the area bounded by the curve x=4−y2 and the Y-axis.
View Solution
