If the line x=α divides the area of region R={(x,y)∈R2:x3≤y≤x,0≤x≤1} into two equal parts, thenSolution in Tamil
2
43
13
23
The correct Answer is:A
We have,
A={(x,y):0≤y≤x|x|+1and−1≤x≤1}
When x≥0, then 0≤y≤x2+1
and when x<0, then 0≤y≤−x2+1
Now, the required region is the shaded region.
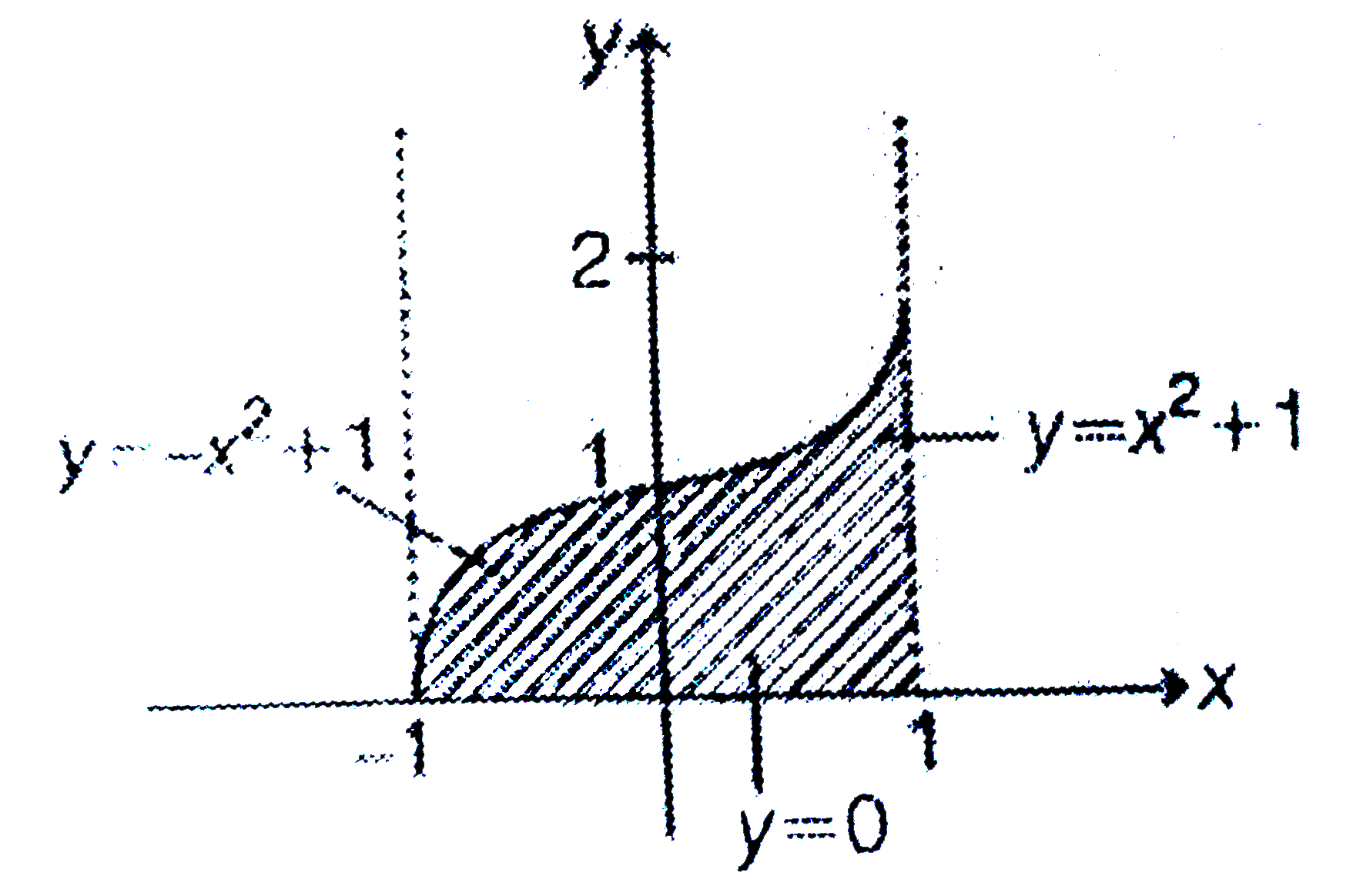
[∵y=x2+1⇒x2=(y−1), parabola with vertex (0, 1) and
y=−x2+1⇒x2=−(y−1),
Parabola with vertex (0, 1) but open downward ]
We need to calculate the shaded area, which is equal to
∫0−1(−x2+1)dx+∫10(x2+1)dx
=[−x33+x]0−1+[x33+x]10
=(0−[−(−1)33+(−1)])+([13+1]−0)
=−(13−1)+43
=23+43=2
Similar Questions
The are between x=y2 and x=4 is divided into two equal parts by the line x=a, find the value of a.
View SolutionThe area between x=y2 and x = 4 is divided into two equal parts by the line x = a find the value of a.
View SolutionThe area between x=y2 and x = 4 is divided into two equal parts by the line x= a, find the value of a.
View SolutionThe area between x=y2 and x=4 is divided into two equal parts by the line x=a, find the value of a.
View SolutionIf the line x=α divides the area of region R={(x,y)∈R2:x3≤y≤x,0≤x≤1} into two equal parts, then
View SolutionIf the line x=α divides the area of region R={(x,y)∈R2:x3≤y≤x,0≤x≤1} into two equal parts, then
View SolutionThe area between the curve y=x2, x-axis and the lines x=0 and x=2 is :
View SolutionThe area between x=y2 and x=4 is divided into two equal parts by the line x=a. Find the value of a.
View Solution
