Question

If two vertices of a triangle are $(0,2)$ and $(4,3)$ and its orthocentre is $(0,0)$ then the third vertex of the triangle lies in (a) $I^{st}$ quadrant (b) $2^{nd}rant\left(c\right)$3^(rd)quadrant (d) 4^(th)quadrant

Options

A. Fourth
B. Third
C. Scond
D. First

Answer

C

Explanation

Let ABC be a given triangle with vertices B(0, 2), C(4, 3) and let third vertex be A(a, b)

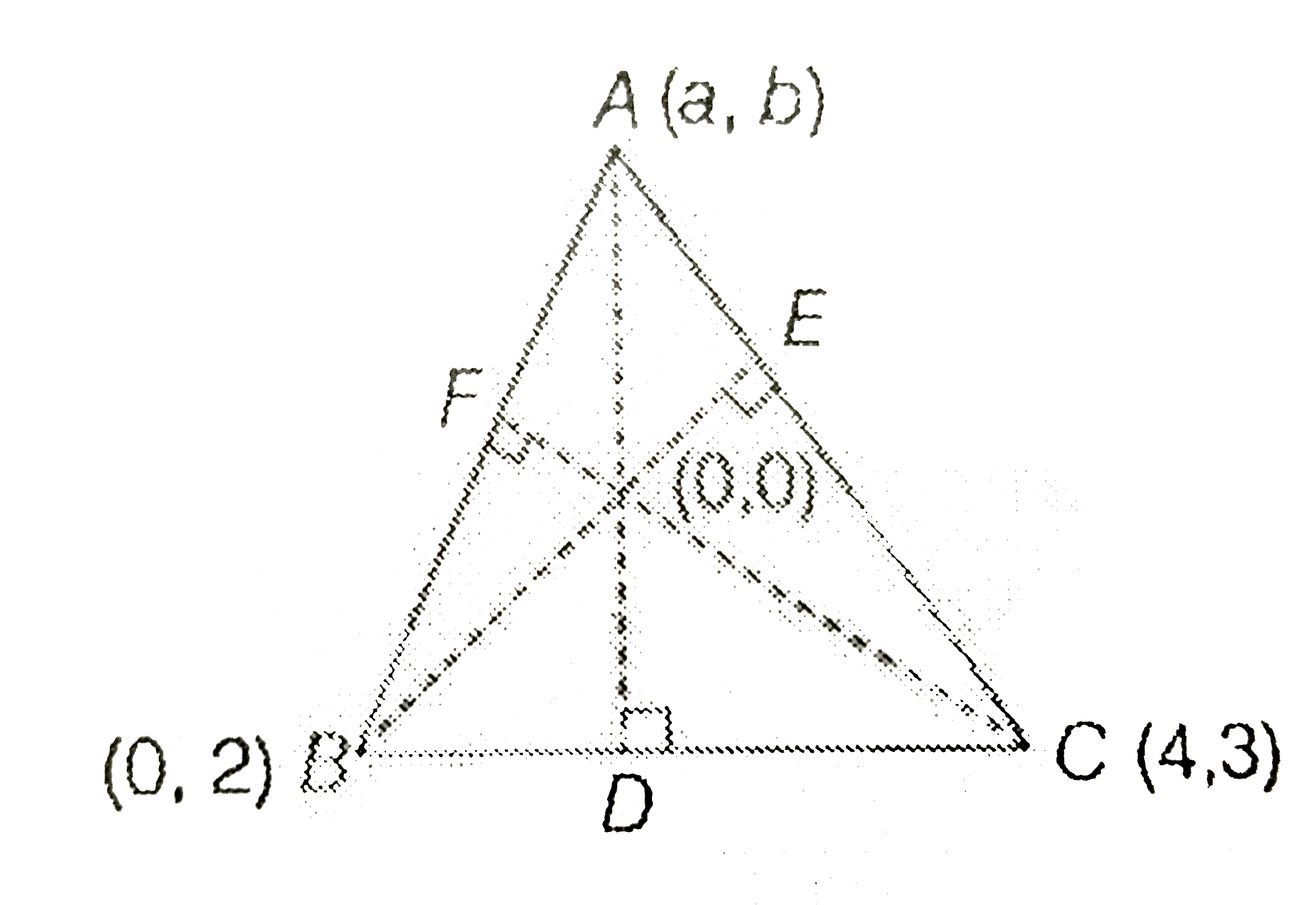
Also, let D, E and F are the foot of perpendiculars drawn from A, B and C respectivly.
Then, `ADbotBCrArr(b-0)/(a-0)xx(3-2)/(4-0)=-1`
[if two lines having slopes `m_(1)" and "m_(2)`are perpendicular then `m_(1)m_(2)=-1`]
`rArr" "b+4a=0`
and `CFbotAB`
`rArr" "(b-2)/(a-0)xx(3-0)/(4-0)=-1`
`rArr" "4a+3b=6`
From Eqs. (i) and (ii), we get
`-b=3b=6rArr2b=6`
`rArr" "b=3`
and `a=-3/4`
So, the third vertex
`(a, b )=(-3/4,3)`, which line in II quadrant.