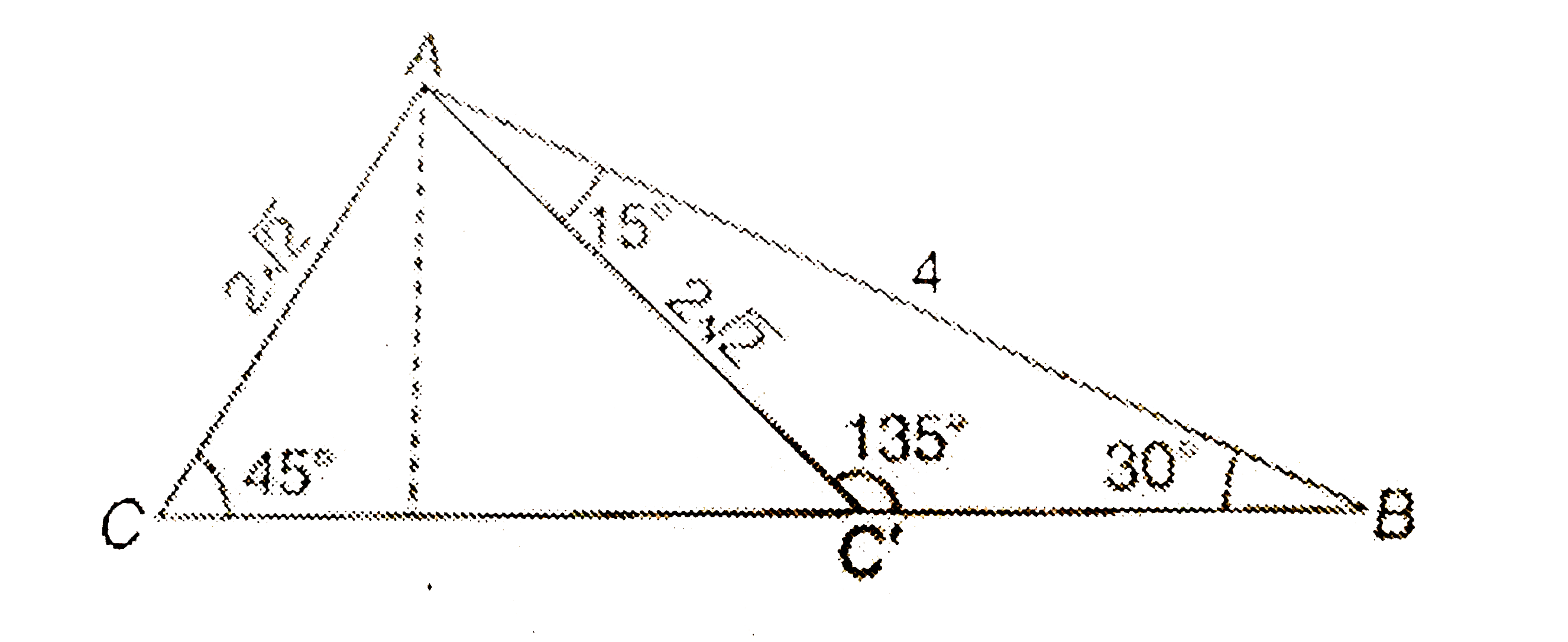
Let $A B C a n d A B C'$ be two non-congruent triangles with sides $A B = 4, A C = A C^{'} = 2 \sqrt{2}$ and angle $B = 30^{0}$ . The absolute value of the difference between the areas of these triangles isSolution in Tamil

Video Solution

Text Solution

Verified by Experts

The correct Answer is:4 sq units

In $D e < A B C,$ by sin rule, $\frac{a}{sin A} = \frac{2 \sqrt{2}}{{sin 30}^{\circ}} = \frac{4}{sin C}$
$\Rightarrow C = 45^{\circ}, C' = 135^{\circ}$
When $C = 45^{\circ} \Rightarrow A = 180^{\circ} - (45^{\circ} + 30^{\circ}) = 105^{\circ}$
When, $C' = 135^{\circ} \Rightarrow A = 180^{\circ} - (135^{\circ} + 30^{\circ}) = 15^{\circ}$

Area of $Δ A B C = \frac{1}{2} A B \times A C sin A$
$= \frac{1}{2} \times 4 \times 2 \sqrt{2} sin (105^{\circ})$
$= 4 \sqrt{2} \times \frac{\sqrt{3} + 1}{2 \sqrt{2}}$
$= 2 (\sqrt{3 + 1})$ sq. units
Area of $Δ A B C' = \frac{1}{2} A B \times A C sin A$
$= \frac{1}{2} \times 4 \times 2 \sqrt{2} sin (45^{\circ})$
$= 2 (\sqrt{3} - 1)$ sq. units
Difference of areas of triangle
$= ∣ ∣ 2 (\sqrt{3} + 1) - 2 (\sqrt{3} - 1) ∣ ∣ = 4$ sq units
Alternate Solution

Here, AD = 2, DC = 2
Difference of areas of $Δ A B C$ and $Δ A B C'$
= Area of $Δ A C C'$
$= \frac{1}{2} A D \times C C' = \frac{1}{2} \times 2 \times 4 = 4$ sq units.

Updated on:21/07/2023

|

Class 12 MATHS PROPERTIES OF TRIANGLES

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile