In triangle ABC,∠B=π3,and∠C=π4. Let D divided BC internally in the ratio 1:3. ThensiN∠BADsin∠CAB equals 1√6 (b) 13 (c) 1√3 (d) √23Solution in Tamil
1√6
13
1√3
√23
The correct Answer is:A
In ΔABD, applying since rule, we get
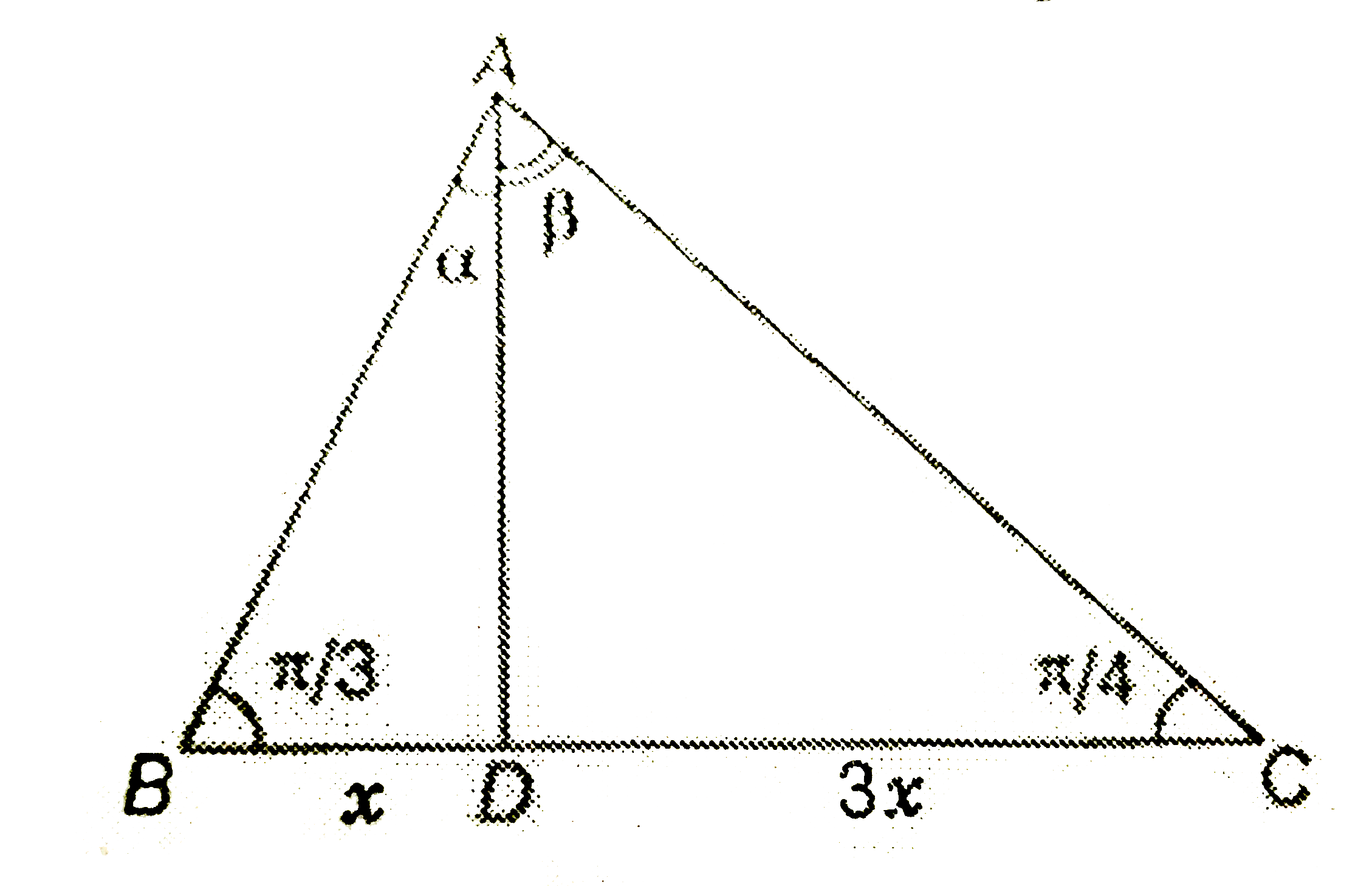
ADsinπ/3=xsinα
⇒AD=3√2xsinβ ....(i)
and in ΔACD, applying since rule, we get
ADsin/π=3xsinβ
⇒AD=3√2xsinβ ....(ii) From Eqs. (i) and (ii) , √3x2sinα=3x√2sinβ
⇒=sinαsinβ=1√6
Similar Questions
In triangle ABC,∠A=600,∠B=400,and∠C=800. If P is the center of the circumcircle of triangle ABC with radius unity, then the radius of the circumcircle of triangle BPC is (a)1 (b) √3 (c) 2 (d) √3 2
View SolutionIn triangle ABC,∠B=π3,and∠C=π4. Let D divided BC internally in the ratio 1:3. Thensin∠BADsin∠CAD equals (a)1√6 (b) 13 (c) 1√3 (d) √23
View SolutionIn a ΔABC,ifa=2,∠B=600and∠C=750 , then b= (a)√3 (b) √6 (c) √9 (d) 1+√2
View SolutionIn a △ABC, if 2∠A=3∠B=6 ∠C, determine ∠A, ∠B and ∠C
View SolutionIn a triangle ABC, the internal bisectors of ∠B and ∠C meet at P and the external bisectors of ∠B and ∠C meet at Q . Prove that ∠BPC+ ∠BQC=1800
View SolutionABC is a triangle in which ∠B=2∠C.D is a point on BC such that AD bisects ∠BAC and AB=CD. Prove that ∠BAC=72∘.
View Solution∞∑n=1sin−1(√n−(√n−1)√(n)(n+1)= (A) π4 (B) π2 (C) −π3 (D) π3
View SolutionIn triangle ABC,∠A=600,∠B=400,and∠C=800. If P is the center of the circumcircle of triangle ABC with radius unity, then the radius of the circumcircle of triangle BPC is (a)1 (b) √3 (c) 2 (d) √3 2
View Solution