Tangents are drawn to the hyperbola 4x2−y2=36 at the points P and Q. If these tangents intersect at the point T(0,3) then the area (in sq units) of △PTQ isSolution in Tamil
45√5
54√3
60√3
36√5
Tangents are drawn to the hyperbola 4x2−y2=36 at the point P and Q.
Tangent intersects at point T(0,3)
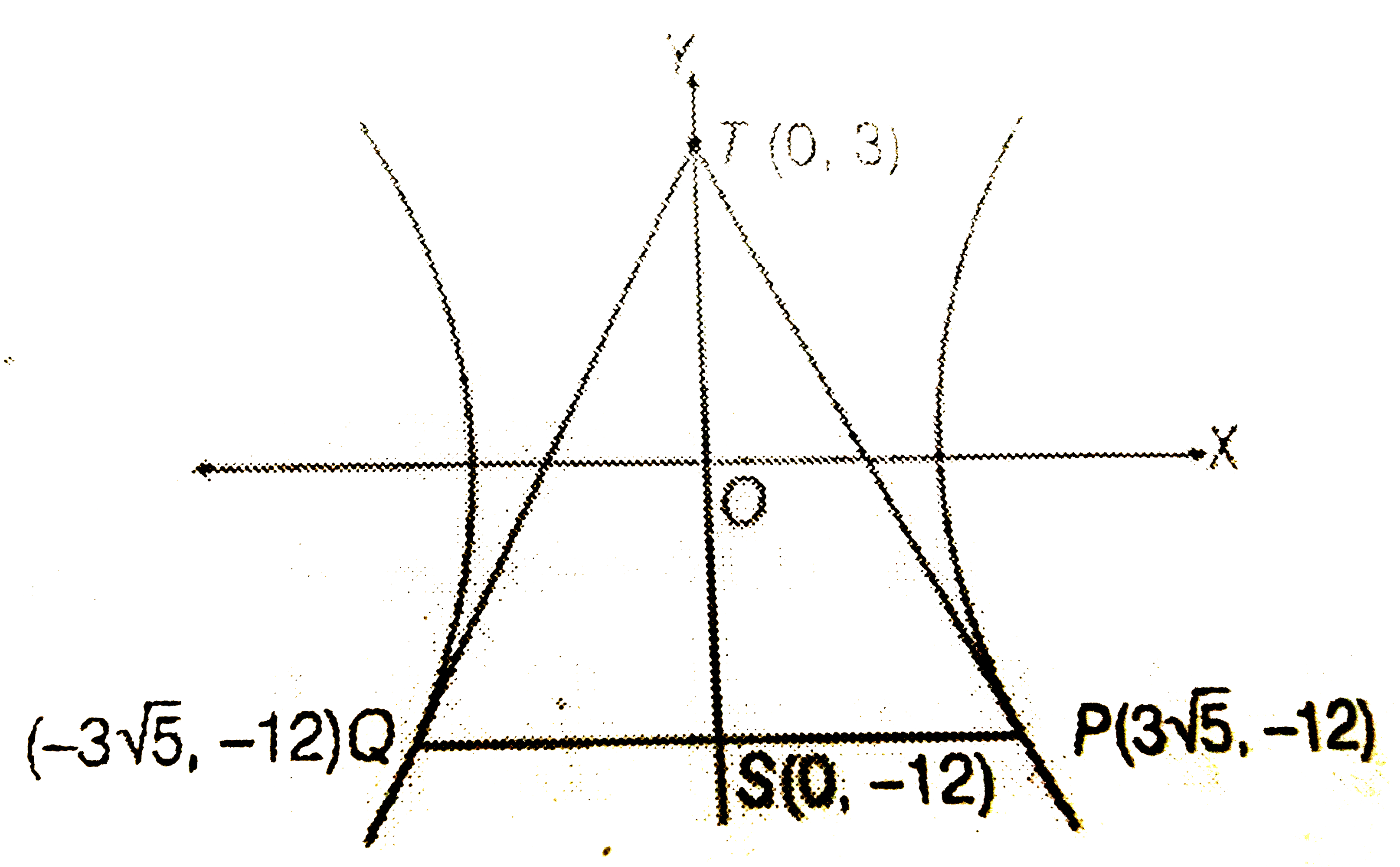
Clearly, PQ is chord of contact.
∴ Equation of PQ is −3y=36
⇒y=−12
Solving the curve 4x2−y2=36andy=−12,
we get x=±3√5
Area of △PQT=12×PQ×ST=12(6√5×15)=45√5
Similar Questions
Tangent are drawn at points P and Q to the hyperbola 4x2−y2=36. They intersects of point T (0, 3). Then area of ΔPTQ =…….. Sq.
View Solutionएक अतिपरवलय 4x2−y2=36 के बिन्दुओं P तथा Q पर स्पर्श रेखाएँ खींची जाती हैं। यदि यह स्पर्श रेखाएँ बिन्दु T(0, 3)पर काटती है, तो ΔPTQ का क्षेत्रफल (वर्ग इकाइयों में) है:
(i) 60√3 (ii) 36√5 (iii) 45√5 (iv) 54√5
View SolutionTangents are drawn to the hyperbola 4x^2-y^2=36 at the points P and Q. If these tangents intersect at the point T(0,3) then the area (in sq units) of triangle PTQ is
View Solutionएक अतिपरवलय 4x2−y2=36 के बिंदुओं P तथा Q पर स्पर्श रेखाएँ खींची जाती हैं | यदि यह स्पर्शरेखाएँ बिन्दु T(0,3) पर काटती हैं, तो ΔPTQ का क्षेत्रफल (वर्ग इकाइयों में) है :
View Solutionयदि परवलय के बिन्दु P व Q पर खींची गयी स्पर्श रेखाओं T पर काटती हैं, तो SP, ST व SQ हैं
View SolutionTangents are drawn from the point (-1, 2) to the parabola y2=4x The area of the triangle for tangents and their chord of contact is
View SolutionTangents are drawn from the point (-1, 2) to the parabola y2=4x The area of the triangle for tangents and their chord of contact is
View SolutionA tangent is drawn at any point on the hyperbola (x^(2))/(a^(2))-(y^(2))/(b^(2)) =1 . If this tangent is intersected by the tangents at the vertices at points P and Q, then which of the following is/are true
View Solution