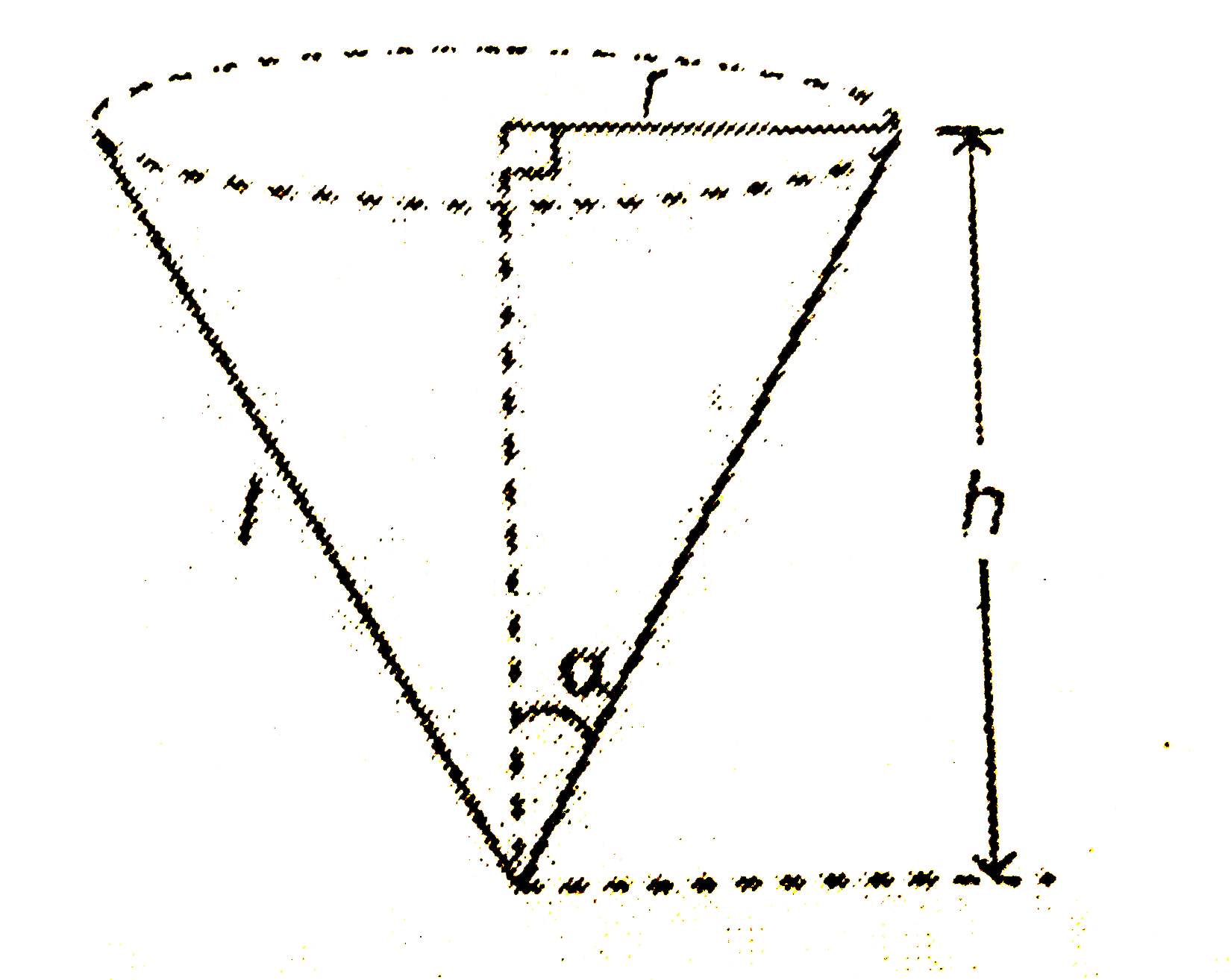
A water tank has the shape of an inverted right circular cone with its axis vertical and vertex lowermost. Its semi-vertical angle is tan−1(0.5). Water is poured into it at a constant rate of 5 cubic metre per hour. Find the rate at which the level of the water is rising at the instant when the depth of water in the tank is 4 m.Solution in Tamil
2π
15π
115π
110π
The correct Answer is:B
Given, semi-vertical angle of right circular cone
tan−1(12)
Let α=tan−1(12)
⇒tanα=12
⇒rh=12 [ from fig. tanα=rh]
⇒ r=12h ... (i)
∵ Volume of cone is (V)=13πr2h
∴V=13π(12h)2(h)=112πh3 [ from Eq. (i)]
On differentiating both sides w.r.t. 't', we get
dVdt=112π(3h2)dhdt
⇒dhdt=4πh2dVdt
⇒dhdt=4πh2×5 [ ∵ given dVdt=5m3/min]
Now, at h=10 m, the rate at which height of water level is rising =dhdt∣∣∣h=10
4π(10)2×5=15πm/min
Similar Questions
A water tank has a shape of an inverted right circular cone with its axis vertical and vertex lower-most. Its semi-vertical angle is tan−1(34) . Water is poured into it at a constant rate of 3 cubic meter per hour. The rate at which the level of the water is rising at an instant when the depth of water in the tank is 4m is
View SolutionA water tank has the shape of an inverted right circular cone with its axis vertical and vertex lowermost. Its semi-vertical angle is tan−1(0.5). Water is poured into it at a constant rate of 5 cubic metre per hour. Find the rate at which the level of the water is rising at the instant when the depth of water in the tank is 4 m.
View SolutionA water tank has the shape of an inverted right circular cone with its axis vertical and vertex lowermost. Its semi-vertical angle is tan−1(0.5). Water is poured into it at a constant rate of 5 cubic metre per hour. Find the rate at which the level of the water is rising at the instant when the depth of water in the tank is 4m.
View SolutionA water tank has the shape of an inverted right circular cone with its axis vertical and vertex lowermost. Its semi-vertical angle is tan−1(0.5) . Water is poured into it at a constant rate of 5 cubic metre per hour. Find the rate at which
View SolutionA water tank has the shape of an inverted right circular cone with its axis vertical and vertex lowermost. Its semi-vertical angle is tan−1(0.5). Water is poured into it at a constant rate of 5 cubic meter per hour. Find the rate at which the level of the water is rising at the instant when the depth of water in the tank is 4 m.
View Solutionਪਾਣੀ ਦੀ ਇੱਕ ਟੈਂਕੀ ਦਾ ਆਕਾਰ, ਲੰਬ ਧੁਰੇ ਵਾਲੇ ਇੱਕ ਉਲਟੇ ਲੰਬਕਾਰੀ ਸ਼ੰਕੂ ਵਰਗਾ ਹੈ ਜਿਸ ਦਾ ਸਿਖਰ ਹੇਠਾਂ ਹੈ। ਇਸ ਦਾ ਅਰਧ ਸਿਖਰ ਕੋਣ tan−1(0.5) ਹੈ। ਇਸ ਵਿੱਚ 0.2m3h ਦੀ ਦਰ ਨਾਲ ਪਾਣੀ ਭਰਿਆ ਜਾਂਦਾ ਹੈ। ਪਾਣੀ ਦੇ ਪੱਧਰ ਦੇ ਵਧਣ ਦੀ ਦਰ ਇਸ ਪਲ ਪਤਾ ਕਰੋ ਜਦੋਂ ਟੈਂਕੀ ਵਿੱਚ ਪਾਣੀ ਦੀ ਉਚਾਈ 1m ਹੈ।
View SolutionA water tank has the shape of an inverted right circular cone with its axis vertical and vertext lowermost. Its semi-vertical angle is tan−1(0.5). Water is poured into it at a constant rate of 5 cubic metre per hour. Find the rate at which the level of the water is rising at the instant when the depth of water in the tank is 4m.
View SolutionA water tank has the shape of an inverted righ circular cone with its axis vertical and vertex lowermost . Its semi-vertical angle is tan−1(0.5) . Water is poured into it at a constant rate of 4 cubic meter per hour . Find the rate at which the level of the water is rising at the instant when the depth of water in the tank is 2 m.
View Solution
