about to only mathematics Solution in Tamil
Video Solution
Text Solution
Let the coordinates of point P be (acosθ,bsinθ).
then , equation of tangent at P is
xacosθ+ybsinθ=1
we have ,d= length fo perpendicular from O to the tangent at P ,
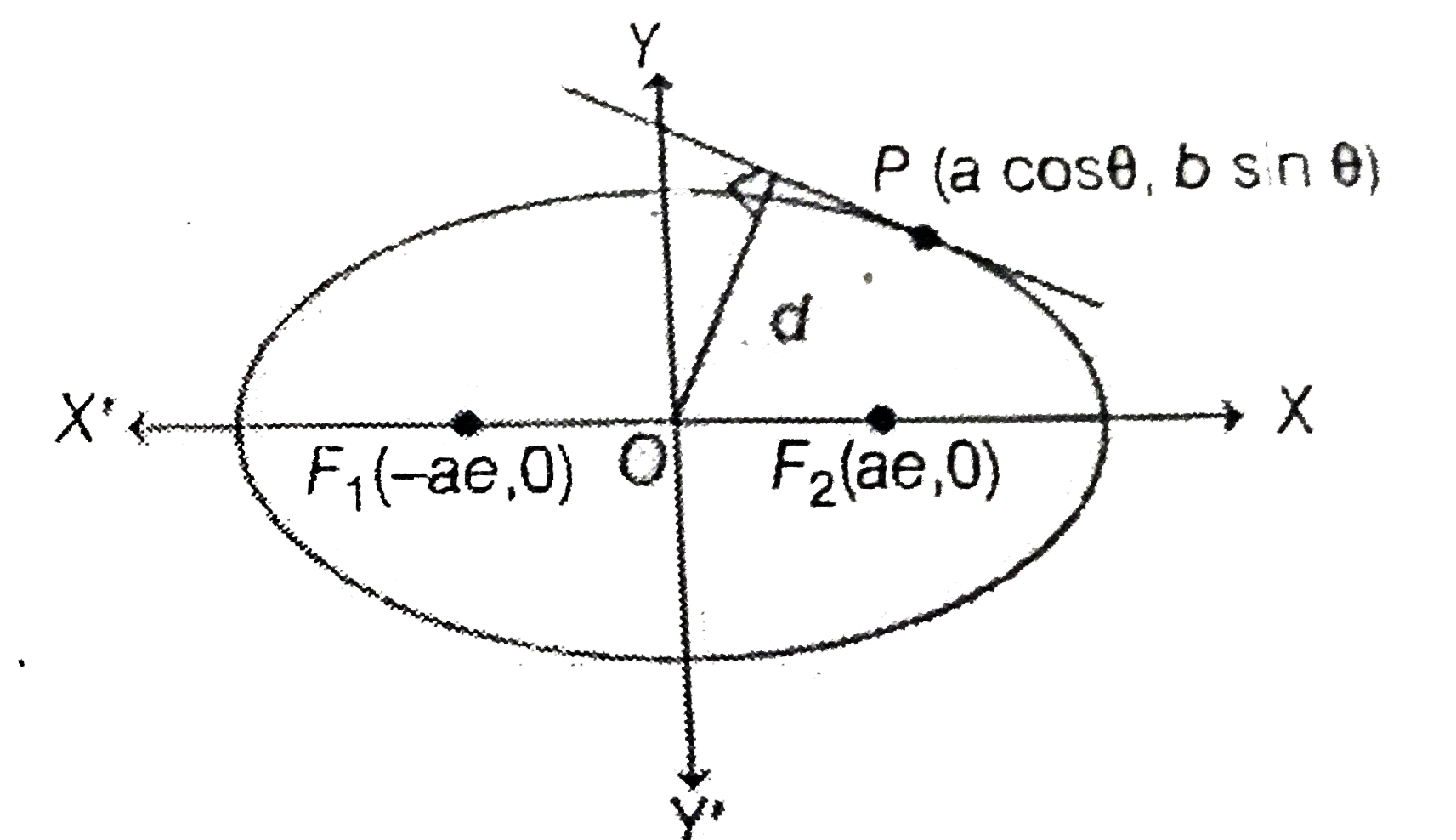
d=|0+0−1|√cos2θa2+sin2θb2
1d=√cos2θa2+sin2θb2
⇒1d2=cos2θa2+sin2θb2
we have to prove (PF1−PF2)2=4a2(1−b2d2)
Now , RHS=4a2(1−b2d2)=4a2−4a2b2d2
=4a2−4a2b2(cos2θa2+sin2θb2)
=4a2−4b2cos2θ−4a2sin2θ
=4a2(1−sin2θ)−4b2cosθ
=4a2cos2θ−4b2cos2θ−4b2cos2θ
=4cos2θ(a2−b2)=4cos2θ.a2e2[∵e=√2−(b/a)2
Again PF1=e|acosθ+a/ee|=a|ecosθθ+1|
=a(ecosθ+1)
[∵−1≤cosθ1and0<e<1]
Similarly , PF2=a(1−ecosθ)
Therefore,LHS=(PF1−PF2)2
=[a(ecosθ+1)−a(1−ecosθ)]2
=(aecosθ+α−a+aecosθ)2
=(2aecosθ)2=4a2e2cos2θ
hence LHS=RHS
|
Similar Questions
about to only mathematics
View Solutionabout to only mathematics
View Solutionabout to only mathematics
View Solutionabout to only mathematics
View Solutionabout to only mathematics
View SolutionWhich of the following statements is not correct about assignments in Mathematics?
View SolutionLearning Mathematics at upper primary level is about
View SolutionLearning mathematics at upper primary level is about
View Solution