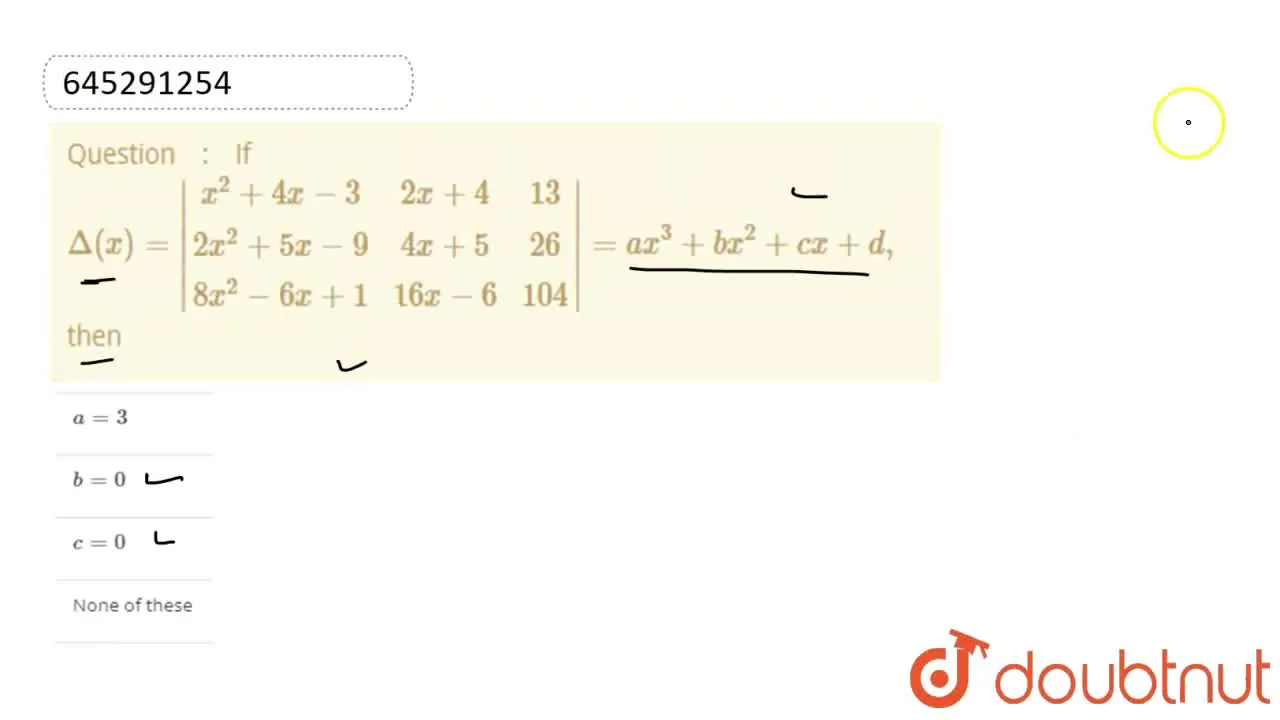
If $Δ (x) = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{2} + 4 x - 3 & 2 x + 4 & 13 2 x^{2} + 5 x - 9 & 4 x + 5 & 26 8 x^{2} - 6 x + 1 & 16 x - 6 & 104 \end{matrix} ∣ ∣ ∣ ∣ ∣ = a x^{3} + b x^{2} + c x + d,$ then
Solution in Tamil

A

$a = 3$

B

$b = 0$

C

$c = 0$

D

None of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B, C

$Δ' (x) = ∣ ∣ ∣ ∣ \begin{matrix} 2 x + 4 & 2 x + 4 & 13 4 x + 5 & 4 x + 5 & 26 16 x \pm 6 & 16 x - 6 & 104 \end{matrix} ∣ ∣ ∣ ∣ + ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{2} + 4 x - 3 & 2 & 13 2 x^{2} + 5 x - 9 & 4 & 26 8 x^{2} - 6 x + 1 & 16 & 104 \end{matrix} ∣ ∣ ∣ ∣ ∣$
$= 0 + 2 \times 13 \times (0) = 0$
$\Rightarrow Δ (x) = constant \Rightarrow a = 0, b = 0 . c = 0$

Updated on:21/07/2023

|

Class 12 MATHS DETERMINANTS

Topper's Solved these Questions

Similar Questions

CENGAGE-DETERMINANTS-All Questions

Home
Ask Doubt
Profile