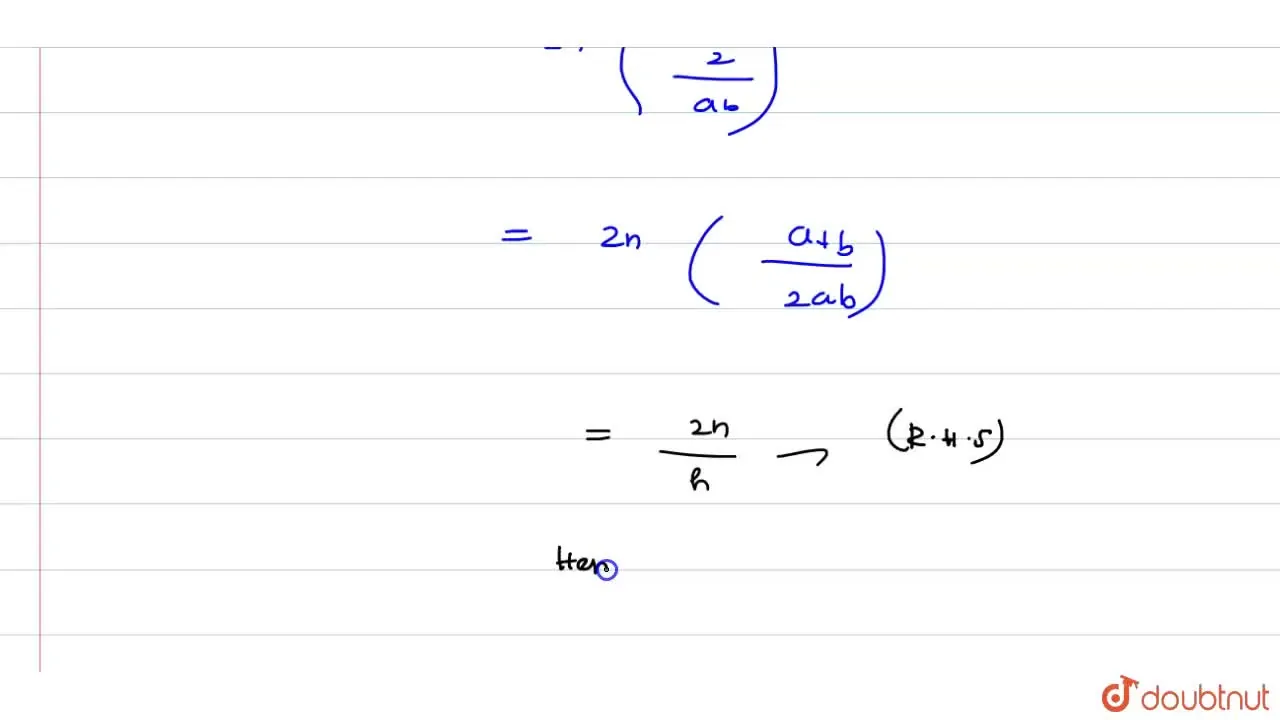If a,a1,a2,a3,a2n,b are in A.P. and a,g1,g2,g3,,g2n,b . are in G.P. and h s the H.M. of aandb, then prove that a1+a2ng1g2n+a2+a2n−1g1g2n−1++an+an+1gngn+1=2nhSolution in Tamil
Here,
a+b=a1+a2n=a2+a2n−1=….=an+an+1
ab=g1×g2n=g2×g2n−1=…=gn×gn+1
⇒andh=2aba+b
∴ Sum of given series=n(a+b)ab=2nh
Related Playlists
- BOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - Exercise 5.74videos
PROGRESSION AND SERIES
- BOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - Exercise 5.810videos
PROGRESSION AND SERIES
- BOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - Exercise 5.510videos
PROGRESSION AND SERIES
- BOOK - CENGAGECHAPTER - PROBABILITY IIEXERCISE - JEE Advanced Previous Year25videos
PROBABILITY II
- BOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - JEE Advanced Previous Year11videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
Similar Questions
If α(≠1) is a nth root of unity then S=1+3α+5α2+..........upto n terms is equal to
09:36View SolutionFind the sum to n terms of the series 1×2×3+2×3×4+3×4×5+.
02:33View SolutionA G.P. consists of an even number of terms. If the sum of all the terms is 5 times the sum of terms occupying odd places, then find its common ratio.
06:04View SolutionA signal which can be green or red with probability 45and15 respectively, is received by station A and then and 3 transmitted to station B. The probability of each station receiving the signal correctly is 34 If the signal received at station B is green, then the probability that the original signal was green is (a) 35 (b) 67 (c) 2023 (d) 920
04:13View SolutionLet ABC be a triangle such that ∠ACB=π6 and let a,bandc denote the lengths of the side opposite to A,B,andC respectively. The value(s) of x for which a=x2+x+1,b=x2−1,andc=2x+1 is(are) −(2+√3) (b) 1+√3 2+√3 (d) 4√3
04:46View SolutionGiven that (1+x+x^2)^n=a_0+a_1x+a_2x^2+.....+a_(2n)x^(2n) find i) a_0 + a_1 +a_2 .. . . .+ a_(2n) ii) a_0 - a_1 + a_2 - a_3 . . . . + a_(2n) iii) (a_0)^2 - (a_1)^2 . . . . .+ (a_(2n))^2
04:48View Solutionif a,a_(1),a_(2),a_(3),......,a_(2n),b are in A.P. and a,g_(1),g_(2),............g_(2n),b are in G.P. and h is H.M. of a,b then (a_(1)+a_(2n))/(g_(1)*g_(2n))+(a_(2)+a_(2n-1))/(g_(2)*g_(2n-1))+.........+(a_(n)+a_(n+1))/(g_(n)*g_(n+1)) is equal
04:38View SolutionIf a1,a2,a3,……..an,an+1,…….. be A.P. whose common difference is d and S1=a1+a2+……..+an,S2=an+1+………..+a2n,S3=a2n+1+…………+a3n etc show that S1,S2,S3,S4………… are in A.P. whose common difference is n2d.
05:33View SolutionLet the sequence a_1 , a_2 , a_3 ......... a_n form an A.P. then a_1^2 - a_2^2 + a_3^2 - a_4^2 +.....+ a_(2n-1)^2 - a_(2n)^2 is equal to:- (1) n/(2n-1)(a_1^2-a_(2n)^2) (2) (2n)/(n-1)(a_(2n)^2-a_1^2) (3) n/(n+1)(a_1^2+a_(2n)^2) (4)none of these
06:15View SolutionLet the sequence a_1 , a_2 , a_3 ......... a_n form an A.P. then a_1^2 - a_2^2 + a_3^2 - a_4^2 +.....+ a_(2n-1)^2 - a_(2n)^2 is equal to:- (1) n/(2n-1)(a_1^2-a_(2n)^2) (2) (2n)/(n-1)(a_(2n)^2-a_1^2) (3) n/(n+1)(a_1^2+a_(2n)^2) (4)none of these
03:22View SolutionIf a1,a2,a3….a2n+1 are in A.P then
a2n+1−a1a2n+1+a1+a2n−a2a2n+a2+....+an+2−anan+2+an is equal to05:47View Solutionयदि a,a1,a2…..a2n,bसमान्तर श्रेणी में है तथा a,g1,g2……g2n,b गुणोत्तर श्रेणी में है तथा h, a व b का हरात्मक मध्य है तब a1+a2ng1g2n+a2+a2n−1g2g2n−1+…..+an+an+1gngn+1=
05:38View SolutionIf the sequence a1,a2,a3,.......an,. forms an A.P., then prove that a21−a22+a23−a24+.......+a22n−1−a22n=n2n−1(a21−a22n)
04:14View SolutionIf a1,a2,a3,,a2n+1 are in A.P., then a2n+1−a1a2n+1+a1+a2n−a2a2n+a2++an+2−anan+2+an is equal to a. n(n+1)2×a2−a1an+1 b. n(n+1)2 c. (n+1)(a2−a1) d. none of these
04:03View SolutionIf a1,a2,a3….a2n+1 are in A.P then
a2n+1−a1a2n+1+a1+a2n−a2a2n+a2+....+an+2−anan+2+an is equal to04:51View SolutionIf ar>0,r∈N and a1.a2,....a2n are in A.P then a1+a2√a1+√a2+a2+a2n−1√a2+√a3+.....+an+an+1√an+√an+1=
07:49View Solution