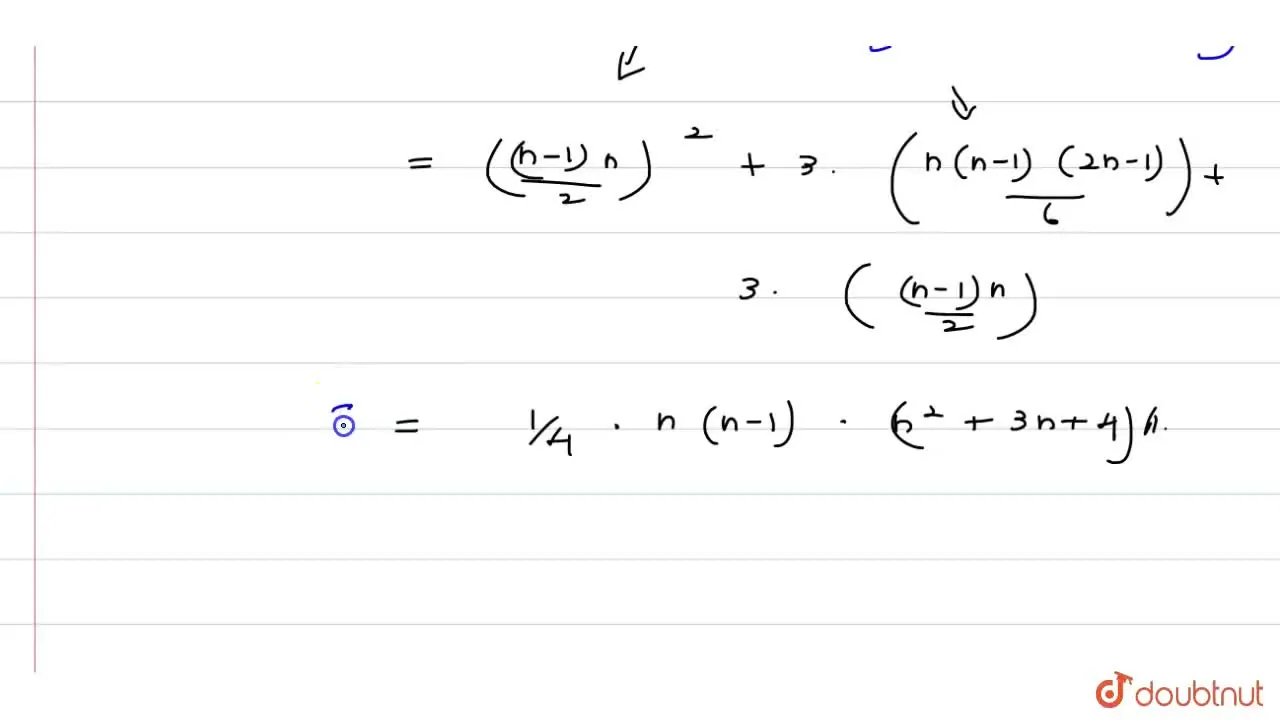The value of the expression 1.(2−ω).(2−ω2)+2.(3−ω)(3−ω2)+.+(n−1)(n−ω)(n−ω2), where omega is an imaginary cube root of unity, is………Solution in Tamil
S=1×(2−ω)×(2−ω2)+2×(3−ω)×(3−ω)×(3−ω2)+…+(n−1)×(n−ω)×(n−ω2)
Here, Tn=(n−1)×(n−ω)×(n−ω2)=n3−1
∴S=n∑n=2(n3−1)
=n∑n=1(n3−1)
=n2(n+1)24−n
=n2(n2+2n+1)−4n4
14n(n3+2n2+n−4)
=12n(n−1)(n2+3n+4)
Related Playlists
- BOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - Exercise 5.13videos
PROGRESSION AND SERIES
- BOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - Exercise 5.210videos
PROGRESSION AND SERIES
- BOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - Multiple Correct Answer4videos
PROGRESSION AND SERIES
- BOOK - CENGAGECHAPTER - PROBABILITY IIEXERCISE - JEE Advanced Previous Year25videos
PROBABILITY II
- BOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - JEE Advanced Previous Year11videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
Similar Questions
Write the first five terms of each of the sequences and obtain the corresponding series:
a1=a2=2,an=an−1−1,n>206:54View SolutionIf the pth term if an A.P. is q and the term of an A.P is p then the rth term is
06:57View SolutionIf a,x, and b and b are in A.P .., a,y , and a,z,b are in H.P such that x=9z and a>0,b>0 then
08:57View SolutionA signal which can be green or red with probability 45and15 respectively, is received by station A and then and 3 transmitted to station B. The probability of each station receiving the signal correctly is 34 If the signal received at station B is green, then the probability that the original signal was green is (a) 35 (b) 67 (c) 2023 (d) 920
03:50View SolutionLet ABC be a triangle such that ∠ACB=π6 and let a,bandc denote the lengths of the side opposite to A,B,andC respectively. The value(s) of x for which a=x2+x+1,b=x2−1,andc=2x+1 is(are) −(2+√3) (b) 1+√3 2+√3 (d) 4√3
03:53View SolutionThe value of the expression (1+(1)/(w))(1+(1)/(w^(2)))+(2+(1)/(w))(2+(1)/(w^(2)))+(3+(1)/(w))(3+(1)/(w^(2)))+......+(n+(1)/(w))(n+(1)/(w^(2))) where w is an imaginary cube root of unity
01:40View SolutionIf omega is a cube root of unity then the value of the expression 2(1+w)(1+w^(2))+3(1+w)(1+w^(2))+....+(n+1)(n+w)(n+w^(2))
04:15View SolutionThe value of the expression (1+1/omega)(1+1/omega^(2))+(2+1/omega)(2+1/omega^(2))+(3+1/omega)(3+1/omega^(2))+…………..+(n+1/omega)(n+1/omega^(2)) , where omega is an imaginary cube root of unity, is
04:13View SolutionThe value of the expression ( 1 + (1)/(omega)) ( 1 + (1)/(omega^(2))) + (2 + (1)/(omega)) (2 + (1)/(omega^(2))) + (3 + (1)/(omega)) (3 + (1)/(omega^(2))) + …… + ((n + (1))/(omega)) (n + (1)/(omega^(2))) Where omega is an imaginary cube root of unity, is
05:15View SolutionThe value of the expression , (2−w)(2−w2)+2.(3−w)(3−w2)+.............+(n−1).(n−w)(n−w2), where w is an imaginary cube root of unity is
09:16View SolutionIf ω is an imaginary cube root of unity then the value of
(2−ω),(2−ω2)+2(2−ω)(3−ω2)+....+(n−1)(n−ω)(n−ω2) is05:18View SolutionIf ω is an imaginary cube root of unity. Find the value of the expression 1(2−ω)(2−ω2)+2(3−ω)+...+(n−1)(n−ω)(n−ω2).
View SolutionIn a survey of 60 people, it was found that 25 people read newspaper H. 26 read newspaper T, 26 read newspaper 1, 9 read both H and I. 11 read both H and T, 8 read both T and I, 3 read all three newspapers. Find: (i) the number of people who read at least one of the newspapers. (ii) the number of people who read exactly one newspaper
02:54View Solution1 -এর কাল্পনিক ঘনমূল ω হলে, 1⋅(2−ω)(2−ω2)+2⋅(3−ω)(3−ω2)+⋅⋅⋅+(n−1)(n−ω)(n−ω2) -এর মান নির্ণয় করো।
04:26View Solutionव्यंजक 1.(2−ω)(2−ω2)+2.(3−ω)(3−ω2)+…..+(n−1).(n−ω)(n−ω2), जहाँ ω इकाई का एक काल्पनिक घनमूल है, का मान है
05:46View Solutionयदि ω इकाई का एक काल्पनिक घनमूल है तो 1(2−ω)(2−ω2)+2(3−ω)(3−ω2)+…+ (n−1)(n−ω)(n−ω2) का मान है
06:04View Solution