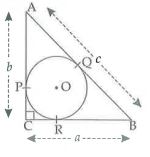
A circle having centre as O' and radius r' touches the incircle of ΔABC externally at. F, where F is on BC and also touches its circumcircle internally at G. It O is the circumcentre of ΔABC and I is its incentre, then Solution in Tamil
OO'=R-r'
Perpendicular distance from O to line joining IO' is ∣∣∣b−c2∣∣∣
Projection of OO' on line joining IO'=r'+R cos A
r'=Δatan2A
The correct Answer is:A, B, C, D
From figure, OO' = OG - O'G = R-r'

OE=DE=|BF−BD|=∣∣∣s−b−a2∣∣∣=|c−b|2
OD = EF = R cos A
From ΔOEO', using Pythagoras theorem, we get
(R−r')2=(RcosA+r')2+(c−b2)2
⇒r'=bc4Rtan2A2
=Δatan2A2
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - SOLUTIONS AND PROPERTIES OF TRIANGLE EXERCISE - SINGLE CORRECT ANSWER TYPE42videos
SOLUTIONS AND PROPERTIES OF TRIANGLE
- BOOK - CENGAGECHAPTER - SOLUTIONS AND PROPERTIES OF TRIANGLE EXERCISE - Comprehension Type6videos
SOLUTIONS AND PROPERTIES OF TRIANGLE
- BOOK - CENGAGECHAPTER - SETS AND RELATIONSEXERCISE - Question Bank3videos
SETS AND RELATIONS
- BOOK - CENGAGECHAPTER - STATISTICSEXERCISE - JEE Previous Year10videos
STATISTICS
Similar Questions
In a triangle ∠A=55∘,∠B=15∘,∠C=110∘. Then c2−a2 is equal to
08:12View SolutionLet ABC be a triangle in which the line joining the circumecentre and incentre is parallel to base BC of the triangle. Then answer the following questions :
Then range of ∠A is08:22View SolutionLet n(U)=700, n(A)=200, n(B)=300 and n(A∩B)=100, then n(Ac∩Bc)=
05:18View SolutionIf the mean deviation of number 1,1+d, 1+2d,…., 1+100d from their mean is 255, then d is equal to
09:57View SolutionThe sides AB,BC and AC of a triangle ABC touch a circle with centre O and radius r at P Q,and R respectively.
09:57View SolutionIf a triangle ABC, the incircle touches the sides BC, CA and AB respectively at D, E and F. If the radius of incircle is 4 units and if BD, CE and AF are consecutive integers, Find the lengths of sides of the triangle ABC.
View SolutionRead the following passage and answer the questions that follow ABC is a triangle,the incircle touches the sides BC,CA and ABatD,E and F respectively. BD,CE and AF are consecutive natural numbers.I is the incentre of the triangles.The radius of the incircle is 4units.
View SolutionA circumcircle is a circle which passes through all vertices of a triangle and an incircle is a circle which is inscribed in a triangle touching all sides of a triangle. Let ABC be a right-angled triangle whose radius of the circumcircle is 5 and its one side AB = 6. The radius of incircle of triangle ABC is r. Area of Delta ABC is
04:12View SolutionA circumcircle is a circle which passes through all vertices of a triangle and an incircle is a circle which is inscribed in a triangle touching all sides of a triangle. Let ABC be a right angled triangle whose radius of circumcircle is 5 and its one side AB = 6. The radius of incircle of triangle ABC is r. The value of r is
View SolutionIn ΔABC,∠A=π3 and its inradius of 6 units. Find the radius of the circle touching the sides AB, AC internally and the incircle of ΔABC externally
02:17View SolutionA circle having centre as O' and radius r' touches the incircle of ΔABC externally at. F, where F is on BC and also touches its circumcircle internally at G. It O is the circumcentre of ΔABC and I is its incentre, then
05:38View SolutionThe radius of the circle touching two sides AB and AC of a triangle ABC and having its center on BC is equal to………………..
05:18View SolutionVertex A of a variable triangle ABC, inscribed in a circle of radius R, is a fixed point. If the angles subtended by the side BC at orthocentre (H), circumcentre (O) and incentre (I) are equal than identify the locus of orthocentre of triangle ABC.
04:23View SolutionIn ΔABC,∠A=π3 and its incircle of unit radius. Find the radius of the circle touching the sides AB, AC internally and the incircle of ΔABC externally is x , then the value of x is
03:01View SolutionA circle with centre 'O' is incircle of △ABC.∠ACB=90∘. Radius of the circle is r. Prove that: 2r=a+b−c.
 01:39View Solution
01:39View Solution

