Two circles C1andC2 both pass through the points A(1,2)andE(2,1) and touch the line 4x−2y=9 at BandD, respectively. The possible coordinates of a point C, such that the quadrilateral ABCD is a parallelogram, are (a,b). Then the value of |ab| is_________Solution in Tamil
The correct Answer is:4
The radical axis bisects the common tangents BD.
Hence, M is the midpoint of BD.
Let C≡(a,b)
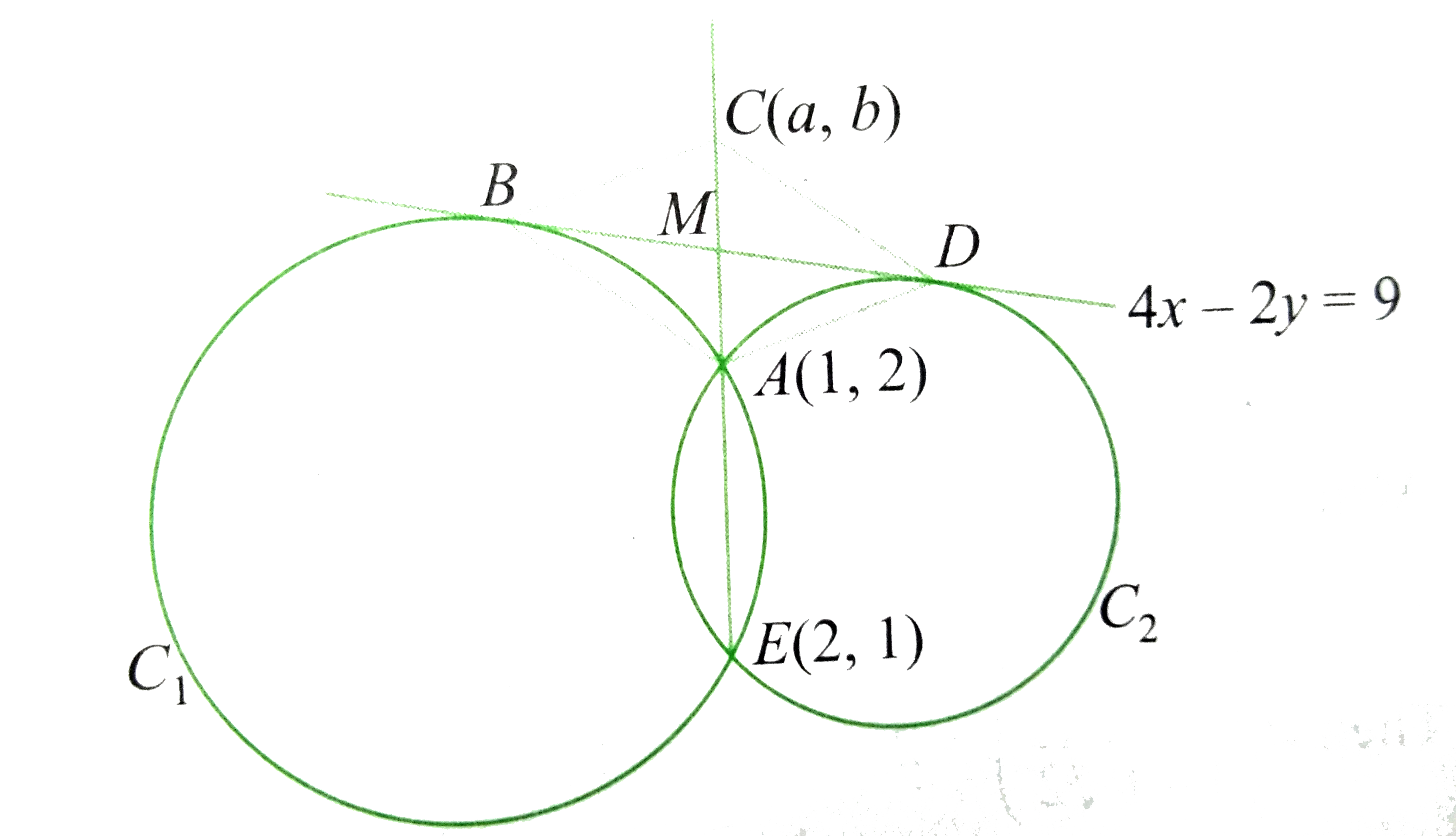
Now, C(a,b) lies on common chord AE which is y−2=−1(x−1) or x+y=3. Therefore,
a+b=3 (1)
Also, M(a+12,b+22) lies on 4x−2y=9. Therefore,
4(a+12)−2(b+22)=9
or 2a+2−b−2=9
or 2a−b=9 (2)
Solving (1) and (2) , a=4 and b=−1. Therefore,
∴|ab|=4
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Main Previous Year
10 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Advanced (Single Correct Answer Type)
14 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Matrix)
1 Videos
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
16 Videos
Similar Questions
If P and Q are the points of intersection of the circles x2+y2+3x+7y+2p=0 and x2+y2+2x+2y−p2=0 then there is a circle passing through P,Q and (1,1) for
View SolutionTangents drawn from the point P(1,8) to the circle x2+y2−6x−4y−11=0 touch the circle at the points A&B ifR is the radius of circum circle of triangle PAB then [R]-
View SolutionLet C1 and C2 be two circles whose equations are x2+y2−2x=0 and x2+y2+2x=0 and P(λ,λ) is a variable point
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionABCD is a cyclic quadrilateral. ABandDC are produced to meet in E. Prove that EBC−EDA.
View SolutionTwo circles C1andC2 intersect at two distinct points PandQ in a line passing through P meets circles C1andC2 at AandB , respectively. Let Y be the midpoint of AB,andQY meets circles C1andC2 at XandZ , respectively. Then prove that Y is the midpoint of XZ.
View SolutionTwo circles C1andC2 both pass through the points A(1,2)andE(2,1) and touch the line 4x−2y=9 at BandD, respectively. The possible coordinates of a point C, such that the quadrilateral ABCD is a parallelogram, are (a,b). Then the value of |ab| is_________
View SolutionTwo circles passing through A(1,2),B(2,1) touch the line 4x+8y-7=0 at C and D such that ACED in a parallelogram.Then:
View Solutionp AND q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar(APB)=ar (BQC).
View SolutionTwo circles C1andC2 both pass through the points A(1,2)andE(2,1) and touch the line 4x−2y=9 at BandD, respectively. The possible coordinates of a point C, such that the quadrilateral ABCD is a parallelogram, are (a,b). Then the value of |ab| is_________
View SolutionTwo circles C1andC2 intersect at two distinct points PandQ in a line passing through P meets circles C1andC2 at AandB , respectively. Let Y be the midpoint of AB,andQY meets circles C1andC2 at XandZ , respectively. Then prove that Y is the midpoint of XZ.
View SolutionTwo circles passing through A(1,2),B(2,1) touch the line 4x+8y−7=0 at C and D such that ACED in a parallelogram. Then:
View SolutionABCD is a parallelogram. If LandM are the mid-points of BCandDC respectively, then express →ALand→AM in terms of →ABand→AD . Also, prove that →AL+→AM=32→AC.
View SolutionIn an isosceles triangle ABC with AB=AC, a circle passing through B and C intersects the sides ABandAC atDandE respectively. Prove that DE∣∣BC.
View SolutionABC is an isosceles triangle in which AB=AC. If DandE are the mid-points of ABandAC respectively, prove that the points B,C,DandE are concylic.
View Solution
