Question

about to only mathematics

Explanation

Here, for the first circle, the center is `C_(1)(-3,1)` and radius `r_(1)` is 1.
For the second circle, the center is `C_(2)(-3,1)` and radius `r_(2)` is 3.
Thus, `C_(1)C_(2)=2sqrt(5)` and `r_(1)+r_(2)=4` or `C_(1)C_(2) gt r_(1)+r_(2),r_(1) cancel(=)r_(2)`. Thus,we have four common tangents.

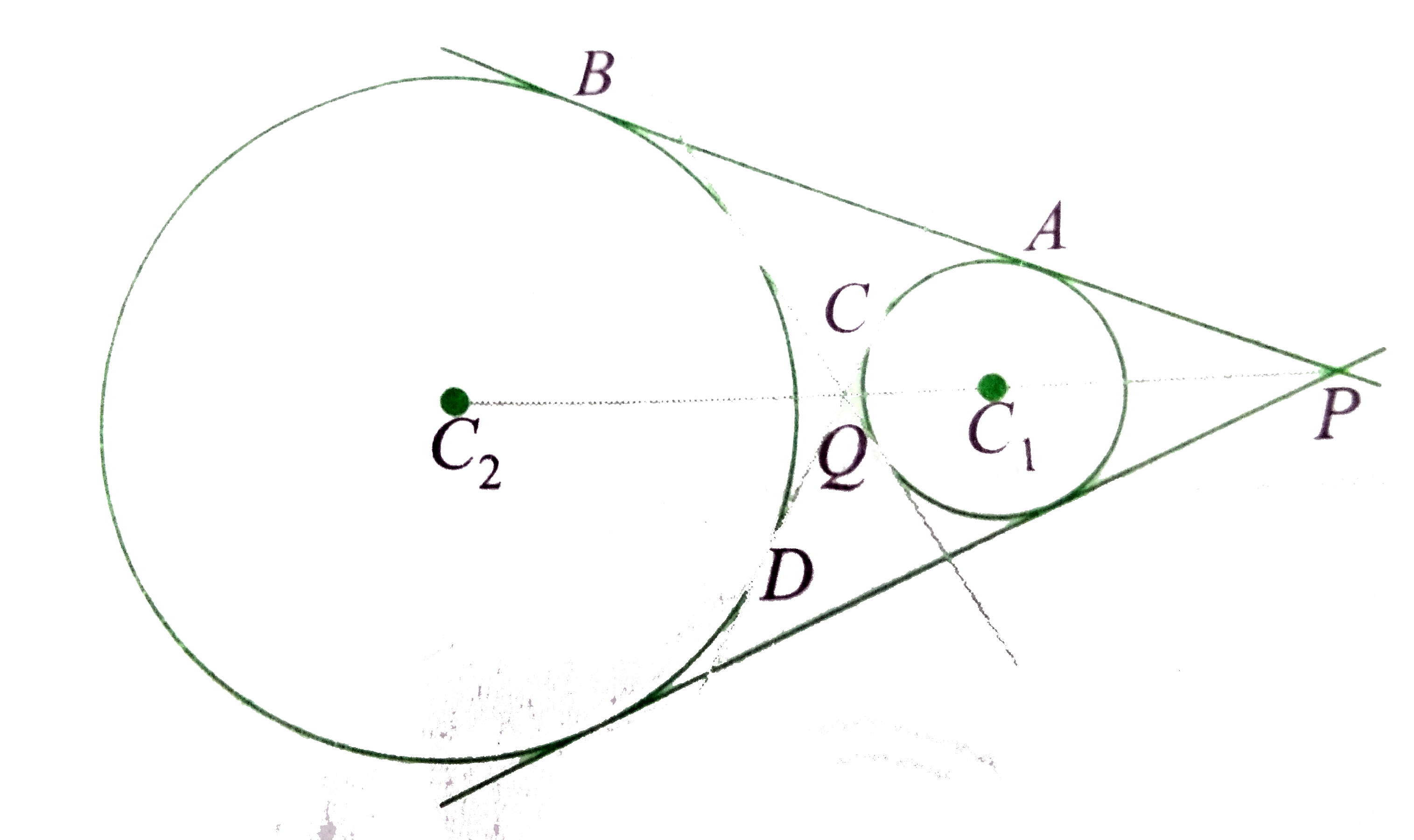
To find direct common tangents `:`
The coordinates of the point P dividing line `C_(1)C_(2)` in the ratio `r_(1) : r_(2), i. e., 1:3`, externally, are
`((1xx(-3)-3xx1)/(1-3),(1xx1-3 xx 3)/(1-3))` or `(3,4)`
Therefore, the equation of any line through point P(3,4) is
`y-4=m_(1)(x-3) `
or `m_(1)x-y+4-3m_(1)=0` (1)
If (1) is tangent to the first circle, then the length of perpendicular from the center `C_(1)(1,3)` of (1) is equal to `r_(1)` (radius) . Therefore, `(|m_(1)-3+4-3m_(1)|)/(sqrt((m_(1)^(2)+1)))=1` or `(1-2m_(1))^(2)=m_(1)^(2)+1`
or` 3m_(1)^(2)-4m_(1)=0`
or `m_(1)=0,(4)/(3)`
Substituting `m_(1)=0` and `4//3` in (1), the equations of direct common tangents are y=4 and `4x-3y=0`.
To find transverse common tangents `:`
The coordinates of the point Q dividing the line `C_(1)C_(2)` in the ratio `r_(1) : r_(2), i.e., 1:3`, internally , are `(0,5//2)`.
Therefore, the equation of any line through `Q(0,5//2)` is
`y-(5)/(2)=m_(2)(x-0)` or `m_(2)x-y+(5)/(2)=0` (2)
If (2) is tanent to the first circle, then the length of perpendicular from the center `C_(1)(1,3)` on (2) is equal to `r_(1)` (radius) . Therefore, `(|m_(2)-3+5//2|)/(sqrt((m_(11)^(2)+1)))=1` ltbr. or `(2m_(2)-1)^(2)=4(m_(2)^(2)+1)`
or `-4m_(2)-3=0`
`:. m_(2)=-(3)/(4)` and `oo` (A coefficient of `m_(2)^(2)` is zero )
Substituting `m_(2)= - 3//4` and `oo` in (2), the equatinos of transverse comon tangents are ,brgt `3x+4y-10=0` and `x=0`
`AB^(2)=C_(1)C_(2)^(2)-(r_(1)-r_(2))^(2)`
`=20-(3-1)^(2)=16`
`:. AB=4`
`CD^(2)=C_(1)C_(2)^(2)-(r_(1)+r_(2))^(2)`
`=20-(3+1)^(2)=4`
`:. CD =2`