Find the area of the region in which the points satisfy the inequaties 4<x2+y2<16 and 3x2−y2>0Solution in Tamil
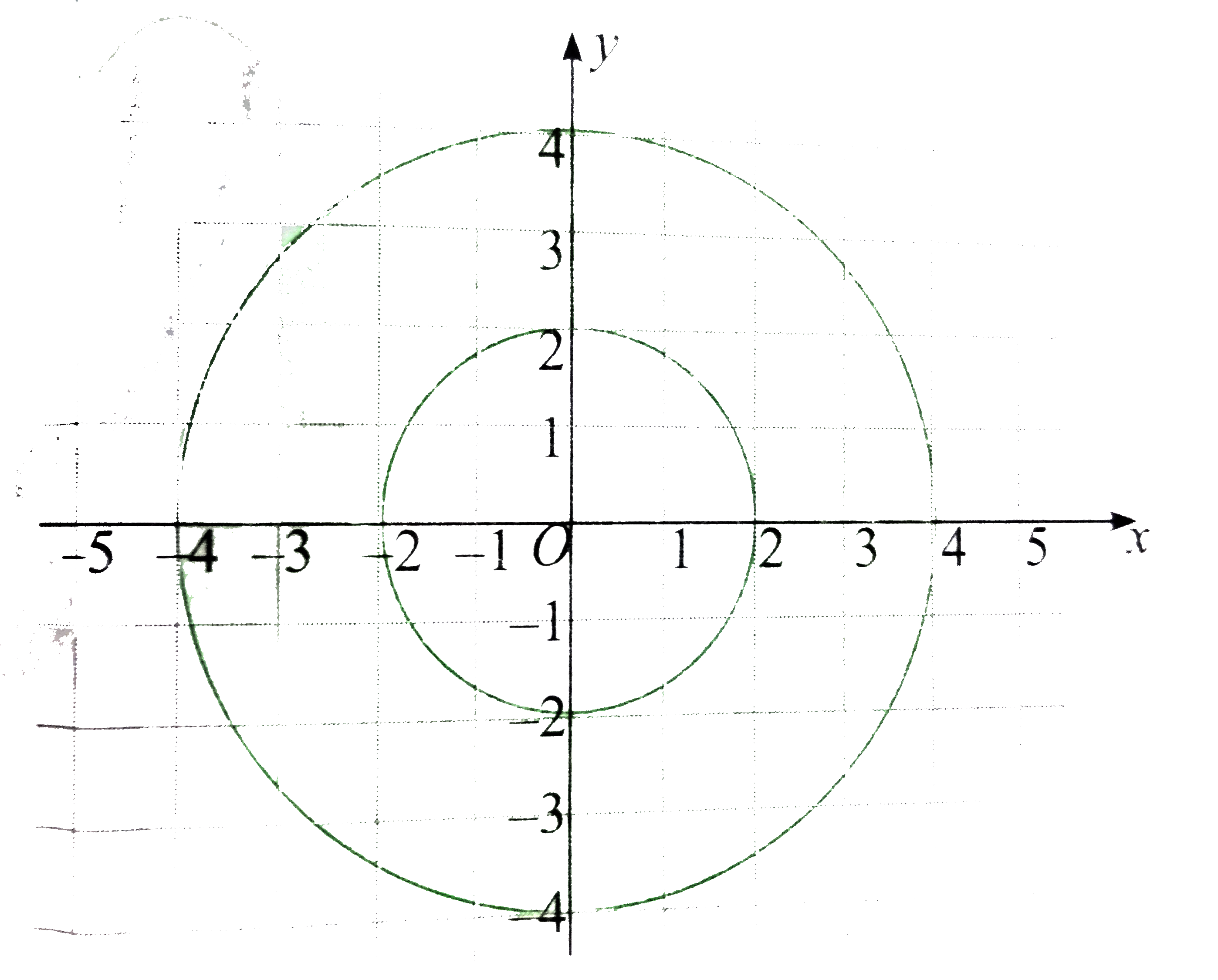
The points satisfying 4<x2+y2<16 lie in the region outside the circle x2+y2=4 and inside the circle x2+y2=16 as shown in the following figure.
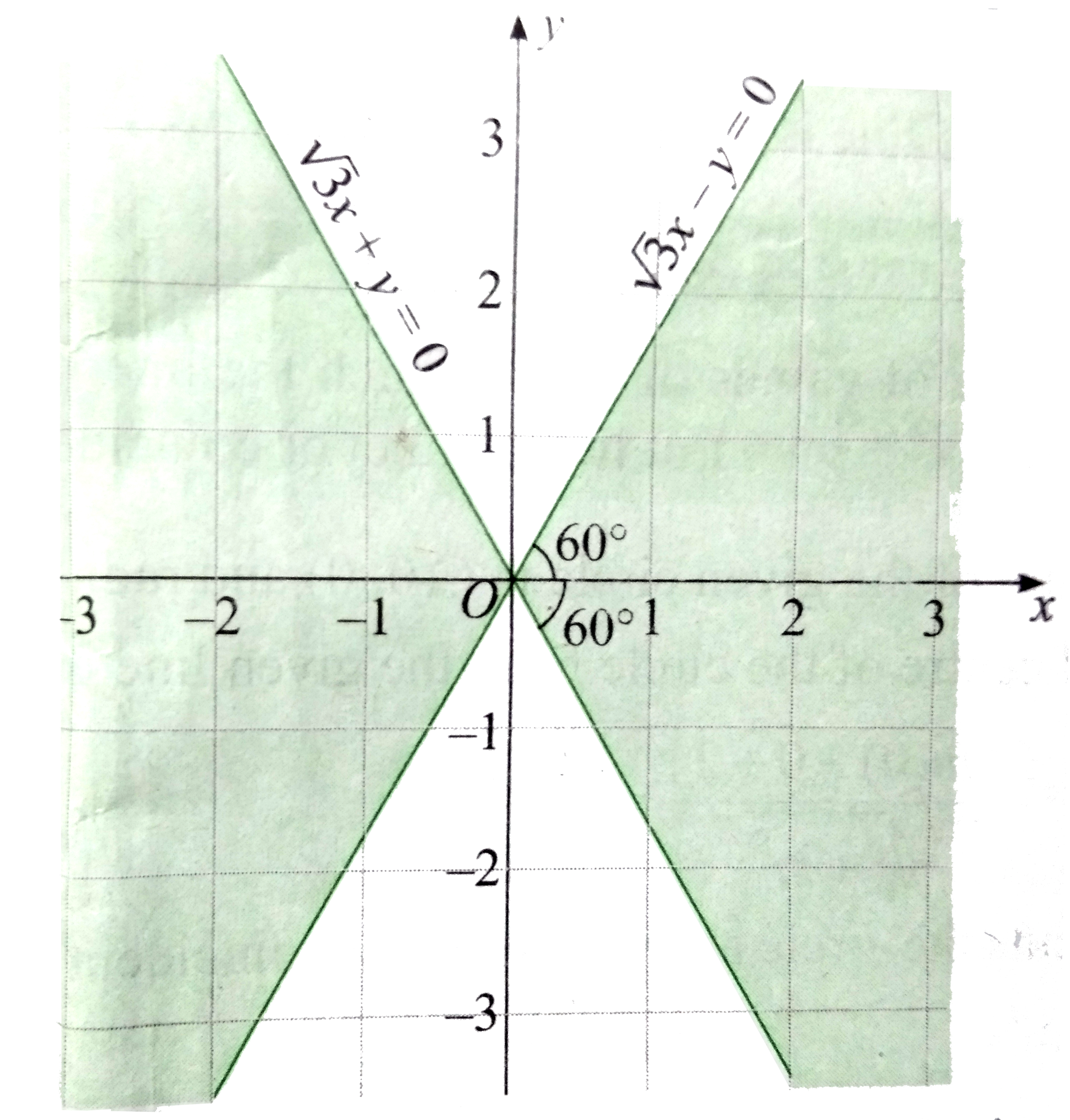
Now, 3x2−y2>0
∴(√3x−y)(√3x+y)>0
Now, draw the lines √3x−y=0 and √3x+y=0.
Therefore, 3x2−y2>0 represents the following region :
Hence, the region of the points satisfying both the inequalities is shown in the following figure.
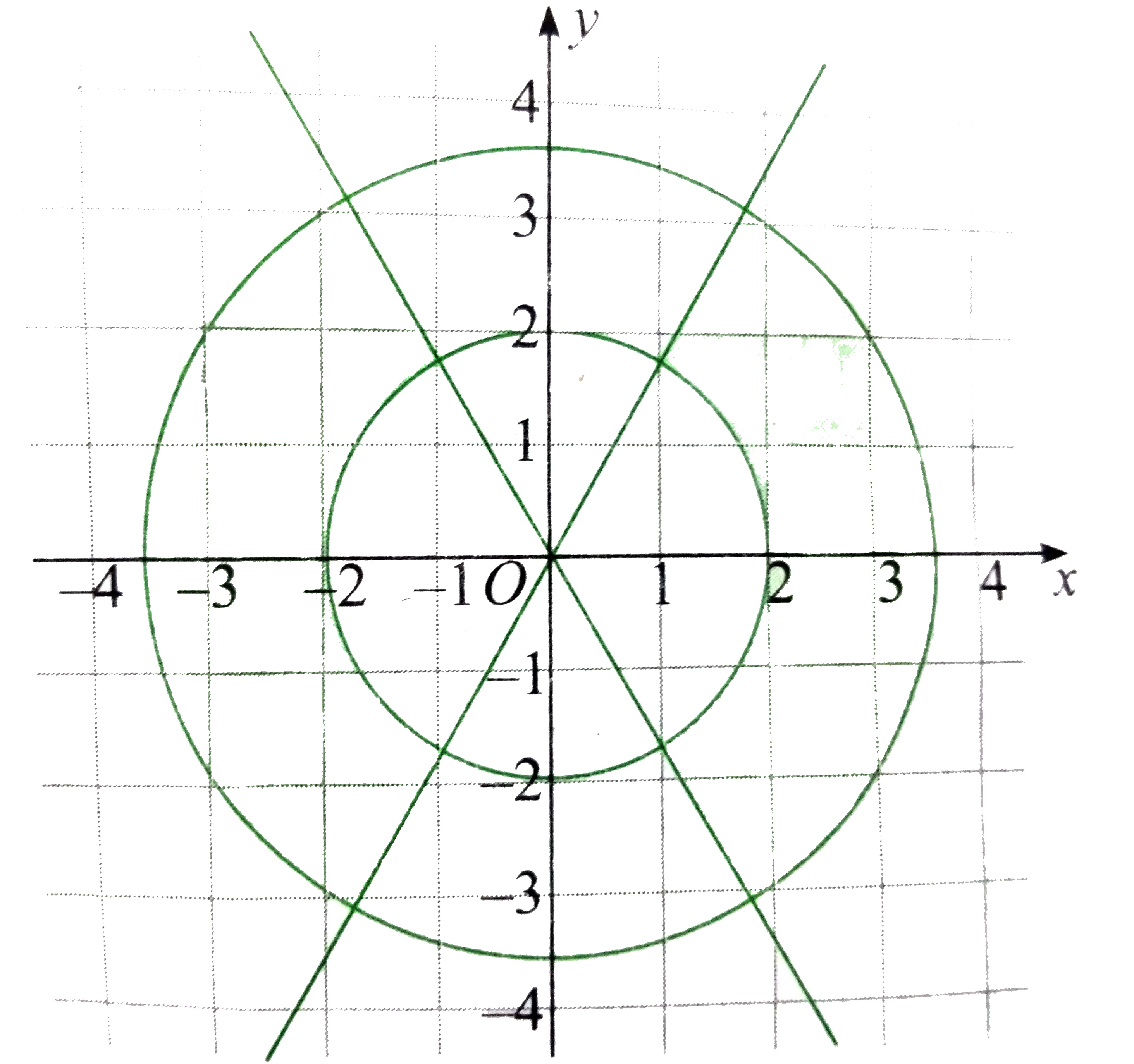
Hence, the required area is
46[π(4)2−π(2)2]=8π
Topper's Solved these Questions
Similar Questions
If a circle whose center is (1,−3) touches the line 3x−4y−5=0 , then find its radius.
View SolutionFind the radius of the circle (x−5)(x−1)+(y−7)(y−4)=0 .
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionFind the area of the region in which the points satisfy the inequaties 4<x2+y2<16 and 3x2−y2≥0.
View SolutionFind area of region bounded by y=x^(2)-3x+2,x=1,x=2 and y=0
View SolutionThe area of the region containing the points (x, y) satisfy-ing 4<=x^(2)+y^(2)<=2(|x|+|y|) is
View SolutionFind tha area of the region containing the points (x,y) satisfying 4≤x2+y2≤2(|x|+|y|).
View SolutionFind the area of the region lying between the parabolas 4y2=9xand3x2=16y.
View SolutionFind the area of the region in which the points satisfy the inequaties 4<x2+y2<16 and 3x2−y2≥0.
View SolutionFind tha area of the region containing the points (x,y) satisfying 4≤x2+y2≤2(|x|+|y|).
View SolutionArea of region enclosed by the region y2≤3x,x2+y2≤4 and y≥0 is :
View SolutionDraw the rough sketch and find the area of the region : {(x,y):16x2+y2≤16,4x+y≥4}.
View SolutionFind the area of the region enclosed by the curves
y=x2andy=3x
View SolutionFind the area of the region bounded by the circles x2+y2=16 and (x+4)2+y2=16
View SolutionFind area of region bounded by y=x2–3x+2,x=1,x=2 and y=0.
View Solution
