The area bounded by the curve x=acos3t,,y=asin3t, is :Solution in Tamil
12a2π/2∫0cos4tsin2tdt
12a2π/2∫0cos2tsin4tdt
2a∫−a(a2/3−x2/3)3/2dx
4a∫0(a2/3−x2/3)dx
The correct Answer is:A, C, D
Eliminating t, we have x23+y23=a23⇒y=(a2/3−x2/3)3/2.
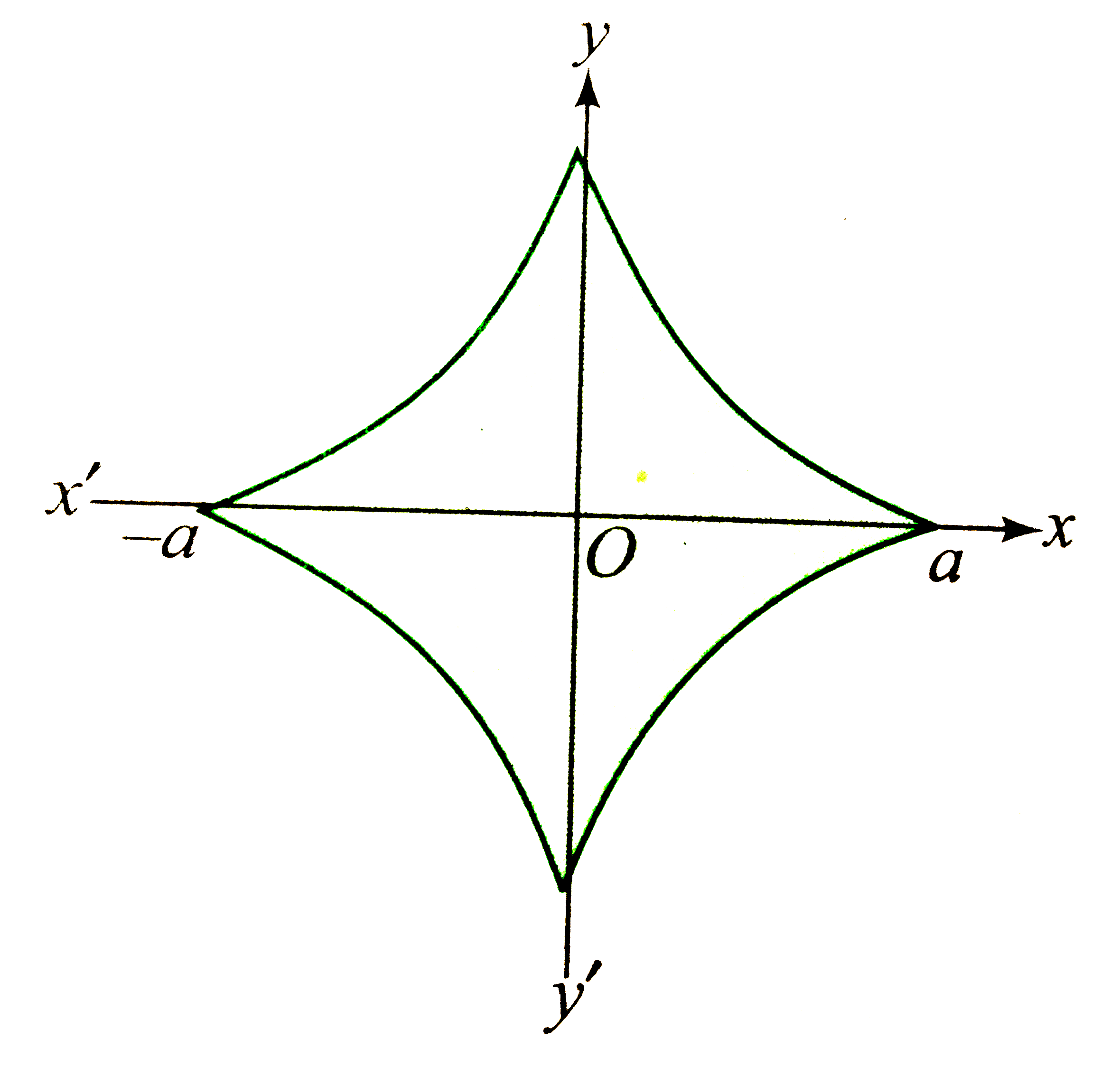
From the figure,
A=2a∫−a(a2/3−x2/3)dx
=4a∫0(a2/3−x2/3)3/2dx=4a∫0ydx
=4a2π/2∫03cos3tsin2tcostdt.
Topper's Solved these Questions
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Comprehension)
21 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Matrix)
4 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Single)
40 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type
2 Videos
- AREA UNDER CURVESBOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
10 Videos
Similar Questions
Let Ar be the area of the region bounded between the curves y2=(e−kr)x(where k>0,r∈N) and the line y=mx(where m≠0), k and m are some constants
A1,A2,A3,… are in G.P. with common ratio
View SolutionMatch the following lists :

View SolutionArea enclosed by the curve y=f(x) defined parametrically as x=1−t21+t2,y=2t1+t2isequa<o πsq.units (b) π2sq.units 3π4sq.units (d) 3π2sq.units
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionThe area of the region (s) enclosed by the curves y=x2 and y=√|x| is
View SolutionFind dydx , if x=sin3t√cos2t , y=cos3t√cos2t
View SolutionFind the equations of the tangent and the normal at the point 't' on the curve x=a s∈3t , y=b cos3t .
View Solutionx=3sint−2sin3t,y=3cost−2cos3tthen find dydx
View Solutionबिंदु t पर वक्र x=cos3t;y=bsin3t के स्पर्शी का समीकरण ज्ञात कीजिए |
View Solutionबिंदु t पर वक्र x=acos3t,y=bsin3t की स्पर्श रेखा का समीकरण ज्ञात कीजिए।
View SolutionIf x=esin3t,y=ecos,3t,thendydx=
View SolutionIf x=esin3ty=ecos,3t,thendydx=
View SolutionShow that the equation of normal at any point t on the curve x=3cost−cos3t and y=3sint−sin3t is 4(ycos3t−xsin3t)=3sin4t.
View SolutionThe area bounded by the curve x=acos3t,,y=asin3t, is :
View SolutionFind the equations of the tangent and the normal at the point 't' on the curve x=a sin3t , y=b cos3t .
View Solution
