Find the area of the region bounded by the x-axis and the curves defined by ,
(where ) and .(where )Solution in Tamil
The correct Answer is:sq. units
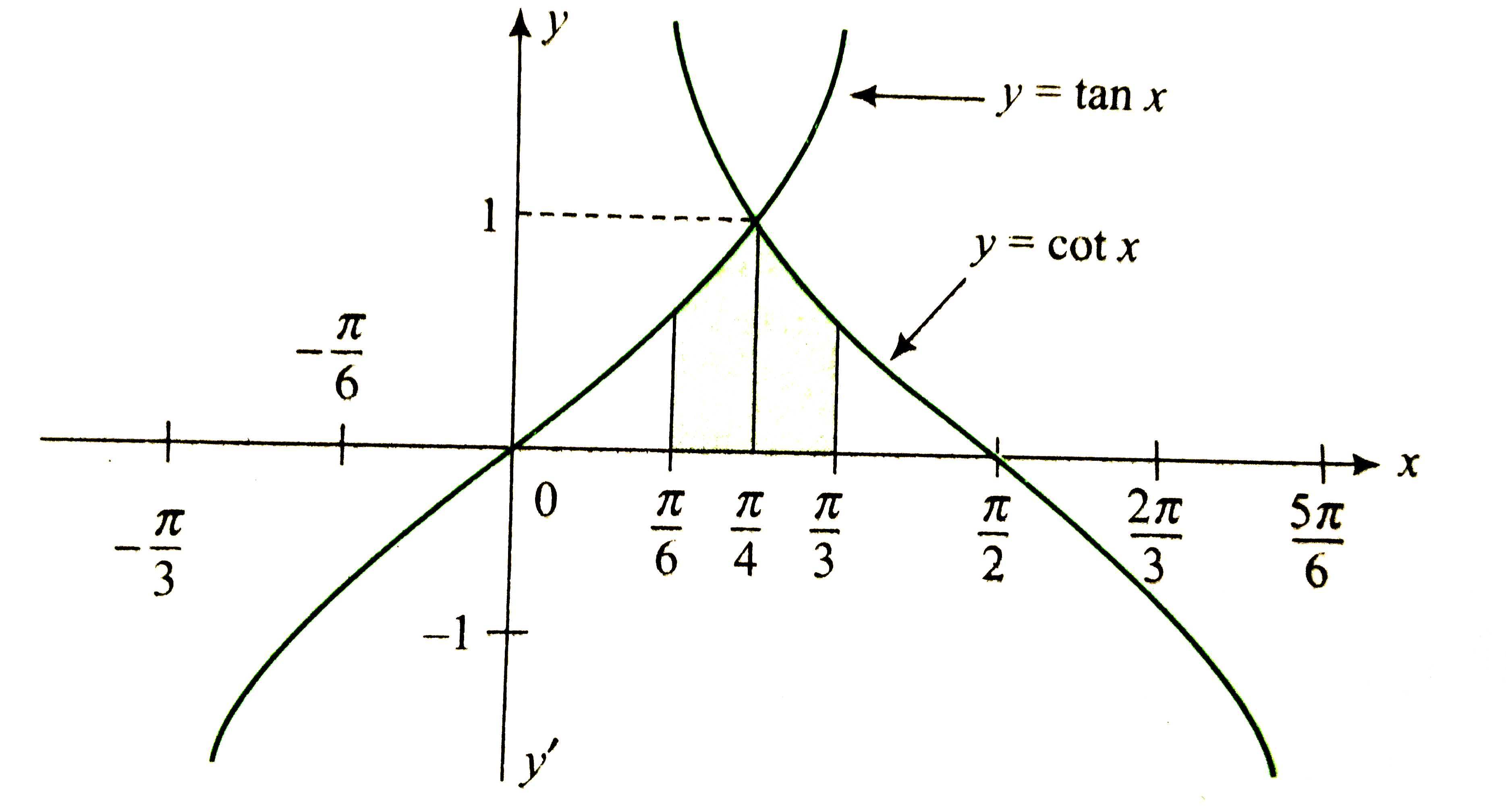
The two curves are
At the point of intersection of the two curves
Thus, the curves interset at
The required area is the shaded area. Therefore,
sq. units.
Topper's Solved these Questions
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.2
14 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.3
7 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Comprehension Type
2 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type
2 Videos
- AREA UNDER CURVESBOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
10 Videos
Similar Questions
Find the area lying in the first quadrant and bounded by the curve y=x3 and the line y=4x.
View SolutionFind the continuous function f where (x4−4x2)≤f(x)≤(2x2−x3) such that the area bounded by y=f(x),y=x4−4x2. then y-axis, and the line x=t, where (0≤t≤2) is k times the area bounded by y=f(x),y=2x2−x3,y−aξs, and line x=t(where0≤t≤2).
View SolutionIn the following figure, the graphs of two functions y = f(x) and y = sin x are givne. They intersect at origin, A(a,f(a)),B(π,0)andC(2π,0).Ai(i=1,2,3) is the area bounded by the curves as shown in the figure, respectively, for x∈(0,a),x∈(a,π),x∈(π,2π).
If A1=1+(a−1)cosa−sin a, then

The function f(x) is
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionThe area of the region (s) enclosed by the curves y=x2 and y=√|x| is
View SolutionFind the area of the region bounded by the x-axis and the curves defined by y=tanx,−π3≤x≤π3 and y=cotx,π6≤x≤3π2
View SolutionFind the area of the region bounded by the x-axis and the curves defined by y=tanx,
(where −π3≤x≤π3) and y=cotx.(where π6≤x≤2π3)
View SolutionThe area of the region bounded by the X-axis and the curves defined by y=tan x,
(−π3≤x≤π3) is
View Solutionवक्र y=cosx,(0≤x≤π) तथा x -अक्ष से घिरे क्षेत्र का क्षेत्रफल ज्ञात कीजिए ।
View SolutionFind the area bounded by y = sin x, 0≤x≤π4,y=cosx,π4≤x≤π2 and the x-axis
View Solutionवह क्षेत्रफल ज्ञात कीजिए , जो x-अक्ष तथा y=tanx,−π3≤x≤π/3 तथा y=cotx,π6≤x≤π/2 द्वारा परिभाषित वक्रों द्वारा घिरा हुआ है।
View Solutionवक्र y= tanx तथा x-अक्ष से घिरे क्षेत्र का क्षेत्रफल (जहाँ - π/3≤x≤π/3) होगा
View SolutionThe area bounded by the curves y=tanx,−π3≤x≤π3,y=cotx,π6≤x≤π2 and the x - axis is
View SolutionThe area between the curves y=tanx,y=cotx and X-axis in π6≤x≤π3 is
View SolutionThe area of the region bounded by the x-axis and the curves y=tanx(−π3≤x≤π3) and y=cotx(π6≤x≤3π2) is
View SolutionThe area of the region above x-axis bounded by y=cotx,π12≤x≤π4 is
View Solution
