Question

Find the equivalent definition of
$f\left(x\right)=max\stackrel{.}{x^2,{(-x)}^2,2x(1-x)}whre0\le x\le 1$

Answer

`f(x)={((1-x)^(2)",",0le x lt 1//3),(2x(1-x)",",1//3 le x lt 2//3),(x^(2)",",2//3 le x le 1):}`

Explanation

Here, for maximum, let us consider `f_(1)(x)=x^(2),f_(2)(x)=(1-x)^(2), " and " f_(3)(x)=2x(1-x)`.
Now graph for `f_(1)(x),f_(2)(x), " and " f_(3)(x)` are as follows.

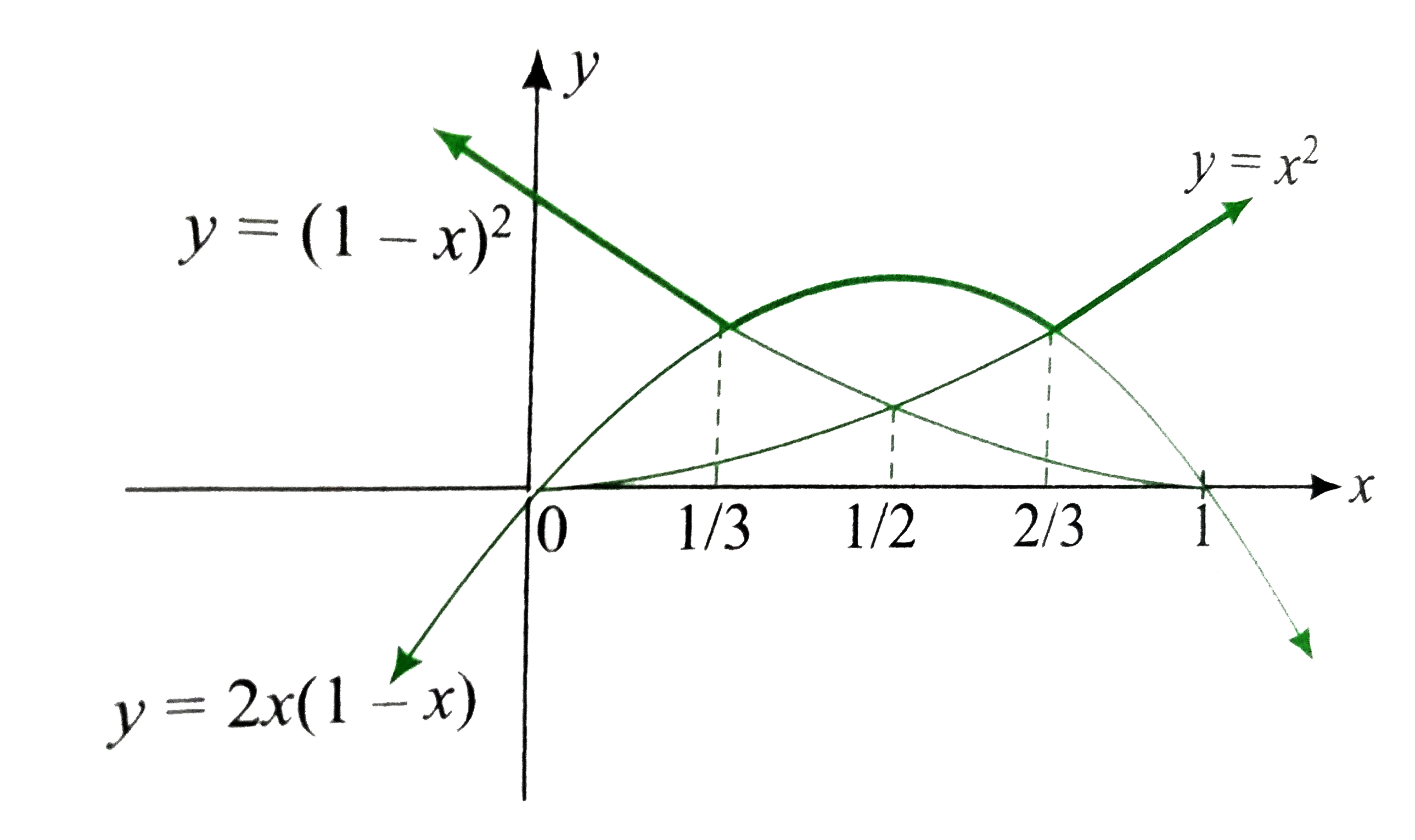
Here, neglect the graph that is below the points of intersection.
`f(x)={((1-x)^(2)",",0le x lt (1)/(3)),(2x(1-x)",",(1)/(3) le x lt (2)/(3)),(x^(2)",",(2)/(3) le x le 1):}`