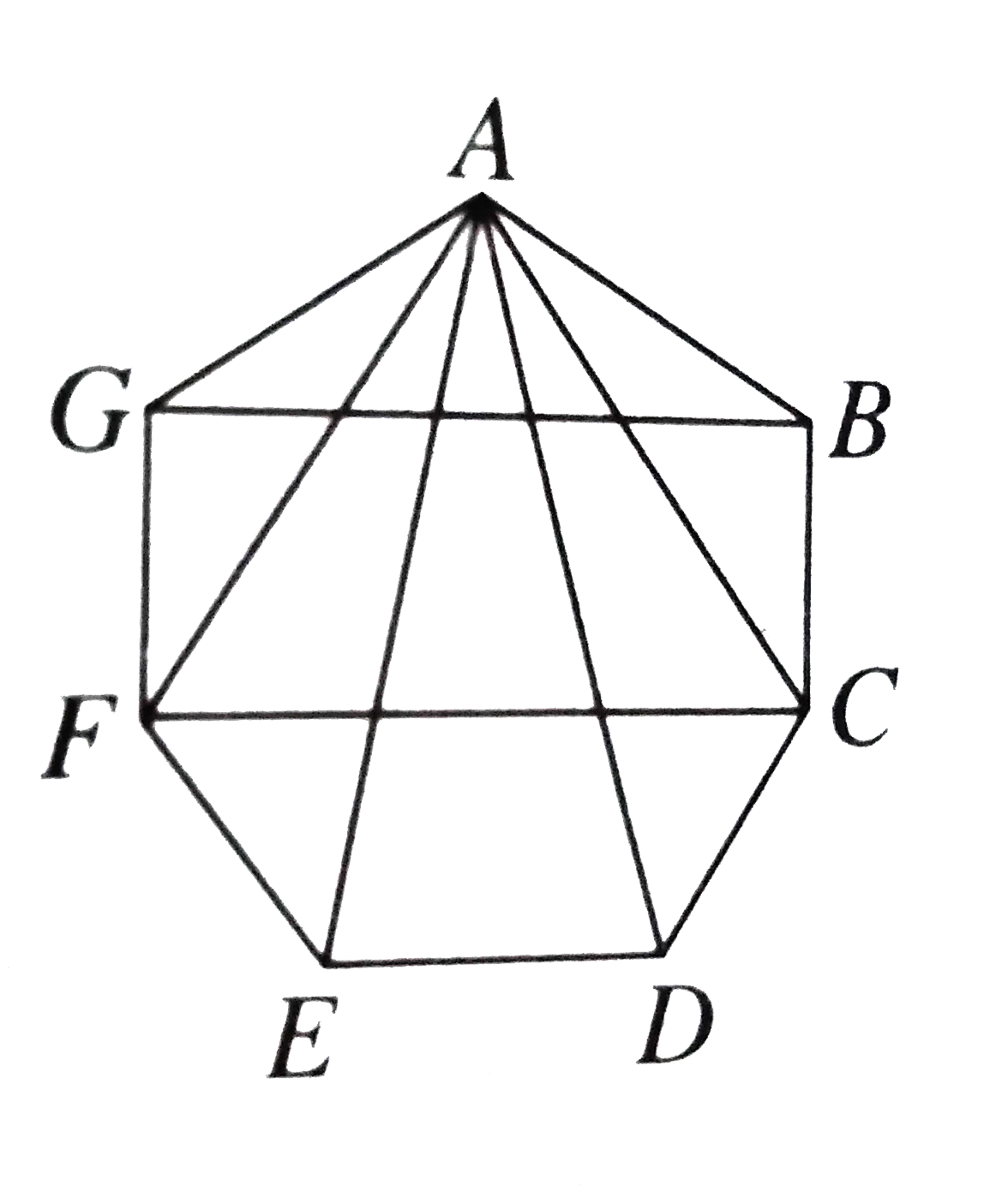
Three vertices are chosen randomly from the seven vertices of a regular 7-sided polygon. The probability that they form the vertices of an isosceles triangle isSolution in Tamil
17
13
37
35
The correct Answer is:D
(d) ΔAGB, ΔAFC and ΔAED are isosceles
∴P=7C1×3'7C3=7×37×6×53×2=35
Topper's Solved these Questions
- PROBABILITYBOOK - CENGAGECHAPTER - PROBABILITYEXERCISE - Comprehension
2 Videos
- PROBABILITYBOOK - CENGAGECHAPTER - PROBABILITYEXERCISE - Multiple Correct Answer
13 Videos
- PRINCIPLE OF MATHEMATICAL INDUCTIONBOOK - CENGAGECHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - Exercise
9 Videos
- PROBABILITY AND STATISTICSBOOK - CENGAGECHAPTER - PROBABILITY AND STATISTICSEXERCISE - Question Bank
24 Videos
Similar Questions
A slip of paper is given to a person A who marks it either with a plus sign or a minus sign. The probability of his writing a plus sign is 1/3. A passes the slip to B, who may either leave it alone or change the sign before passing it to C. Next C passes the slip to D after perhaps changing the sign. Finally D passes it to a refere after perhaps changing the sign. B,C,Deachchan≥thesignwithprobability2//3.<br>TheprobabilitytˆtherefereeobservesaplussignontheslipifitisknowntˆA wrote a plus sign is
View SolutionThe probablities of events , A∩B, A, B and A∪B are respectively in A.P. with second term equal to the common difference. Therefore A and B are
View SolutionProve that by using the principle of mathematical induction for all n∈N:
1.3+3.5+5.7+....+(2n−1)(2n+1)=n(4n2+6n−1)3
View SolutionIf two events A and B are such that P(Al)=0.3, P(B)=0.4 and P(A∩Bl)=0.5, then P(BA∪Bl) equals
View SolutionThree distinct vertices are chosen at random from the vertices of a given regular polygon of (2n + 1) sides Let all such choices are equally likely and the probability that the centre of the given polygon lies in the interior of the triangle determined by these three chosen random points is 5/14 Three vertices of the polygon are chosen at random. The probability that these vertices form an isosceles triangle is.
View SolutionThree vertices are chosen from the vertices of a regular hexagon, then the probability that they will form an equilateral triangle is
View SolutionThree vertices are chosen randomly from the seven vertices of a regular 7-sided polygon. The probability that they from the vertices of an isosceles triangle is-
View SolutionThree vertices are chosen randomly from the seven vertices of a regular 7-sided polygon. The probability that they form the vertices of an isosceles triangle is
View SolutionThree vertices are chosen at random from the vertices of a regular hexagon then the probability that the triangle with these vertices is an equilateral triangle is equal to
View SolutionThree vertices are chosen at random from the vertices of a regular hexagon then
The probability that the triangle has exactly one side common with the side of the hexagon is
View SolutionThree vertices are chosen at random from the vertices of a regular hexagon then
The probability that the triangle has exactly two sides common with the side of the hexagon is
View Solution3 out of 6 vertices of a regular hexagon are chosen at a time at random. The probability that the triangle formed with these three vertices is an equilateral triangle , is
View Solutionकिसी समषटभुज के 6 शीर्षों में 3 शीर्षों को यदृच्छया चुना गया है। इन तीन शीर्षों से बनने वाले त्रिभुज समबाहु हो, तो इसकी प्रायिकता है
View Solutionयदि एक सम षट्भुज के छः शीर्षो में से तीन यादृश्चिक चुने जाते है, तो इन चुने गए शीर्षो द्वारा बने त्रिभुज के समबहु होने की प्रायिकता है
View SolutionThree distinct vertices are randomly chosen among the vertices of a cube. The probability that they from equilateral triangle is
View Solutionएक समषट्भुज के छ: शीर्षों में से तीन शीर्षों को यादृच्छिक रूप से चुना जाता है। इन तीन शीर्ष से बने त्रिभुज का समबाहु होने की प्रायिकता है
View Solution
