Draw the graph of f(x)=secx+cosec x,x∈(0,2π)−{π/2,π,3π/2}
Also find the values of 'a' for which the equation secx+cosec x=a has two distinct root and four distinct roots.Solution in Tamil
We have f(x)=secx+cosec x,x∈(0,2π)−{π/2,π,3π/2}
Consider each of the intervals (0,π/2),(π/2,π),(π,3π/2),(3π/2,2π)
For x∈(0,π/2)
When x→0+orx→π/2−,secx+cosec x→∞
Also in the 1st quadrant, sec x + cosec x gt 0
f'(x)=secxtanx−cosec xcotx
f'(π/4)=0, so x=π/4 is clearly a point of minima.
f(π/4)=2√2
For x∈(π/2,π)
When x→π/2+,secx+cosec x→−∞
When x→π−,secx+cosec x→∞
Also f'(x)+secxtanx−cosec xcotx>0
Hence f(x) is an increasing function.
f(3π/4)=0
For x∈(π,3π/2)
When x→π+,secx+cosec x→−∞
When x→3π/2−,secx+cosec x→−∞
f'(5π/4)=0, so x=5π/4 is clearly a point of maxima.
f(5π/4)=−2√2
For x∈(3π/2,2π)
When, x→3π/2+,secx+cosec x→∞
When x→2π−,secx+cosec x→−∞
Also f'(x)+secxtanx−cosec xcotx<0
Hence f(x) is a decreasing function.
f(7π/4)=0
From the above discussion, the graph of the function is as shown in the following figure. 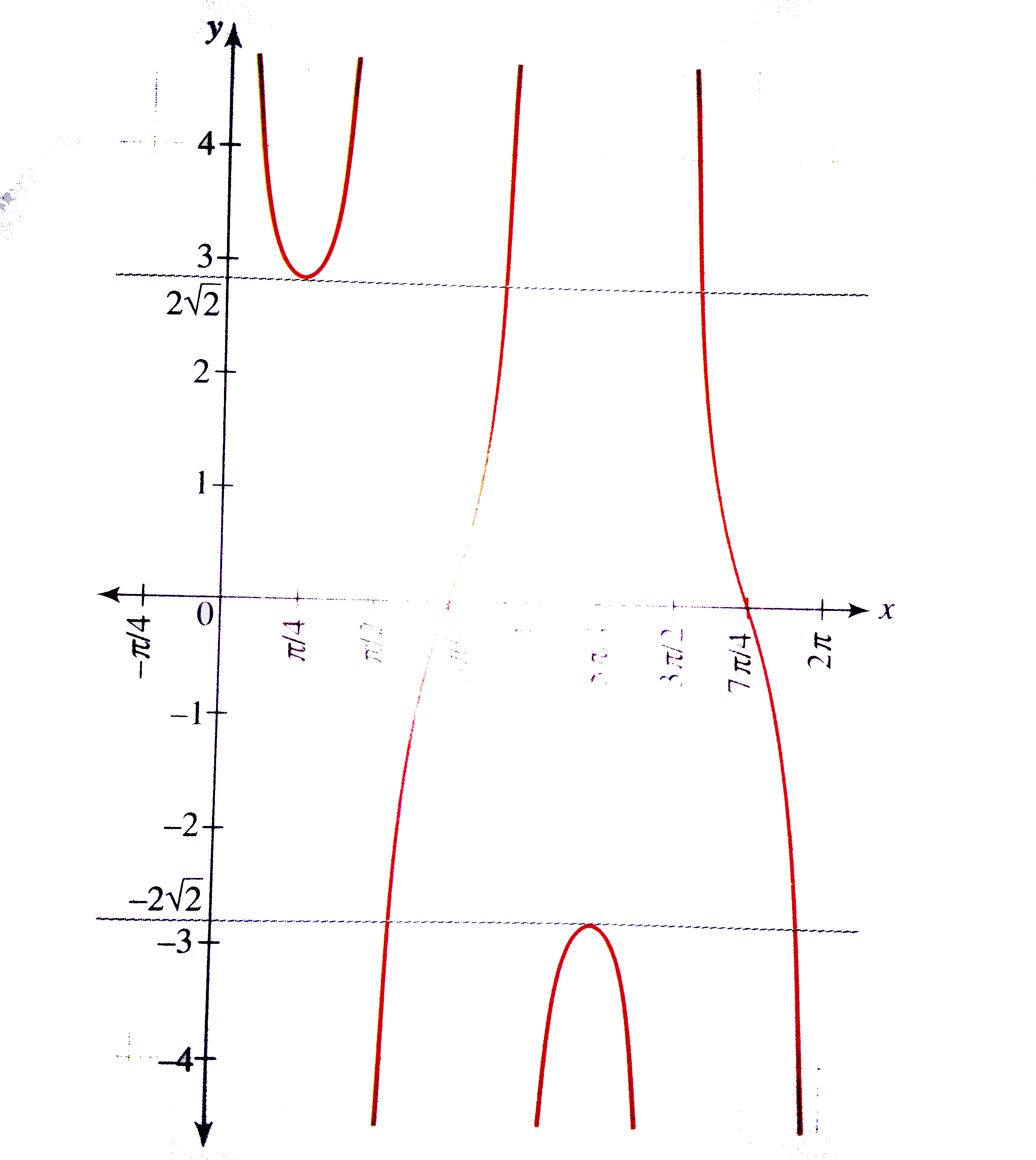
Topper's Solved these Questions
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
- HIGHT AND DISTANCE BOOK - CENGAGECHAPTER - HIGHT AND DISTANCE EXERCISE - JEE Previous Year
3 Videos
Similar Questions
Draw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
View SolutionDraw the graph of y=1(1/x).
View SolutionA bird is sitting on the top of a vertical pole 20 m high and its elevation from a point O on the ground is 45o . It flies off horizontally straight away from the point O. After one second, the elevation of the bird from O is reduced to 30o . Then the speed (in m/s) of the bird is (1) 40(√2−1) (2) 40(√3−2) (3) 20√2 (4) 20(√3−1)
View SolutionIf lim_(x rarr0)x^(2)cos(pi sec^(2)x)csc(pi sec^(2)x)=(1)/(a pi) then a is equal to
View SolutionDraw the graph of f(x)=secx+cosec x,x∈(0,2π)−{π/2,π,3π/2}
Also find the values of 'a' for which the equation secx+cosec x=a has two distinct root and four distinct roots.
View SolutionConsider the equation
secθ+cosecθ=a,θ∈(0,2π)−{π/2,π,3π/2}
If the equation has four distinct real roots, then
View SolutionConsider the equation
secθ+cosecθ=a,θ∈(0,2π)−{π/2,π,3π/2}
If the equation has two distinct real roots, then
View SolutionConsider the equation
secθ+cosecθ=a,θ∈(0,2π)−{π/2,π,3π/2}
If the equation has no real roots, then
View Solution∫π/20f(secx)f(secx)+f(cosecx)dx=
View Solution∫π/203Secx+5cosecxSecx+cosecxdx=
View SolutionLet f(x)=2cosec2x+secx+cosecx, then the minimum value of f(x) for x∈(0,π2) is
View SolutionDraw the graph of f(x)=secx+cosec x,x∈(0,2π)−{π/2,π,3π/2}
Also find the values of 'a' for which the equation secx+cosec x=a has two distinct root and four distinct roots.
View SolutionConsider the equation
secθ+cosecθ=a,θ∈(0,2π)−{π/2,π,3π/2}
If the equation has no real roots, then
View Solution∫π204 sec x + 5 cosec xsec x + cosec xdx
View Solutionमाना f(x)=2 cosec 2x+secx+cosec x है, तो x∈(0,π2) के लिए f(x) का न्यूनतम मान है
View Solution
