Find the shortest distance between the lines whose vector equations are→r=(4ˆi−ˆj)+λ(ˆi+2ˆj−3ˆk) and →r=(ˆi−ˆj+2ˆk)+μ(ˆi+4ˆj−5ˆk)Solution in Tamil
The correct Answer is:a→qs;b→p;c→s;d→r
The given lines is x=4y+5,z=3y−6
or x−54=y,z+63=y
or x−54=y1=z+63=λ (say)
Any point on the line is of the form (4λ+5,λ,3λ−6).
The distance between (4λ+5,λ,3λ−6)and(5,3,−6) is 3 units (given). Therefore
(4λ+5−5)2+(λ−3)2+(3λ−6+6)2=9
or 16λ2+λ2+9−6λ+9λ2=9
or 26λ2−6λ=0
or λ=0,3/13
The point is (5,0,−6).
b. The equation of the plane containing the lines x−23=y+35=z+57 and parallel to ˆi+4ˆj+7ˆk is
∣∣
∣
∣∣x−2y+3z+5147357∣∣
∣
∣∣=0
or x−2y+z−3=0
Point (−1,−2,0) lies on this plane.
c. The line passing through points A(2,−3,−1)andB(8,−1,2) is x−28−2=y+3−1+3=z+12+1
or x−26=y+32=z+13=λ (say).
Any point on this line is of the form P(6λ+2,2λ−3,3λ−1), whose distance from point
A(2,−3,−1) is 14 units. Therefore,
PA=14
or PA2=(14)2
⇒ (6λ)2+(2λ)2+(3λ)2=196
or 49λ2=196
or λ2=4
or λ=±2
Therefore, the required points are (14, 1, 5) and (−10,−7,−7). The point nearer to the origin is (14,1,5).
d. Any point on line AB,x2=y−23=z−34=λ is M(2λ,3λ+2,4λ+3). Therefore, the direction ratios of PM are 2λ−3,3λ+3and4λ−8.
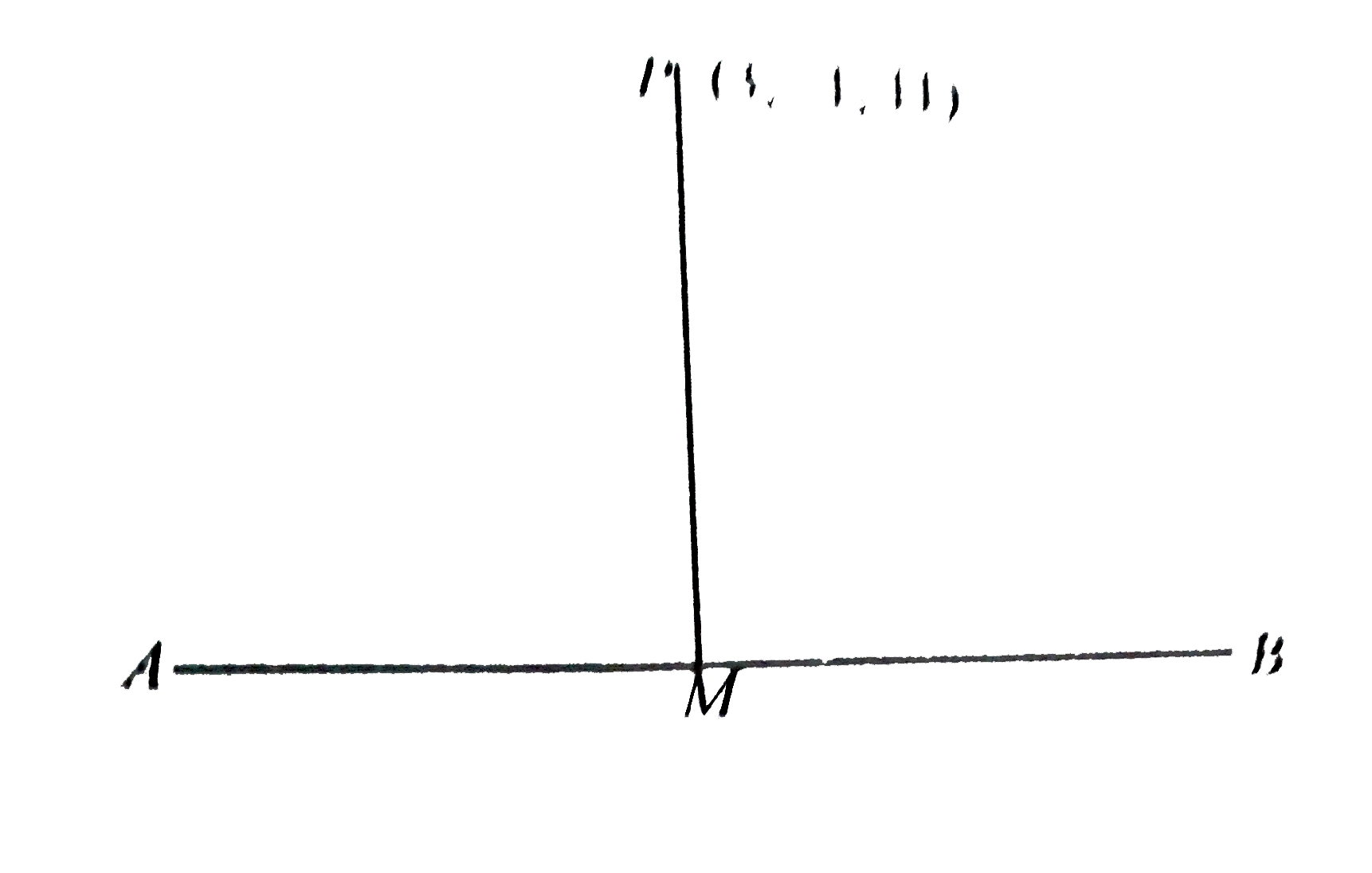
But PM⊥AB
∴ 2(2λ−3)+3(3λ+3)+4(4λ−8)=0
4λ−6+9λ+9+16λ−32=0
29λ−29=0
λ=1
Therefore, foot of the perpendicular is M(2,5,7).
Topper's Solved these Questions
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Numerical)
10 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - JEE Previous Year
26 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Comprehension)
12 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - CENGAGECHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - Question Bank
12 Videos
- TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONSEXERCISE - Question Bank
4 Videos
Similar Questions
Find the number of sphere of radius r touching the coordinate axes.
View Solution(i) Find the equation of the plane passing through the points(2,1,0),(5,0,1)and(4,11). (ii) If P s the point (2,1,6), then the find the point Q such that PQ is perpendicular to the plane in (i) and the midpoint of PQ lies on it.
View SolutionGiven four points A(2,1,0),B(1,0,1),C(3,0,1)andD(0,0,2). Point D lies on a line L orthogonal to the plane determined by the points A, B and C.
The equation of the plane ABC is
View SolutionThe equation of the plane which has the property that the point Q(5,4,5) is the reflection of point P(1,2,3) through that plane, is ax+by+cz=d where a,b,c,d∈N. Find the least value of (a+b+c+d).
View SolutionThe number of solution (s) of the equation
√2+cos2x=(sinx+cosx)∈[−π2,π]
View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk)and →r=2ˆi−ˆj−ˆk+μ(2ˆi+ˆj+2ˆk)
View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk) and →r=(2ˆi−ˆj−ˆk)+μ(2ˆi+ˆj+2ˆk)
View SolutionDetermine whether the following pair of lines intersect or not. (1) →r=ˆi−5ˆj+λ(2ˆi+ˆk);→r=2ˆi−ˆj+μ(ˆi+ˆj−ˆk) (2) →r=ˆi+ˆj−ˆk+λ(3ˆi−ˆj);→r=4ˆi−ˆk+μ(2ˆi+3ˆk)
View SolutionFind the distance between the parallel lines L1 and L2 whose vector equations are
→r=(ˆi+2ˆj+3ˆk)+λ(ˆi−ˆj+ˆk) and (2ˆi−ˆj−ˆk)+μ(ˆi−ˆj+ˆk)
View Solutionउन रेखाओ के बीच की न्यूनतम दूरी ज्ञात कीजिए जिनके सदिश समीकरण -
→r=ˆi+ˆj+λ(2ˆi−ˆj+ˆk) तथा →r=2ˆi+ˆj−ˆk+μ(3ˆi−5ˆj+2ˆk) है।
View Solutionरेखाओं →r=(ˆi−2ˆj+3ˆk)+λ(−ˆi+ˆj−2ˆk) और →r=(ˆi−ˆj+ˆk)+μ(ˆi+2ˆj−2ˆk) के मध्य न्यूनतम दूरी ज्ञात कीजिए।
View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk)and →r=2ˆi−ˆj−ˆk+μ(2ˆi+ˆj+2ˆk)
View SolutionFind the shortest distance between lines →r=6ˆi+2ˆj+ˆk+λ(ˆi−2ˆj+2ˆk)and →r=−4ˆi−ˆk+μ(3ˆi−2ˆj−2ˆk).
View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk) and →r=(2ˆi−ˆj−ˆk)+μ(2ˆi+ˆj+2ˆk)
View SolutionDetermine whether the following pair of lines intersect or not. (1) →r=ˆi−5ˆj+λ(2ˆi+ˆk);→r=2ˆi−ˆj+μ(ˆi+ˆj−ˆk) (2) →r=ˆi+ˆj−ˆk+λ(3ˆi−ˆj);→r=4ˆi−ˆk+μ(2ˆi+3ˆk)
View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk)and →r=2ˆi−ˆj−ˆk+μ(2ˆi+ˆj+2ˆk)
View Solution
