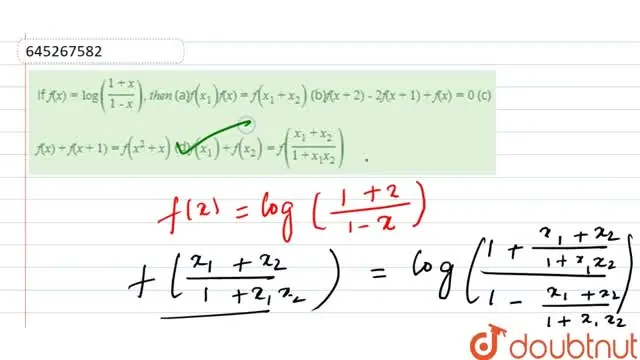
If f(x)=log(1+x1−x),then (a)f(x1)f(x)=f(x1+x2) (b)f(x+2)−2f(x+1)+f(x)=0 (c)f(x)+f(x+1)=f(x2+x) (d)f(x1)+f(x2)=f(x1+x21+x1x2)Solution in Tamil
f(x1)+f(x2)=ln(1+x11−x1)+ln(1+x21−x2)
ln[(1+x1)(1+x2)(1−x1)(1−x2)]
ln[1+x1+x2+x2x11−x1−x2+x1x2]−(1)
f(x1+x21+x1x2)=ln⎛⎜
⎜⎝1+x1+x21+x1x21−(x1+x21+x1x2)⎞⎟
⎟⎠
=ln(1+x1x2+x1+x21+x1x2−x1−x2)−(2)
f(x)+f(x+1)=ln(1+x1−x)+ln(1+x+11−x−1)
ln[(1+x)(2+x)(1−x)x]=ln(x2+3x+2x(1−x))
f(x2+x)=ln(1+x+x21−(x2+x))=ln(x2+x+11−x2−x).
Related Playlists
Similar Questions
Solve |x|+|x−2|=2.
02:36View SolutionThe number of triangles that can be formed with 10 points as vertices n of them being collinear, is 110. Then n is a. 3 b. 4 c. 5 d. 6
07:47View SolutionIf f is a real valued function, not identically zeroand satisfying f(x_1 + x_2)) + f(x_1 - x_2) = 2 f(x_1)f(x_2)forall x_1,x_2 , then f(x) is (1) An odd function(2) An even function (3) Neither even nor odd(4) f(0) = 1/2
03:38View SolutionFind the domain of definition of f(x)=loge.1−x1+x. Further show that,
f(x1)+f(x2)=f(x1+x21+x1x2),x1,x2∈(−1,1)07:30View SolutionI : If f(x) = cosh x + sinh x then f(x1+x2+...+xa)=f(x1)⋅f(x2)⋆f(xn)
II : If f(x) = cosh x + sinh x then f(x1)+f(x2)+...+f(xn)=f(x1)⋅f(x2)⋆f(xn)02:45View Solutionf(x)=loge(1−x1+x) অপেক্ষকটির সংজ্ঞার ক্ষেত্র নির্ণয় করো। দেখাও যে , f(x1)+f(x2)=f(x1+x21+x1x2),x1,x2∈(−1,1)
02:38View SolutionIf f:N→N,and x2>x1⇒f(x2)>f(x1) for x1,x2∈N a n df(f(n))=2n∀n∈N, then f(2)=_________
View Solutionif |f(x1)−f(x2)|≤(x1−x2)2Find the equation of tangent to the curve y=f(x) at the point (1,2).
04:28View SolutionLet x1,x2,x3 be the points where f(x)=|1−|x−4∣∣,x∈R is not differentiable then f(x1)+f(x2)+f(x3) =
05:48View SolutionIf f(x)>0" तथा "f(x)>0,AA x in R If any two real numbers x_1" तथा "x_2(x_1 ne x_2) for
View SolutionFind the domain of definition of f(x)=loge(1−x1+x) Further show thatf(x1)+f(x2)=f(x1+x21+x1x2),x1,x2∈(−1,1)
05:20View SolutionLet f(x)=x1(−x) and ' a ' be a real number. If x0=a, x1=f(x0),x2=f(x1),x3=f(x2) and so on. If x2009=1, then the value of reciprocal of ' a ' is
03:25View SolutionIf f(x)=log(1+x1−x),then (a)f(x1)f(x2)=f(x1+x2) (b)f(x+2)−2f(x+1)+f(x)=0 (c)f(x)+f(x+1)=f(x2+x) (d)f(x1)+f(x2)=f(x1+x21+x1x2)
03:20View SolutionIf f:N→N,and x2>x1⇒f(x2)>f(x1) for x1,x2∈N a n df(f(n))=3n∀n∈N, then f(2)=_________
02:35View Solutionif |f(x1)−f(x2)|≤(x1−x2)2Find the equation of gent to the curve y=f(x) at the point (1,2).
03:15View Solution