A particle executes S.H.M. along x-axis. The force acting on it is given by :
A cos (kx)
Ae−kx
Akx
−Akx
The correct Answer is:D
To solve the problem of identifying the correct force acting on a particle executing Simple Harmonic Motion (S.H.M.) along the x-axis, we can follow these steps:
1. Understanding the Force in S.H.M.:
The force acting on a particle in simple harmonic motion is given by Hooke's Law, which states that the force (F) is proportional to the displacement (x) from the equilibrium position and is directed towards that position. Mathematically, this is expressed as:
F=−kx
where k is a positive constant and the negative sign indicates that the force is directed opposite to the displacement.
Hint: Remember that in S.H.M., the force always acts towards the equilibrium position.
2. Analyzing the Given Options:
We need to evaluate the provided options for the force and determine which one matches the form of F=−kx.
- Option A: Acos(kx)
This expression does not have a linear relationship with x and does not satisfy the form of −kx. It also does not change direction based on the sign of x.
Hint: Check if the force is linear with respect to displacement.
- Option B: −kx2
This expression is quadratic in x and does not fit the linear form required for S.H.M. The force cannot be proportional to the square of the displacement.
Hint: The force should be linearly proportional to displacement, not quadratic.
- Option C: Akx
This expression is linear but lacks the negative sign. Therefore, it does not represent a restoring force as required in S.H.M.
Hint: Look for the negative sign that indicates the force is restoring.
- Option D: −Akx
This expression is in the correct form of F=−kx where Ak can be considered as a constant (let's denote it as k′). This indicates that the force is proportional to the displacement and directed towards the equilibrium position.
Hint: Ensure that the force has the correct direction indicated by the negative sign.
3. Conclusion:
After analyzing all the options, we find that the only expression that correctly represents the force acting on a particle in S.H.M. is:
F=−Akx
Therefore, the correct answer is Option D.
Final Answer:
The correct force acting on the particle executing S.H.M. along the x-axis is given by:
F=−Akx
The force acting on a particle in simple harmonic motion is given by Hooke's Law, which states that the force (F) is proportional to the displacement (x) from the equilibrium position and is directed towards that position. Mathematically, this is expressed as:
F=−kx
where k is a positive constant and the negative sign indicates that the force is directed opposite to the displacement.
We need to evaluate the provided options for the force and determine which one matches the form of F=−kx.
This expression does not have a linear relationship with x and does not satisfy the form of −kx. It also does not change direction based on the sign of x.
This expression is quadratic in x and does not fit the linear form required for S.H.M. The force cannot be proportional to the square of the displacement.
This expression is linear but lacks the negative sign. Therefore, it does not represent a restoring force as required in S.H.M.
This expression is in the correct form of F=−kx where Ak can be considered as a constant (let's denote it as k′). This indicates that the force is proportional to the displacement and directed towards the equilibrium position.
After analyzing all the options, we find that the only expression that correctly represents the force acting on a particle in S.H.M. is:
F=−Akx
Therefore, the correct answer is Option D.
The correct force acting on the particle executing S.H.M. along the x-axis is given by:
F=−Akx
Topper's Solved these Questions
- OSCILLATIONS BOOK - PHYSICS WALLAHCHAPTER - OSCILLATIONS EXERCISE - NEET PAST 5 YEARS QUESTIONS
5 Videos
- OSCILLATIONS BOOK - PHYSICS WALLAHCHAPTER - OSCILLATIONS EXERCISE - NEET PAST 5 YEARS QUESTIONS
5 Videos
- MOVING CHARGES AND MAGNETISM BOOK - PHYSICS WALLAHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - NEET PAST 5 YEARS QUESTIONS
12 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTS BOOK - PHYSICS WALLAHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTS EXERCISE - NEET PAST 5 YEARS QUESTIONS
16 Videos
Similar Questions
A particle executes S.H.M. of 2 cm. At the extreme position, the force is 4 N. What is the force at a point midway between mean and extreme positions ?
View SolutionA particle is executing S.H.M. along a straight line. The graph showing the variation of kinetic, potential and total energy K, U and T respectively with displacement is -
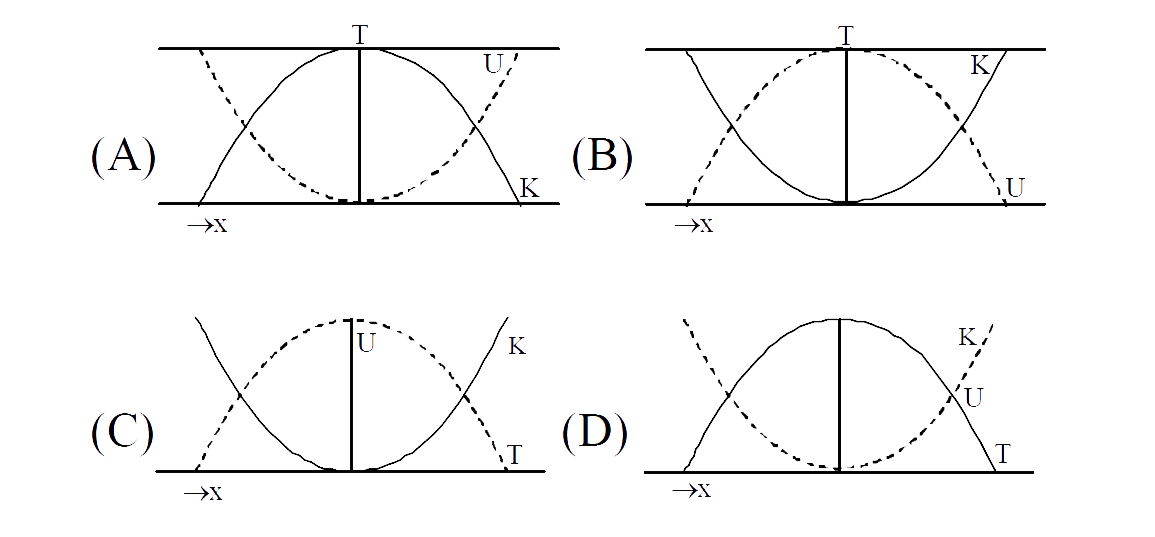
View Solution
Knowledge Check
For a particle executing SHM along x-axis, force acting on it, is given by (A,k>0)
A−AkxBA cos kxCA exp (-kx)DAkxStatement-I : A particle is moving along x-axis. The resultant force F acting on it is given by F=−ax−b, wher a and b are both positive constants. The motion of this particle is not S.H.M.
Statement-II : In S.H.M. resultant force must be proportional to the dispacement form mean position.AStatement-I is true, Statement-Ii is true, Statement-II is a correct explanation for Statement-I.BStatement-I is true, Statement-II is true, Statement-II is NOT a correct explanantion for Statement-I.CStatement-I is true, Statement-II is falseDStatement-I is false, Statement-II is trueA particle is moving along the x-axis and force acting on it is given by F=F0sinωxN, where ω is a constant. The work done by the force from x=0 to x=2 will be
AF0ω(1−cosω)BF02ω(1−cos2ω)C2F0sin2ωωDF0ω(1+cos2ω)
A particle executes S.H.M. of amplitude A . At what positions of its displacement (x), will its acceleration be zero and maximum ?
View SolutionA particle moving along the X-axis executes simple harmonic motion, then the force acting on it is given by
where, A and K are positive constants.
View SolutionA particle is moving along the x-axis whose acceleration is given by a=3x−4, where x is the location of the particle. At t = 0, the particle is at rest at x=4/3m. The distance travelled by the particles in 5 s is
View SolutionTwo particles execute S.H.M. along the same line at the same frequency. They move in opposite direction at the mean position. The phase difference will be :
View SolutionA particle is executing S.H.M. along a straight line. The graph showing the variation of kinetic, potential and total energy K, U and T respectively with displacement is -

View Solution
