Starting from the centre of the earth having radius R the variation of g (acceleration due to gravity) is shown by
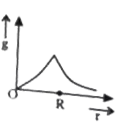
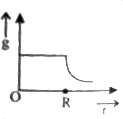
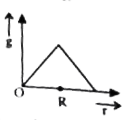
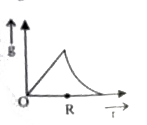
The correct Answer is:D
To solve the question regarding the variation of acceleration due to gravity (g) starting from the center of the Earth with radius R, we will derive the relationship and analyze the options provided.
1. Understanding the Concept:
- The acceleration due to gravity (g) varies with depth inside the Earth. At the surface of the Earth, g is maximum, and it decreases as we go deeper.
2. Formula for g at Depth:
- The formula for the acceleration due to gravity at a depth d inside the Earth is given by:
g′=g(1−dR)
- Here, g′ is the acceleration due to gravity at depth d, g is the acceleration due to gravity at the surface, R is the radius of the Earth, and d is the depth below the surface.
3. Analyzing the Formula:
- From the formula, we can see that as d increases (i.e., as we go deeper), g′ decreases linearly. This indicates a linear relationship between g′ and d.
4. Graphical Representation:
- If we plot g′ against d, the graph will be a straight line starting from g at d=0 (surface) and decreasing to 0 at d=R (center of the Earth).
5. Conclusion:
- Therefore, the variation of g as we move from the center of the Earth to the surface is linear and decreases from g to 0. This means that the correct option must represent a linear decrease of g with increasing depth.
Final Answer:
The correct option is the one that shows a linear decrease in g as we move from the surface to the center of the Earth.
- The acceleration due to gravity (g) varies with depth inside the Earth. At the surface of the Earth, g is maximum, and it decreases as we go deeper.
- The formula for the acceleration due to gravity at a depth d inside the Earth is given by:
g′=g(1−dR)
- Here, g′ is the acceleration due to gravity at depth d, g is the acceleration due to gravity at the surface, R is the radius of the Earth, and d is the depth below the surface.
- From the formula, we can see that as d increases (i.e., as we go deeper), g′ decreases linearly. This indicates a linear relationship between g′ and d.
- If we plot g′ against d, the graph will be a straight line starting from g at d=0 (surface) and decreasing to 0 at d=R (center of the Earth).
- Therefore, the variation of g as we move from the center of the Earth to the surface is linear and decreases from g to 0. This means that the correct option must represent a linear decrease of g with increasing depth.
The correct option is the one that shows a linear decrease in g as we move from the surface to the center of the Earth.
Topper's Solved these Questions
- GRAVITATIONBOOK - PHYSICS WALLAHCHAPTER - GRAVITATIONEXERCISE - Level- 2
30 Videos
- GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTSBOOK - PHYSICS WALLAHCHAPTER - GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTSEXERCISE - NEET Past 5 Years Questions
14 Videos
- KINETIC THEORY BOOK - PHYSICS WALLAHCHAPTER - KINETIC THEORY EXERCISE - NEET PAST 5 YEARS QUESTIONS
11 Videos
Similar Questions
Knowledge Check
At the centre of earth the acceleration due to gravity is
AinfiniteBzeroC9.8ms−2Dall of theseIf g is acceleration due to gravity on the surface of the earth, having radius R, the height at which the acceleration due to gravity reduces to g/2 is
AR/2B√2RCR√2D(√2−1)ROne goes from the centre of the earth to a distance two third the radius of the earth. The acceleration due to gravity is highest at
Athe centre of the earthBa distance half the radius of the earthCa distance one-third of the radius of the earthDa distance two-third of the radius of the earth
Starting from the surface of earth, the variation of acceleration due to gravity (g) with height (h) can be represented by
View SolutionObtain an expression for the acceleration due to gravity on the surface of the earth in terms of mass of the earth and its radius. Discuss the variation of acceleration due to gravity with altitude and depth. If a body is taken to a height R / 4 from the surface of the earth, find percentage decrease in the weight of the body ? Here R is the radius of the earth.
View SolutionIf g is acceleration due to gravity on the surface of the earth,having radius R ,the height at which the acceleration due to gravity reduces to g/2 is
View SolutionDoes the concentration of the earth's mass near its centre change the variation of g (acceleration due to gravity) with height from its surface?
View SolutionAt what distance from the centre of the earth, the value of acceleration due to gravity g will be half that on the surface ( R = radius of earth)
View Solution
