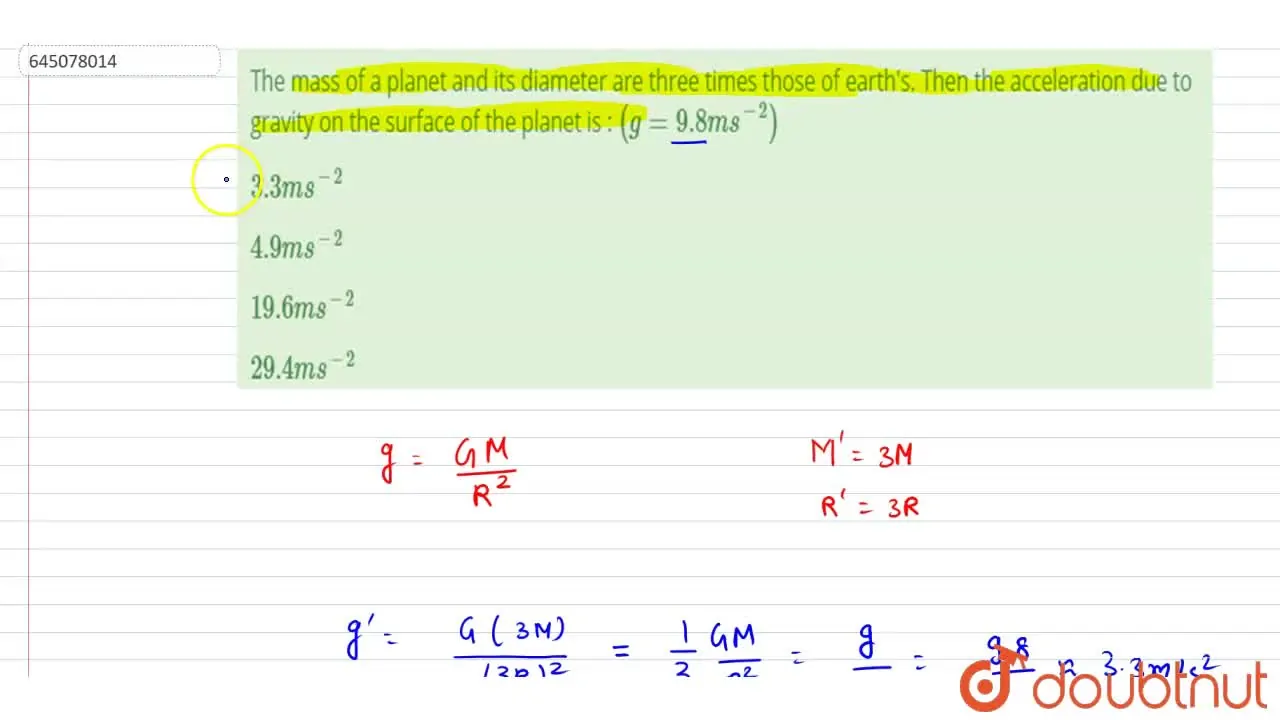
The mass of a planet and its diameter are three times those of earth's. Then the acceleration due to gravity on the surface of the planet is : (g=9.8ms−2)
3.3ms−2
4.9ms−2
19.6ms−2
29.4ms−2
The correct Answer is:A
Step by step video & image solution for The mass of a planet and its diameter are three times those of earth's. Then the acceleration due to gravity on the surface of the planet is : (g =9.8 ms^(-2)) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
The mass and diameter of a planet have twice the value of the corresponding parameters of earth. Acceleration due to gravity on the surface of the planet is
View SolutionHow is the acceleration due to gravity on the surface of the earth related to its mass and radius ?
View SolutionThe mass of a planet is twice the mass of earth and diameter of the planet is thrie the diameter of the earth, then the acceleration due to gravity on the planet's surface is
View SolutionThe density of a newly discovered planet is twice that of earth. The acceleration due to gravity at the surface of the planet is equal to that at the surface of the earth. If the radius of the earth is R, the radius of the planet would be
View SolutionAssuming the mass of Earth to be ten times the mass of Mars, its radius to be twice the radius of Mars and the acceleration due to gravity on the surface of Earth is 10m/s2 . Then the accelration due to gravity on the surface of Mars is given by
View SolutionMass and radius of a planet are two times the value of earth. What is the value of escape velocity from the surface of this planet?
View SolutionIf the density of the planet is double that of the earth and the radius 1.5 times that of the earth, the acceleration due to gravity on the planet is
View SolutionImagine a new planet having the same density as that of earth but 3 times bigger than the earth in size. If the acceleration due to gravity on the surface of earth is g and that on the new plane is g , then :
View SolutionThe moon's radius is 1/4 that of the earth and its mass 1/80 times that of the earth. If g represents the acceleration due to gravity on the surface of the earth, that on the surface of the moon is
View Solutionimagine a new planet having the same density as that of earth but it is 3 times bigger than the earth is size. If the acceleration due to gravity on the surface of earth is g and that on the surface of the new planet is g', then find the relation between g and g'.
View Solution