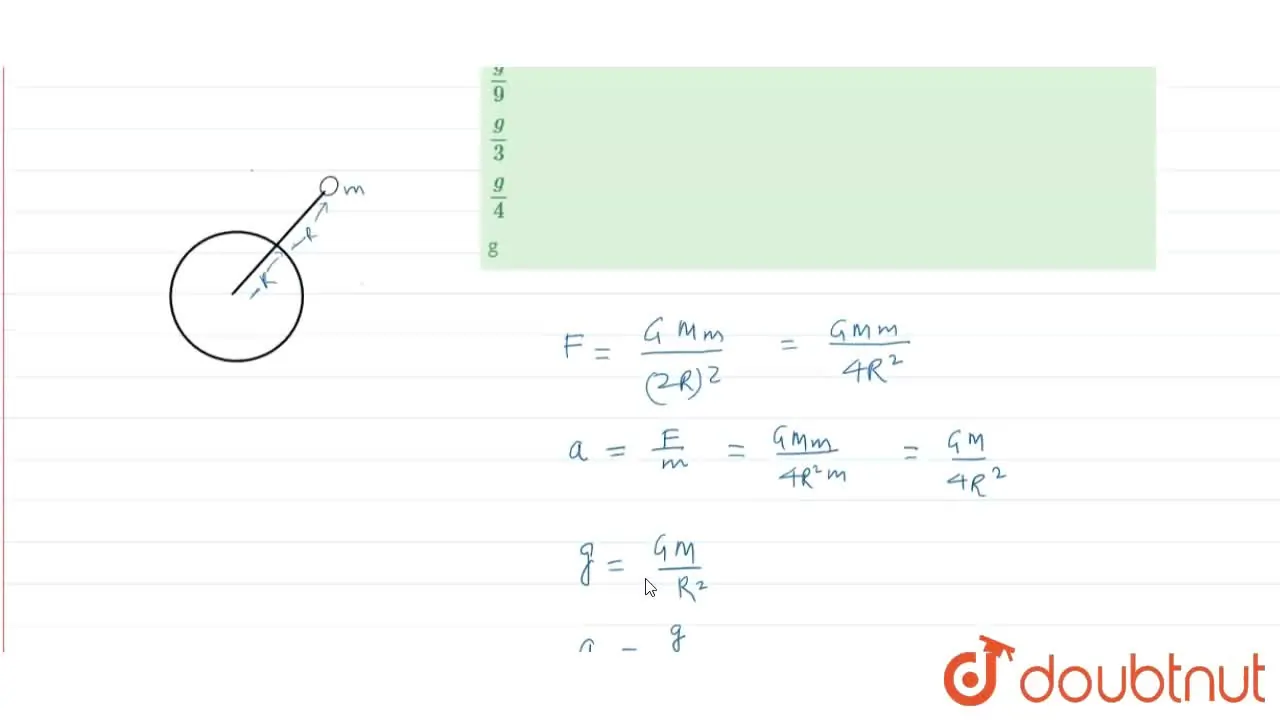
The acceleration of a body due to the attraction of the earth (radius R) at a distance 2R form the surface of the earth is (g=acceleration due to gravity at the surface of the earth)
g9
g3
g4
g
The correct Answer is:A
Step by step video & image solution for The acceleration of a body due to the attraction of the earth (radius R) at a distance 2R form the surface of the earth is (g=acceleration due to gravity at the surface of the earth) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
The value of acceleration due to gravity at the surface of earth
View SolutionThe acceleration due to gravity at a depth R/2 below the surface of the earth is
View SolutionAt what depth from the surface of the earth, the acceleration due to gravity will be half the value of g on the surface of the earth ?
View SolutionCalculate the distance from the surface of the earth at which above the surface, acceleration due to gravity is the same as below the earth.
View SolutionIf the earth were to spin faster, acceleration due to gravity at the poles :
View SolutionAt what depth from the surface of the earth, the acceleration due to gravity will be 14 th the value of g on the surface of the earth ?
View SolutionA satellite of mass m revolves around the earth of radius R at a hight x from its surface. If g is the acceleration due to gravity on the surface of the earth, the orbital speed of the satellite is
View SolutionThe ratio of acceleration due to gravity at a height 3 R above earth's surface to the acceleration due to gravity on the surface of earth is
(R = radius of earth)View SolutionIf the radius of earth were to decrease by 1%, its mass remaining the same, the acceleration due to gravity on the surface of the earth will
View SolutionAt what depth below the surface of the earth acceleration due to gravity will be half its value at 1600 km above the surface of the earth ?
View Solution