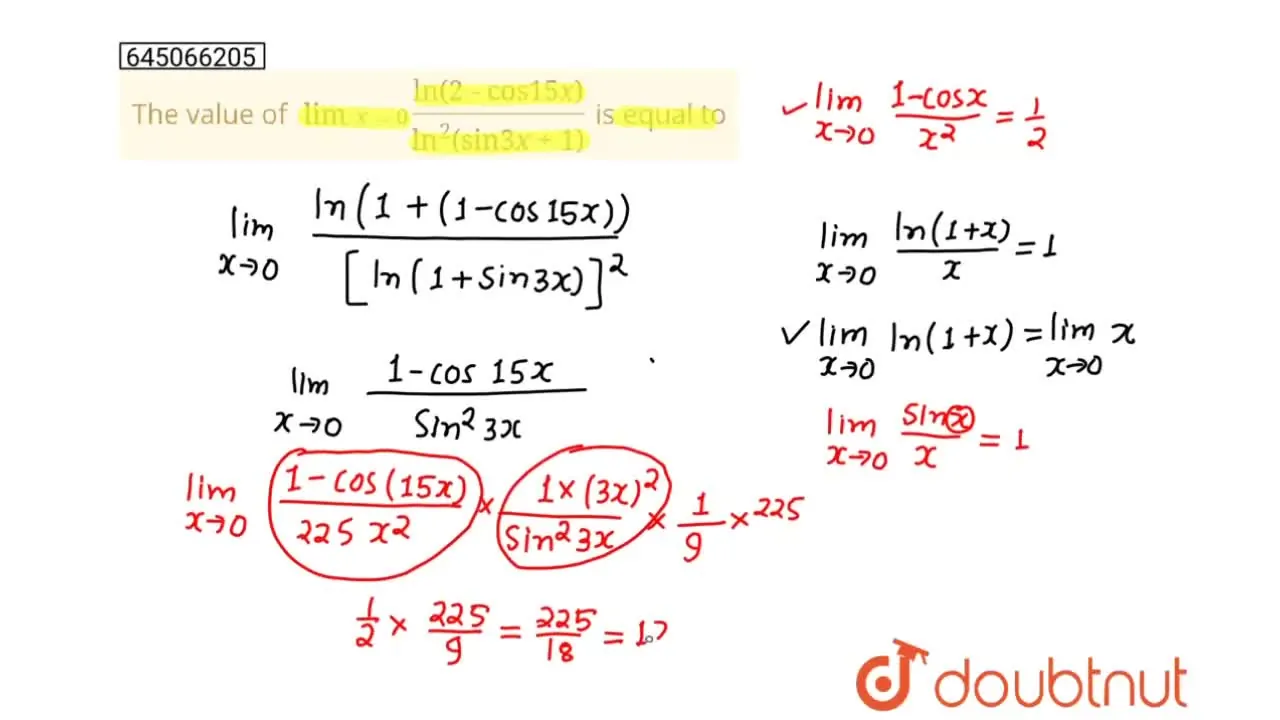
The value of limx→0ln(2−cos15x)ln2(sin3x+1) is equal to
Video Solution
Text Solution
The correct Answer is:12.5
|
Answer
Step by step video & image solution for The value of lim_(xrarr0)(ln(2-cos15x))/(ln^(2)(sin3x+1)) is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Similar Questions
The value of limx→0ln(10−9cos2x)ln2(sin3x+1) is equal to
View SolutionThe value of limx→09ln(2−cos25x)5ln2(sin3x+1) is equal to
View Solutionlimx→0(1−cosxx2)
View SolutionThe value of limx→0ex−cos2x−xx2 , is
View SolutionThe value fo limx→01−cos4x(sin2xcosx)is equal to
View SolutionThe value of limx→01−cos3xsin2xcosx is equal to
View SolutionThe value of limx→01−cos3xxsinxcosx
View SolutionThe value of limx→0((1−cos4x)(5+cosx)xtan5x) is equal to
View SolutionThe value of limx→2π1−(secx)secxln(secx) is equal to
View SolutionThe value of limx→0x2sin(1x)sinx, is
View Solution