The exponential growth can be expressed as wt=w0en where e denotes
growth rate
time of growth
base of natural logarithm
final size
The correct Answer is:C
To solve the question regarding the expression of exponential growth, we will break it down step by step.
1. Understanding the Equation: The exponential growth can be expressed as Wt=W0e(n). Here, Wt represents the size at time t, W0 is the initial size, and e is a mathematical constant.
2. Identifying Components:
- W0: This is the initial size or the starting amount at the beginning of the observation period.
- n: This usually represents the growth rate multiplied by time, often denoted as n=rt, where r is the growth rate and t is the time.
3. Understanding 'e': The letter 'e' in the equation stands for the base of the natural logarithm. It is an important constant in mathematics, approximately equal to 2.71828. This constant is used in exponential growth models because it describes continuous growth processes.
4. Conclusion: Therefore, in the context of the given equation, e denotes the base of the natural logarithm.
Final Answer:
The correct answer is that e denotes the base of the natural logarithm.
---
- W0: This is the initial size or the starting amount at the beginning of the observation period.
- n: This usually represents the growth rate multiplied by time, often denoted as n=rt, where r is the growth rate and t is the time.
The correct answer is that e denotes the base of the natural logarithm.
Similar Questions
The exponential growth can be mathematically expressed as
View SolutionTwo coherent sources emit light waves which superimpose at a point where these can be expressed as
E1=E0sin(ωt+π/4)
E2=2E0sin(ωt−π/4)
Here, E1andE2 are the electric field strenghts of the two waves at the given point.
If I is the intensity of wave expressed by field strenght E1, find the resultant intensity
View Solution
Knowledge Check
Exponential growth is expressed as
ALt=L0+rtBL0=LertCW1=W0+ertDW0=W1ertThe exponential growth can be expressed as W1=W0ertris –
ARelative growth rate and depends on final sizeBIt is initial sizeCRelative growth and also referred to as efficiency indexDNone of the aboveExponential growth in plants can be expressed as
ALt=L0+rtBLe=LtrtCW1=W0ertDW1=W0ert
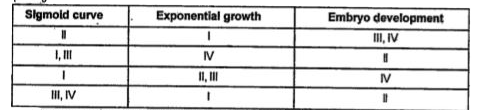
Go through the following points I. Slow growth, thereafter exponential growth and then stationary phase II. Geometric and arithmetic phases III. Characteristic of all living organisms growing in natural environment IV. W1=W0ert
View SolutionIf f(x) is a polynomial of nth degree then ∫exf(x)dx= Where fn(x) denotes nth order derivative of f(x) w.r.t.x
View SolutionShow that every square matrix A can be uniquely expressed as P+iQ,wherePandQ are Hermitian matrices.
View SolutionExponential growth in plant can be expressed as :-
View SolutionIn exponential growth formula, Wt=W0ert,e denotes
View Solution
