Assuming that the energy transfer efficiency between trophic levels is 10%, how much grain would be required to produce 70 kg of human biomass if the grain is eaten by cows and the cows are eaten by humans?
210 kg
700 kg
2,100 kg
7,000 kg
The correct Answer is:D
To solve the problem of how much grain is required to produce 70 kg of human biomass, given that the energy transfer efficiency between trophic levels is 10%, we can follow these steps:
1. Understand the Trophic Levels:
- In this scenario, there are two trophic levels:
- First trophic level: Grain (food source)
- Second trophic level: Cows (primary consumers)
- Third trophic level: Humans (secondary consumers)
2. Energy Transfer Efficiency:
- The energy transfer efficiency between trophic levels is given as 10%. This means that only 10% of the energy (or biomass) from one level is passed on to the next level.
3. Calculate the Biomass Needed at Each Level:
- We need to find out how much biomass is needed at the cow level to produce 70 kg of human biomass.
- Since only 10% of the biomass from cows is converted into human biomass, we can set up the equation:
Biomass needed from cows=Human biomassEfficiency=70 kg0.10=700 kg
4. Calculate the Grain Required for Cows:
- Now, we need to find out how much grain is required to produce 700 kg of cow biomass.
- Again, using the same efficiency:
Grain needed=Biomass needed from cowsEfficiency=700 kg0.10=7000 kg
5. Final Answer:
- Therefore, the total amount of grain required to produce 70 kg of human biomass is 7000 kg.
Summary of Steps:
- Calculate the biomass needed at the cow level using the efficiency.
- Calculate the grain needed to produce that cow biomass using the same efficiency.
- In this scenario, there are two trophic levels:
- First trophic level: Grain (food source)
- Second trophic level: Cows (primary consumers)
- Third trophic level: Humans (secondary consumers)
- The energy transfer efficiency between trophic levels is given as 10%. This means that only 10% of the energy (or biomass) from one level is passed on to the next level.
- We need to find out how much biomass is needed at the cow level to produce 70 kg of human biomass.
- Since only 10% of the biomass from cows is converted into human biomass, we can set up the equation:
Biomass needed from cows=Human biomassEfficiency=70 kg0.10=700 kg
- Now, we need to find out how much grain is required to produce 700 kg of cow biomass.
- Again, using the same efficiency:
Grain needed=Biomass needed from cowsEfficiency=700 kg0.10=7000 kg
- Therefore, the total amount of grain required to produce 70 kg of human biomass is 7000 kg.
- Calculate the biomass needed at the cow level using the efficiency.
- Calculate the grain needed to produce that cow biomass using the same efficiency.
Similar Questions
Food chains and the energy flow within an ecosystem provide an important understanding of contingencies and mutual dependencies of organisms. The given below flow chart depicts the energy flow within some members of a grassland ecosystem. The grass in the below ecosystem transducer 120,000 J of sunlight and fixes it into 12,000 J of energy. It is established that 90% of the energy of one trophic level is not passed to the next trophic level. Further assume that the energy transferred from one trophic level to the next is equally shared among the different organisms at that trophic level.
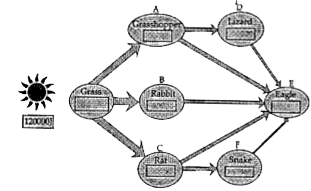
How many food chains are present in the food web depicted above?
View SolutionFood chains and the energy flow within an ecosystem provide an important understanding of contingencies and mutual dependencies of organisms. The given below flow chart depicts the energy flow within some members of a grassland ecosystem. The grass in the below ecosystem transducer 120,000 J of sunlight and fixes it into 12,000 J of energy. It is established that 90% of the energy of one trophic level is not passed to the next trophic level. Further assume that the energy transferred from one trophic level to the next is equally shared among the different organisms at that trophic level.
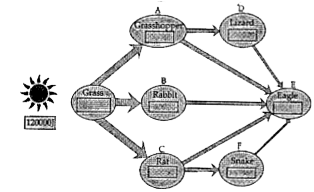
In the food web depicted above, identify the most energy efficient link for tertiary consumer.
View Solution
Knowledge Check
If the electron in a hydrogen atom were in the energy level with n 3, how much energy in joule would be required to ionise the atom ? ( Ionisation energy of H - atoms is 2.18×10−18J ) :
A6.54×10−19B1.43×10−19C2.42×10−19D3.14×10−20A person trying to lose weight by burning fat filts a mass of 10kg upto a being of 1m1000 time . Assume that the potential energy lost each time be lower the mass is dissipated . How much far will be use up considering the work done only when the weight is lifted up ? Far supplies 3.8×107J of energy per kg wich is canverted to mechanical energy with x20% efficiency rate Take =9.8ms−2
A9.89×10−3kgB12.89×10−3kgC2.45×10−3kgD6.45×10−3kgAssume that the green plants in an ecosystem produce 1000 grams of dry mass per square meter per year and there is an efficiency of 10 percent in each transfer of energy from one trophic level to the next. How many total grams of dry mass will be produced by the tertiary consumers per square meter per year?
A100B10C1D0.1
Polonium (_84210Po)emits_2^4Hepartic≤sandisconverted∫o≤ad(_82^206Pb).Thisreactionisusedfor∏uc∈ge≤ctricpower∈aspacemission.Po^210hashalf−lifeof138.6days.As∑∈ganefficiencyof10%forthethermoe≤ctricmaχ≠,howμch^210Poisrequired→∏uce1.2xx10^7Jofe≤ctrice≠rgyperdayattheendof693days.Alsof∈dthe∈itialactivityofthematerial.<br>Given:Massesofνc≤i<br>^210Po=209.98264aμ,^206Pb=205.97440aμ,_2^4He=4.00260aμ,<br>1 am u=931 MeV//c^2andAvogadro'sνmber = 6xx10^23//mol
View SolutionCalculate the quantity of electricity that would be required to reduce 12.3g of nitrobenzene to aniline, if the current efficiency for the process is 50%. If the potential drop across the cell is 3.0V, how much energy will be consumed?
View SolutionCalculate the quantity of electricity that would be required to reduce 12.3g of nitrobenzene to aniline, if current efficiency is 50% . If the potential drops across the cell is 3.0V, how much energy will be consumed ?
View SolutionCalculate the quantity of electricity that would be required to reduce 12.3g of nitrobenzene to aniline, if the current efficiency for the process is 50%. If the potential drop across the cell is 3.0V, how much energy will be consumed?
View SolutionAt a place, the solar energy incident in one second is 640 J, on an area of one square metre. Assuming that all the solar energy is absorbed by 10 kg water, incident on its surface of area 400 cm2. How much time will it take to raise its temperature by 70∘C? (specific heat capacity of water is 4.2 J g−1.∘C−1)
View Solution
