It has been experimentally observed that the electric field in a large region of the earth.s atmosphere is directed vertically down. At an altitude of 300m, the electric field is 60Vm−1. At an altitude of 200m, the field is 100Vm−1. Calculate the net amount of charge contained in the cube of 100m edge located between 200m and 300m altitude.Solution in Odia
Let E1 be electric field at 200m
and E1=100V/m (given), E2 be electric field at 300m and E2=60V/m(given)
As wire of 100m edge is situated in electric field with its area normal to electric field, so flux through the cube at height of 200m is
ϕ1=E1.A=E1Acos0∘
=E1A=100×(100×100)
=10×105V−m
Flux through the cube at height of 300m is
ϕ2=E2.A=E2Acos180∘
=−[60×(100×100)]
=−6×105V−m
∴ Net flux through the cube is
ϕ=ϕ1+ϕ2
⇒ϕ=10×105−6×105⇒ϕ=4×105V−m
By Gauss.s theorem, ϕ=qε0 (where, q is net amount of charge)
q=ϕε0=(4×105)(8.85×10−12)
=3.54×10−6C
Similar Questions
An electric pole stands at the vertex A of the equilateral triangular field and subtends an angle 60^(@) at either of the remaining vertices. If the altitude of the field is 50 meters, the height of the pole is
View SolutionThe length of a rectangular field is 100m. If its perimeter is 300m, what is its breadth?
View SolutionThe electric field in a large region of earth's atmosphere is directed vertically down. At an altitude of 300m, the electric field is 60 V/m. At an altitude of 200m, the electric field is 100 V/m. The net amount of charge contained in the cube of 100m edge, located between 200m and 300m altitude is
View SolutionIn a region, electric field depends on X-axis as E = E_0x^2 . There is a cube of edge a as shown. Then find the charge enclosed in that cube.
View SolutionIt has been experimentally observed that the electric field in a large region of earth's atmosphere is directed vertically down. At an altitude of 300 m, the electric field 60Vm−1. At an altitude of 200 m, the field is 100 V m^(-1), the field is 100Vm−1. Calculate the net amount of charge contained in the cube of 100 m edge, located between 200 and 300 m altitude.

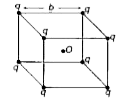
View SolutionA cube of side b has a charge q at each of its vertices. Determine the potential and electric field due to this charge array at the centre of the cube.
Here, we have to find the electric potential and electric field due to multiple charge so we have to apply superposition principle to calculate net electric field and potential
View SolutionAn electric pole stands at the vertex A of the equilateral triangular field and subtends an angle 60^(@) at either of the remaining vertices. If the altitude of the field is 50 meters, the height of the pole is
View Solution
