Examine that sin |x| is a continuous function.
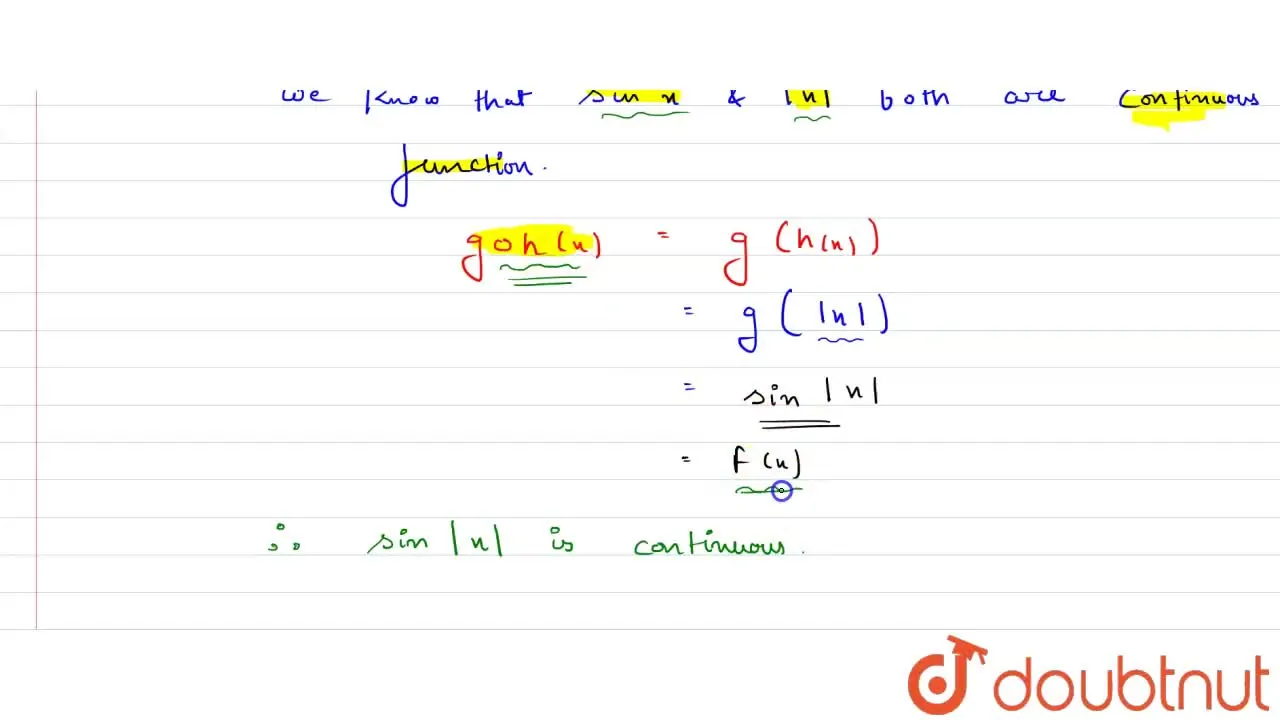
Let g(x)=|x| and h(x) = sin x
We know that g(x) = |x| is continuous for x ∈ R.
and h(x) = cos x is also continuous for each x ∈ R.
Now (hog) (x) = h[g(x)]=h[|x|]=sin|x|
∵ g(x) and h(x) both are continuous functions.
∴ Composite function (hog) (x) is also a continuous function.
⇒sin|x| is continuous for all x ∈ R.
Related Playlists
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - Continuity and DifferentiabilityEXERCISE - Exercies 5.210videos
Continuity and Differentiability
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - Continuity and DifferentiabilityEXERCISE - Exercies 5.315videos
Continuity and Differentiability
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - Continuity and DifferentiabilityEXERCISE - Exercies 5p20videos
Continuity and Differentiability
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - APPLICATIONS OF INTEGRALSEXERCISE - Miscellaneous Exercise19videos
APPLICATIONS OF INTEGRALS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - DETERMINANTSEXERCISE - Miscellaneous Exercise19videos
DETERMINANTS
Similar Questions
Show that the function defined by f(x)=|cosx| is a continuous function.
View SolutionProve that the function defined by f(x)=tanxis a continuous function.
View SolutionShow that the function defined by f(x)=sin(x2)is a continuous function.
View SolutionIs the function defined by f(x)=|x|, a continuous function?
View SolutionShow that the function defined by f(x)=cos(x2)is a continuous function.
View SolutionDetermine if f defined byf(x)={x2sin(1x),ifx≠0,0ifx=0 is a continuous function?
View SolutionShow that the function f defined by f(x)=|1−x+x|, where x is any real number, is a continuous function.
View SolutionStatement -1 : y = [x] , ([.] denotes greatest integer function) is not a continuous function .
Statement -2 : {x} ({.} denotes fractional fractional function) is discontinuous at integral points.
Statement -3 : y=7x is continuous in its domain.View SolutionThe composition of two continuous function is a continuous function.
View SolutionThe composition of two continuous function is a continuous function.
View Solution