Prove that tangent function is continous in its domain.
To prove that the tangent function y=tanx is continuous in its domain, we will follow these steps:
Step 1: Define the Tangent Function
The tangent function is defined as:
y=tanx=sinxcosx
Step 2: Identify the Domain of the Tangent Function
The tangent function is continuous wherever it is defined. The function tanx is undefined when cosx=0. The cosine function is zero at odd multiples of π2:
x=(2n+1)π2,n∈Z
Thus, the domain of tanx is:
Domain=R∖{(2n+1)π2:n∈Z}
Step 3: Continuity of Sine and Cosine Functions
The sine and cosine functions are continuous everywhere on the real line:
- sinx is continuous for all x∈R
- cosx is continuous for all x∈R
Step 4: Continuity of the Quotient of Functions
The quotient sinxcosx is continuous wherever the denominator cosx is not zero. Since we have established that cosx is zero at odd multiples of π2, the function tanx is continuous in its domain, which excludes these points.
Step 5: Conclusion
Therefore, we conclude that the tangent function tanx is continuous in its domain:
Continuous in R∖{(2n+1)π2:n∈Z}
The tangent function is defined as:
y=tanx=sinxcosx
The tangent function is continuous wherever it is defined. The function tanx is undefined when cosx=0. The cosine function is zero at odd multiples of π2:
x=(2n+1)π2,n∈Z
Thus, the domain of tanx is:
Domain=R∖{(2n+1)π2:n∈Z}
The sine and cosine functions are continuous everywhere on the real line:
- sinx is continuous for all x∈R
- cosx is continuous for all x∈R
The quotient sinxcosx is continuous wherever the denominator cosx is not zero. Since we have established that cosx is zero at odd multiples of π2, the function tanx is continuous in its domain, which excludes these points.
Therefore, we conclude that the tangent function tanx is continuous in its domain:
Continuous in R∖{(2n+1)π2:n∈Z}
Topper's Solved these Questions
- Continuity and DifferentiabilityBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - Continuity and DifferentiabilityEXERCISE - Exercies 5a
30 Videos
- Continuity and DifferentiabilityBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - Continuity and DifferentiabilityEXERCISE - Exercies 5b
8 Videos
- APPLICATIONS OF INTEGRALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - APPLICATIONS OF INTEGRALSEXERCISE - Miscellaneous Exercise
19 Videos
- DETERMINANTSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - DETERMINANTSEXERCISE - Miscellaneous Exercise
19 Videos
Similar Questions
Prove that cot x is continuous in its domain
View SolutionStatement -1 : y = [x] , ([.] denotes greatest integer function) is not a continuous function .
Statement -2 : {x} ({.} denotes fractional fractional function) is discontinuous at integral points.
Statement -3 : y=7x is continuous in its domain.
View SolutionIf f(x)=[x]sin(π[x+1]), where [.] denotes the greatest integer function, then the set of point of discontiuity of f in its domain is
View SolutionDefine greatest integer function. Write its domain and range.
View SolutionShow that y='log(1+x)-(2x)/(2+x)', is an increasing function of x throughout its domain.
View SolutionWhich of the following function is continuous everywhere but not differentiable at atleat one point in its domain
View SolutionWhich of the following relation are functions? Give reasons. In cane of a function, determine its domain and range.
{(1,0),(1,−1),(2,3),(4,10)}
View SolutionWhich of the following relation are functions? Give reasons. In cane of a function, determine its domain and range.
{(1,−2),(3,7),(4,−6),(8,11)}
View SolutionShow that y=log(1+x)−2x2+x,x>1 is an increasing function of x throughout its domain.
View SolutionThe functions defined have domain R
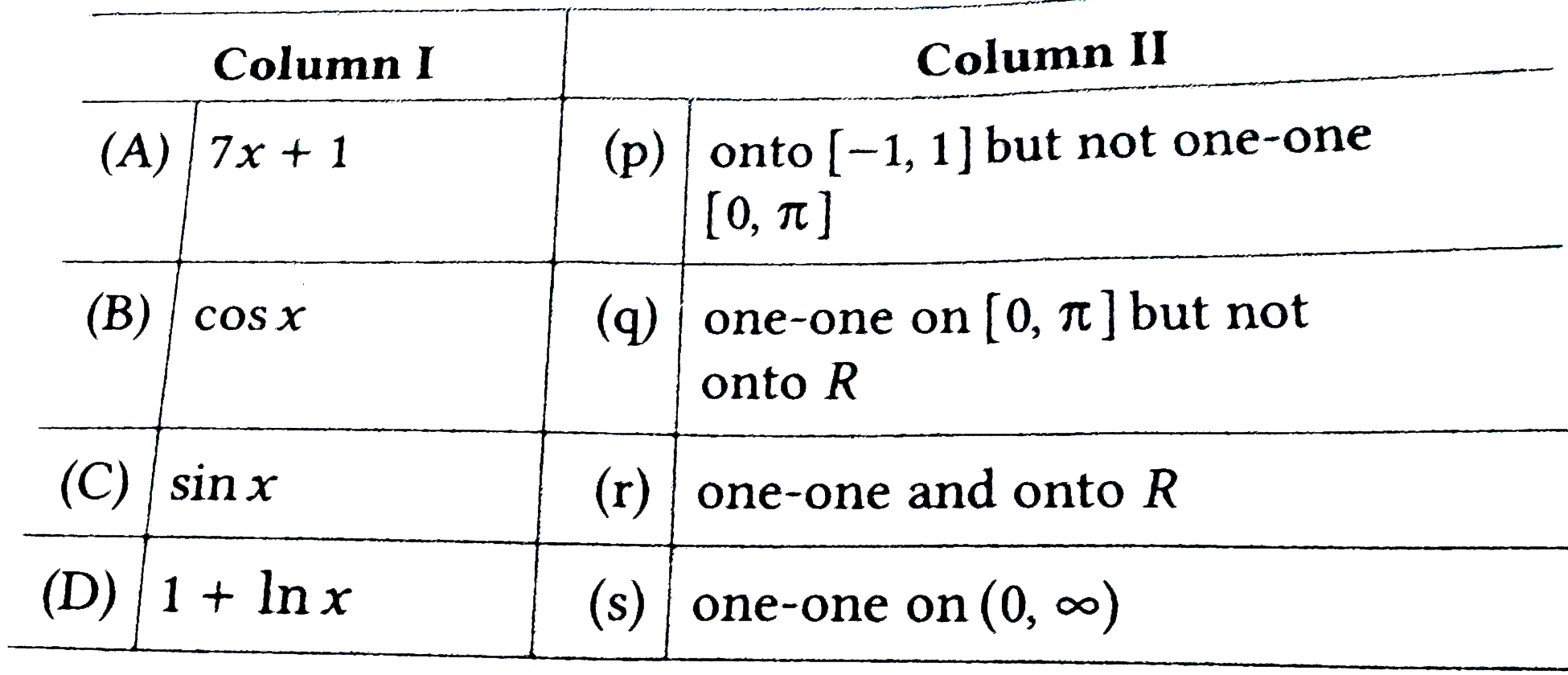
View Solution
