In Figure, $A B C$ is a right triangle right angled at $B$ and points $D a n d E$ trisect $B C$ . Prove that $8 A E^{2} = 3 A C^{2} + 5 A D^{2} .$

Video Solution

Text Solution

Verified by Experts

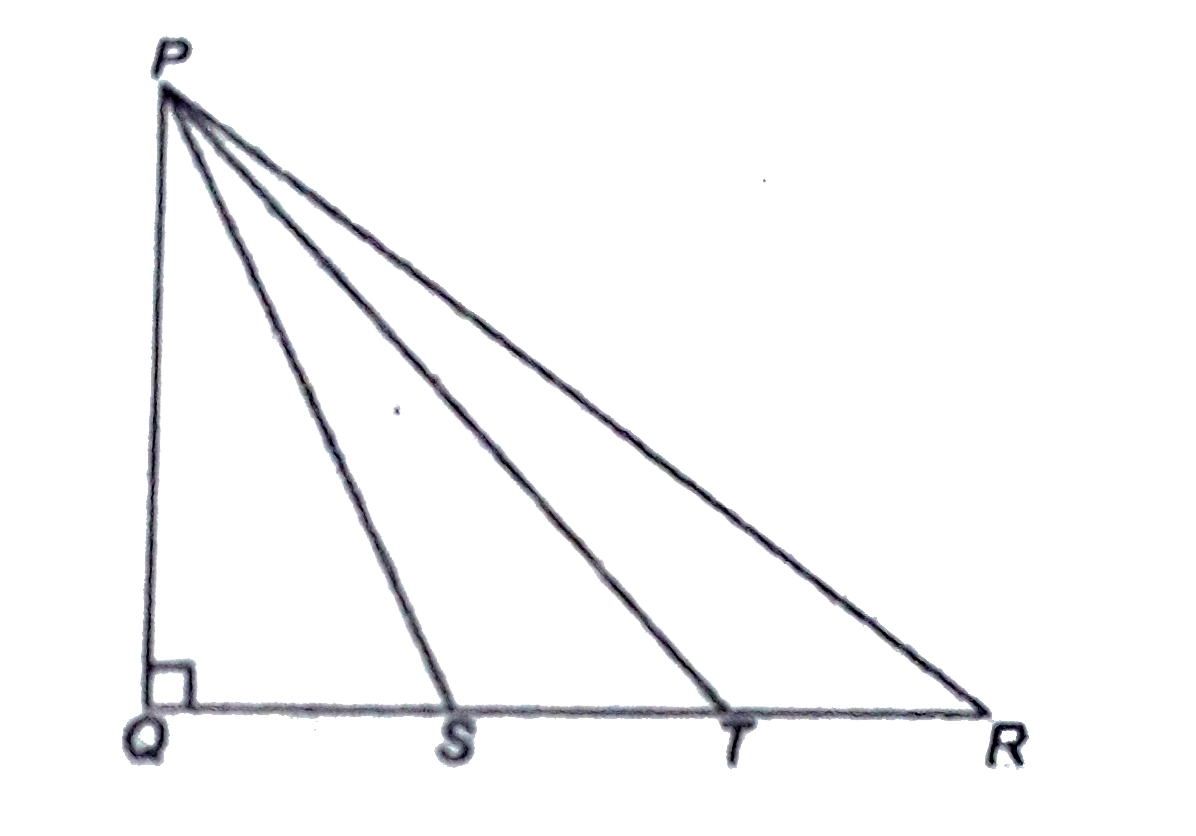
Let QS= ST = TR=x
Now by pythagoras theorem
$P R^{2} = P Q^{2} + Q R^{2} = P Q^{2} + {(3 x)}^{2}$
$3 P R^{2} = 3 P Q^{2} + 27 x^{2}$
Also , $P S^{2} = P Q^{2} + Q S^{2}$
(by pythagoras theorem)
$= P Q^{2} + x^{2}$
$\Rightarrow 5 P S^{2} = 5 P Q^{2} + 5 x^{2}$
Adding (1) and (2) ,we get
$R . H . S = 3 P R^{2} + 5 P S^{2} = 8 P Q^{2} + 32 x^{2} = 8 (P Q^{2} + 4 x^{2}) = 8 [P Q^{2} + {(2 x)}^{2}]$
$= 8 (P Q^{2} + Q T^{2}) = 8 P T^{2}$ ( by Pyhagores theorem)
L.H.S. Hence proved.

Updated on:21/07/2023

|

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

Similar Questions

NAGEEN PRAKASHAN ENGLISH-TRIANGLES -Revision Exercise Long Questions

Home
Ask Doubt
Profile