Question

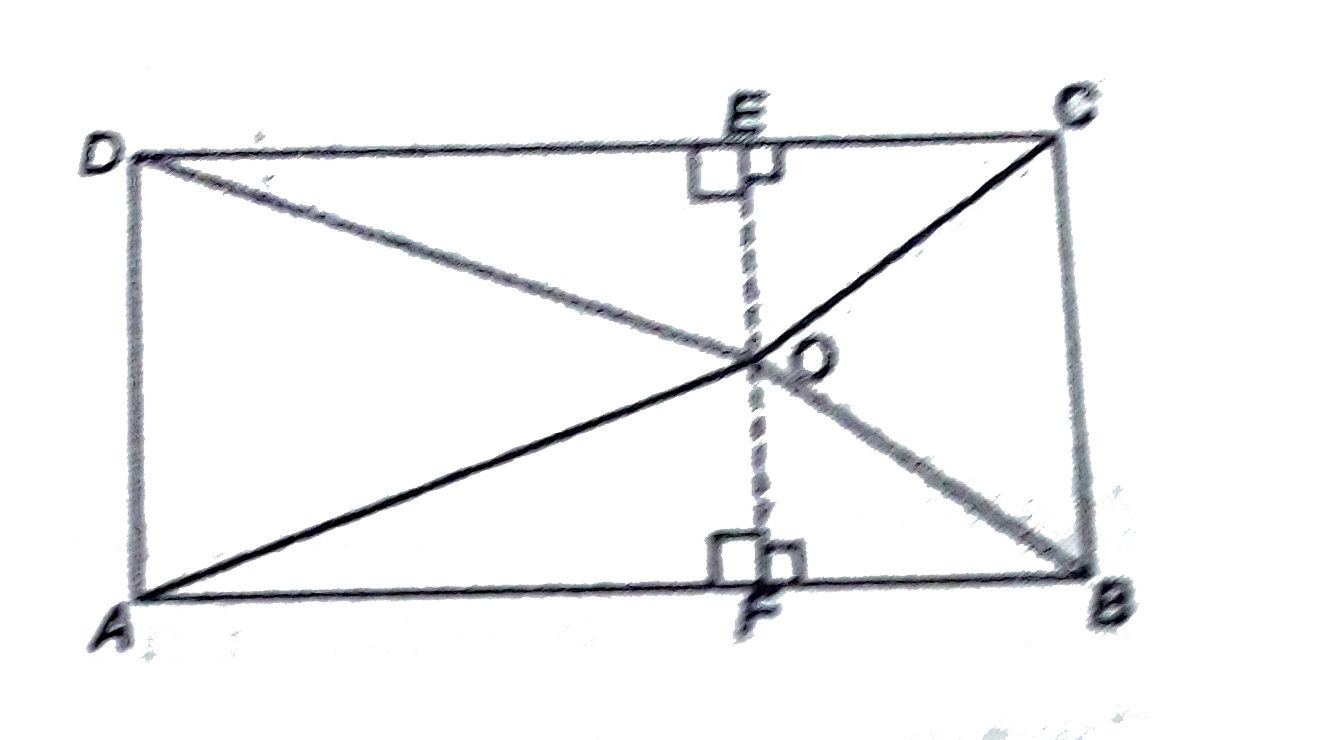
O is any point inside a rectangle ABCD. Prove that $O{B}^2+O{D}^2=O{A}^2+O{C}^2$.

Explanation

ABCD is a rectangle and O is a point interior the rectangle. Through O, draw a line parallel to BC meeting AB and DC at F and E respectively.
Then EC= FB and DE = AF
L.H.S = `OB^(2)+OD^(2)`
= `(OF^(2) + FB^(2)) + (OE^(2)+DE^(2))`
(by pythagoras theorem)
`OF^(2)+EC^(2)+OE^(2)+AF^(2)`
( EC = FB and DE = AF)
` (OE^(2)+EC^(2))+ (OF^(2)+AF^(2))`
` OC^(2) + OA^(2) `
= R.H.S.
Hence `OB^(2)+OD^(2) = OC^(2) + OA^(2)` Hence proved.