Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
Given : △ABC~△DEF AP and DQ are the mdians of △ABCand△DEF respectively.
To Prove: ar(△ABC)ar(△DEF)=AP2DQ2
Proof : AP and DQ are medians
Therefore, BP=PC and EQ=QF
and given △ABC~△DEF
Therefore, ABDE=ACDF=BCEFand∠A=∠D,∠B=∠Eand∠C=∠F
Now ABDE=BCEF
⇒ABDE=2BP2EQ⇒ABDE=BPEQ ...(1)
and ∠B=∠E
Hence △ABP~△DEQ (by SAS similarity)
Now in △ABCand△DEF
ar(△ABC)ar(△DEF)=AB2DE2
( the ratio of areas of two similar △'s is equal to ratio of squares of their corresponding sides)
form (1) and (2) , we have
ar(△ABC)ar(△DEF)=AP2DQ2 Hence proved
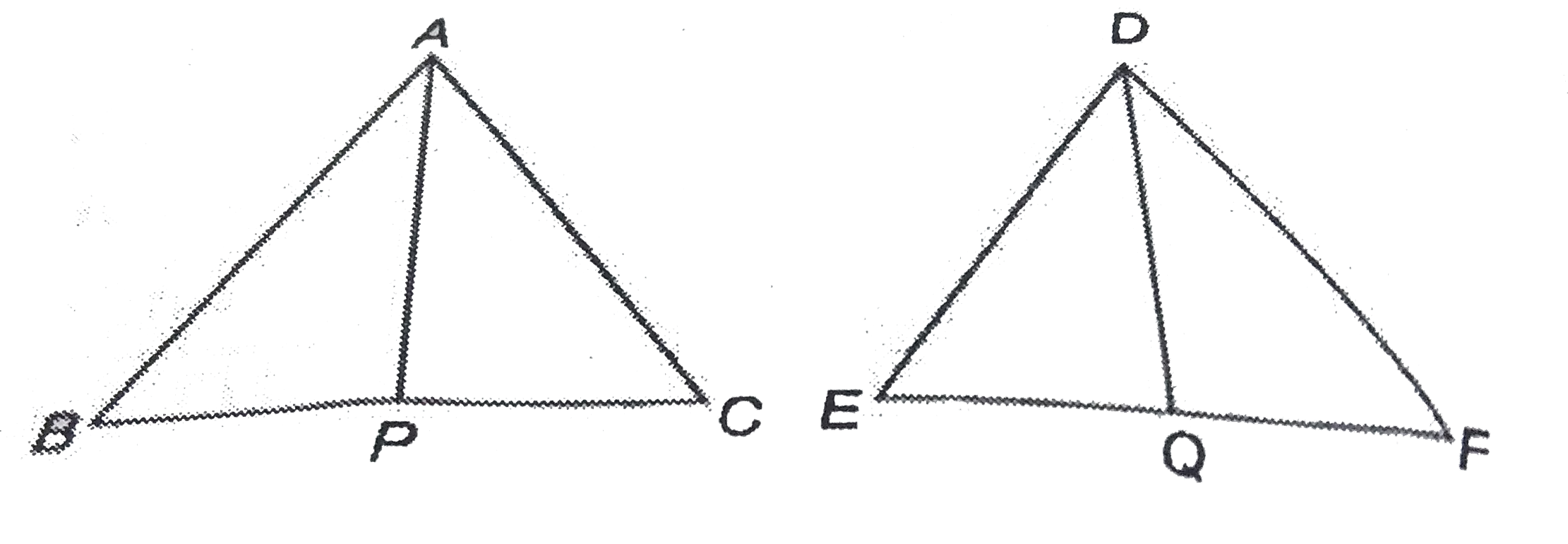
Topper's Solved these Questions
- TRIANGLES Book:NAGEEN PRAKASHAN ENGLISHChapter:TRIANGLES Exercise:Problems From NCERT/ Exemplar
Explore 14 Videos
- TRIANGLES Book:NAGEEN PRAKASHAN ENGLISHChapter:TRIANGLES Exercise:Exercise 6a
Explore 24 Videos
- STATISTICS Book:NAGEEN PRAKASHAN ENGLISHChapter:STATISTICS Exercise:Revision Exercise Long Answer Questions
Explore 4 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBook:NAGEEN PRAKASHAN ENGLISHChapter:VOLUME AND SURFACE AREA OF SOLIDSExercise:Revisions Exercise Long Answer Questions
Explore 5 Videos
Similar Questions
Theorem 6.6 : The ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides.
View SolutionThe ratio between the areas of two similar triangles is 16 : 25. Find the ratio between their :
corresponding medians.
View Solutionprove that The areas of the two similar triangles are in the ratio of the square of the corresponding medians.
View SolutionThe ratio between the altitudes of two similar triangles is 3 : 5, write the ratio between their :
corresponding medians.
View SolutionThe area of two similar traingles are 25 sq cm and 121 sq cm. Find the ratio of their corresponding sides
View SolutionThe ratio between the areas of two similar triangles is 16 : 25. Find the ratio between their :
corresponding altitudes.
View SolutionIf the areas of two similar triangles are equal, prove that they are congruent.
View SolutionIf the areas of two similar triangles are equal, prove that they are congruent.
View SolutionThe areas of two similar triangles are 9 cm2 and 16 cm2 respectively . The ratio of their corresponding sides is
View SolutionIf the altitude of two similar triangles are in the ratio 2:3 , what is the ratio of their areas?
View Solution