Question

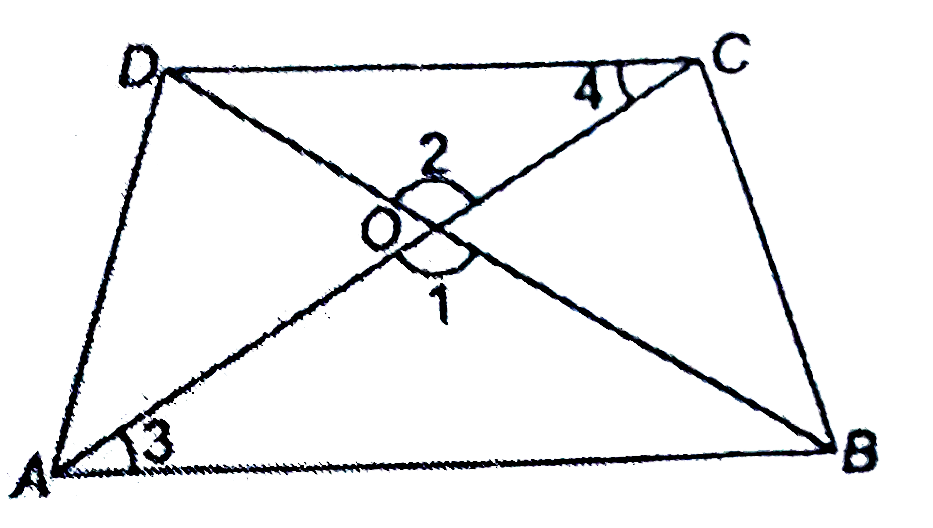
In Fig.
4.170, $ABCD$
is a
trapezium in which $AB\parallel DC$
and $AB=2DC$
. Determine
the ratio of the areas of $AOB$
and $COD$
.
(FIGURE)

Explanation

In `triangleAOB and triangleCOD`.
`angle1=angle2` ( vertically opposite angles)
`angle3=angle4` (alternate angles as AB||DC)
`triangleAOB~triangleCOD` (AA corollary)
`(ar(triangleAOB))/(ar(triangleCOD))=(AB^(2))/(CD^(2))`
( ratio of areas of two similar triangles is equal to the ratio of squares of their corresponding sides)
`((2CD)^(2))/(CD^(2))` ( AB= 2CD, given)
`(4CD^(2))/(CD^(2))=4/1`
`ar(triangleAOB): ar(triangleCOD) 4:1`